All planets and moons are inevitably subjected to that UNIVERSAL PHENOMENON
because (everything else equals),
(Tsat.planet.1) /(Tsat.planet.2) =
= [ (N1*cp1) /(N2*cp2) ] 1/16
The PLANETARY (Tsat /Te.correct) CRITERION
The Rotational Warming Factor (β*N*cp)1/16
The PLANETARY (Tsat /Te.correct) CRITERION
Table of data
................HORIZONTAL...............1 GRAPH........2 GRAPH
Planet..Warming factor... Φ.......(Tsat /Te)....(Tsat /Te.correct)
...............(β*N*cp)1/16 criterion
Mercury........0,895..........0,47........0,773..............0,934
Moon............0,998..........0,47........0,815..............0,982
Earth............1,368..........0,47........1,134..............1,365
Mars.............1,227..........0,47....... 1.....................1,207
Ceres............1,4535...........1............ - ................... - ......
Io..................1,169...........1............1,156..............1,156
Europa.......1,264.........0,47......1,072............1,294
Ganymede....1,209..........0,47........1,028..............1,242
Calisto..........1,147...........1............1,169..............1,169
Enceladus....1,341...........1.............1,340..............1,340
Tethys..........1,315...........1............1,292..............1,292
Titan..............1,1015.........1............1,1086............1,1086
Triton...........1,158..........? .............1,297. ?...........1,297 ?
Pluto............1,116...........1............1,189..............1,189
Charon.........1,218...........1............1,265..............1,265
***********************
I had the impression that I need to work on it.
A lot of things about it were really exciting, the biggest being that you'll potentially discover a new PHENOMENON from the PLANETS TEMPERATURES COMPARISON.
***********************
The Rotational Warming is a UNIVERSAL PHENOMENON, because what we have discovered is that
for all planets and moons the average surface temperatures, measured by satellites (Tsat)
RELATE,
(everything else equals),
as their respective (N*cp) product
in SIXTEENTH ROOT.
(Tsat.planet.1) /(Tsat.planet.2) =
= [ (N1*cp1) /(N2*cp2) ] 1/16
Where:
Tsat - Kelvin, is the planet's average surface temperature
N - rotations/day, is the planet's axial spin.
cp - cal/gr*oC, is the planet's average surface specific heat.
**********************
Here it is a simplified example to explain the above relation:
Let's consider two identical planets (1) and (2) at the same distance from the sun, and
Planet (1) rotates twice as fast as Planet (2).
(N1) = 2*(N2) everything else equals - then the
average surface
temperatures (T1) and (T2) relate as:
(T1) /(T2)= [ (N1*cp1) /(N2*cp2) ] 1/16
everything else equals, and, additionally, in the present simplified example,
the average surface specific heat (cp) for both planets (1) and (2) is the same, so
cp1 = cp2
and, the above equation re-writes as:
(T1) /(T2)= [ (N1) /(N2) ] 1/16
and because N1 = 2*N2, then we shall have:
(T1) /(T2) = ( 2 ) 1/16
or
(T1) /(T2) = 1,0443
If for Planet (2) the (T2) = 250K,
Then for Planet (1) the
(T1) = (T2) *1,0443
(T1) = 250K *1,0443 = 261K
(T1) = 261K
***************
And it happens so (the discovered relation), because when solar light hits the surface, the EM energy, what it does, is to INTERACT with MATTER.
****************
It is widely known, that Earth on average surface is warmer than Moon.
The above relation begs for a question:
So why it is warmer on Earth, compared to Moon ?
It is very interesting...
In science, “you only find what you are looking for, and you only look for what you know."
To be able to find the origin of this whole process, we had to think about the unthinkable. And we did it.
And we begun thinking about the unthinkable !!!
Because,
"There are tons of discoveries where scientists were looking for one thing, but found something else."
************************
The lunar surface is much hotter for many earthern days, but if you shade an area, the shaded area could be colder than any place on Earth.
Isn't it what people in Sahara desert do? They shade a place and hide underneath the shade for the hot solar lit hours of the day.
So with the lunar regolith, it’s surface [top cm] can be about
120 °C (at Lunar Equator), but a meter below the surface it would be about
-40 °C, so only top few [cm] gets hot. You may keep ice cream quite frozen [forever] less than 1 meter below the lunar surface.
Of course at the 120 °C the heated top surface, radiates into space a lot more than Earth's surface can, so the top meter of the lunar surface is much colder, than Earth's surface can be, and on global average it is a lot colder.
–
Exactly! On Earth we have the Sahara desert’s similar to the lunar regolith phenomenon. Sahara deserts’ surface [top cm] can be about 80 °C, but a meter below it is very comfortable for lizards, snakes and insects to hide – it is a comfortaly cool environment there.
So it is hotter on the Moon’s surface (compared to Earth’s Sahara) during the long lunar day, but it is very much colder a meter below. It is -40 °C a meter below the lunar surface during the long lunar day.
This observed phenomenon happens due to the very slow rotation with respect to the sun Moon has.
Earth, on the other hand, rotates 29,5 TIMES FASTER.
On Earth there is much more effective the solar energy INPUT in the surface – thus Earth’s surface is on average much warmer than Moon’s.
It is the Planet Surface Rotational Warming Phenomenon.
–
–
We have a simple example in our everyday's practice – it is the chicken rotisserie. The faster the spit rotates the better the chicken is cooked inside.
When the spit is slow, the chicken is burned outside and it is cold and raw inside.
It is what we see happening to our Moon’s surface.
It is 120 °C [top cm] and it is -40 °C a meter below.
-
-
Also, we know, that there are vast areas on Earth covered with permafrost.
If the atmospheric greenhouse warming effect was real, permafrost shouldn't have been there.
"Around 15% of the Northern Hemisphere or 11% of the global surface is underlain by permafrost,[5] covering a total area of around 18 million km2 (6.9 million sq mi).[6] This includes large areas of Alaska, Canada, Greenland, and Siberia. "
https://en.wikipedia.org/wiki/Permafrost
The rising temperatures melt some of the permafrost coverage, allright.
But the very existence of permafrost testifies against the atmospheric greenhouse warming effect.
-
-
And, a 1,5 °C rise of the global air temperature of course doesn’t thaw glaciers.
True, true and there is nothing subtle about the fact that ice on the North Pole is mostly floating seawater, whereas at the South Pole it’s snow falling on a landmass.
-
-
To maintain permafrost the annual average temperature must be -2 °C or lower.
Please explain the significance of this value in the context of the greenhouse effect.
What you provide is the annual average "air" temperature. It is a mixed measurement.
In the mixed measurement the temperature is influenced from the screen’s the own temperatures IR emissions, the air temperature and the soil's (beneath the screen) temperature.
And this is only a simplified description of the Standardised STEVENSON screens "measurements" of "air" temperature.
But let's admit it measures a kind of complex temperature at the spot.
Which, of course, is not the spot's skin surface temperature.
Also, of course, at the places where the permafrost is maintained, the "air" temperature would be low enough.
But it is not the "air" temperature that makes the soil to freeze.
*********************
Let's proceed with it systematically. Let's do it scientifically.
Let's begin with the most helpful comparison - Let's begin with Earth's and Moon's average surface temperatures comparison.
Earth's average surface temperature is much higher than Moon's average surface temperature.
Planets and moons are celestial bodies which rotate under the sun. All planets and moons are inevitably subjected to the same Physical Laws.
What we consider happening to the Earth's and to the Moon's temperatures should be necessarily confirmed by the rest planets' and moons' temperatures same behavior.
Earth's average surface temperature is much higher than Moon's average surface temperature.
Why?
What it is the so dramatically different between those two celestial bodies, (Earth and Moon), to have resulted to the so much great average surface temperatures differences?
It was asserted that Earth has an atmosphere, and Moon doesn't have. It was said Earth's atmosphere acts as a blanket, which keeps Earth's surface warm.
What we have discovered, is that the warming-blanket-atmosphere theory is all mistaken. Earth's atmosphere is very thin, and therefore Earth's atmosphere is not capable to warm the surface to any significant extend.
Earth's atmosphere doesn't have any considerable greenhouse warming effect on the surface.
So what else we have?
We have discovered that Earth on average is much warmer than Moon, because Earth rotates very much faster than Moon.
Actually Earth rotates 29,5 times faster than our Moon.
Also Earth's surface is covered with water, and the continents' surface is mostly extremely wet.
On the other hand, Moon's surface is covered with dry lunar regolith.
Actually water has five times (5) higher the specific heat capasity than the dry lunar regolith.
It is 1 cal/gr*oC for water, vs 0,19 cal/gr*oC for dry lunar regolith.
The very POWERFUL the Solar Irradiated planet surface Rotational Warming Phenomenon ( N*cp )1/16
********************
The method we use is the "Planets and moons surface temperatures comparison".
We are comparing the various different planets and moons (without-atmosphere, or with a thin atmosphere, Earth included).
*****************
Earth and Moon are at the same distance from the sun, and, therefore, Earth and Moon are solar irradiated with the same intensity flux, because at Earth's and Moon's distance from the sun,
the So = 1.361 W/m² (So - it is the Solar constant - the solar flux at the Earth's average distance from the sun).
We shall first compare the Earth's and Moon's the average surface temperatures
(for equal average Albedo a=0,306
the mean surface temperatures
Tmean.earth = 288K
vs Tmean.moon = 206,7K)
because Earth and Moon share the same intensity solar flux So = 1.361 W/m² , and therefore it makes the comparison most simple.
For avr. surface Moon T =220K
{ [(220K)⁴ /(1-0,11)]*(1-0,306) }¹∕ ⁴ = 206,7 K
So we shall have:
Tmean.earth /Tmean.moon =
= 288K /206.7K = 1.3933
and the comparison for Earth and Moon,
their (N*cp) products' sixteenth root:
[ Earth(N*cp) /Moon(N*cp) ] 1/16=
= [ (1*1) /(0,0339*0,19)] 1/16 =
= (155,42)1/16= 1,3709
where
N.earth = 1 rot/day
N.moon = 0,0339 rot/day
Earth’s cp = 1 cal/gr*oC (oceanic waters, and land mostly wet)
Moon's cp = 0,19 cal/gr*oC (dry lunar regolith)
...........................
When we compare the results (1,3933) and (1,3709) we recognize that they are almost identical!
It is a demonstration of the Planet Surface Rotational Warming Phenomenon:
Planets' and moons' mean surface temperatures relate (everything else equals) as their (N*cp) products' sixteenth root.
(Tmean.planet.1) /(Tmean.planet.2) =
= [ (N1*cp1) /(N2*cp2) ] 1/16
.............................
.............................
............................................
More Planets and Moons the satellite measured average surface temperatures comparison
Links:
Jupiter/Saturn/Neptune 165K /134K /72K
The Rotational Warming Phenomenon is right, because it has been many times demonstrated and, also, it has been theoretically explained by the physics first principles.
There are more comparisons and there are more Links further on.
******************
What is very important to accent to is that, at every given moment, lunar surface temperatures are very much differenciated.
Earth's surface temperatures are also very much differenciated.
When comparing, though, the lunar and Earth's surfaces' temperature behaviors, we can clearly see that lunar surface temperatures are way much more differenciated than Earth's surface temperatures are.
Because, as we have seen above, Earth's and Moon's the respective surface properties, both (N) and (cp), which are mutually compared by their respective (N*cp) products - they are very much different:
Earth(N*cp) /Moon(N*cp) = 155,42
Because Earth's surface is 155,42 TIMES more prepared, when compared to lunar surface's properties for the solar energy INPUT capasity.
***************
There is the known physical concept:
For two identical celestial bodies IR emitting the same amount of EM radiation,
or, in general case, for two celestial bodies IR emitting, on the average surface, the same amount of EM radiation,
the body with the less differenciated surfaces' temperatures has the higher average surface temperature.
Very well. Let's see.
We have them precisely measured by satellites, but do we have them calculated theoretically?
To theoretically estimate the planet average surface temperature there is for more that forty (40) years the use of the planet theoretical effective temperature (Te).
The planet theoretical effective temperature (Te) is a mathematical abstraction. It theoretically calculates for the planet some uniform surface temperature (Te) which is an abstract approximation of the measured planetary average surface temperature (Tsat).
Nevertheless, the (Te) brings in a brilliant insight, it assumes the possibility of determining the planet's or moon's the average surface temperature by simply measuring the incident on the surface radiative energy flux.
The idea was, that when a celestial body is considered as a blackbody, and the incident on the surface radiative energy flux, which would be evenly averaged over the entire selestial body's surface,
and when the evenly averaged energy induces the uniform surface temperature (Te), according to the Stefan-Boltzmann emission law, eventually that energy is evenly emitted, when eventually that energy is evenly emitted, and when using the S-B law backwards, then we could have calculated the planet's or moon's a uniform surface temperature, the (Te), which is an approximate temperature, and which is named the planet effective temperature (Te).
It is an approximate surface temperature, because of the simple reason - planets and moons do not have a uniform surface temperatures.
The fact is, the planets' and moons' the calculated (Te) are not matching the data, the NASA satellite measured temperature (Tsat).
Moreover, the measured (Tsat) are almost always higher than the calculated (Te).
Nevertheless, when based on the philosophical principle, which states about the ORDERLY UNIVERSE, we distiquished there should be a deterministic relationship between planet average temperature (Tsat) and the planet theoretical effective temperature (Te).
In present research we compared for all planets and moons their respective average surface temperatures, measured by satellites, the (Tsat), with their respective the theoretical effective temperatures (Te), and with their respective the corrected effective temperatures, the (Te.correct).
There was an obvious need to correct the (Te) values, because for the smooth surface planets and moons, their surfaces' the strong specular reflection was neglected in the planetary radiative energy balance estimation.
Thus the PLANETARY (Tsat /Te.correct) CRITERION started to getting shape.
Table of data
................HORIZONTAL...............1 GRAPH........2 GRAPH
Planet..Warming factor... Φ.......(Tsat /Te)....(Tsat /Te.correct)
...............(β*N*cp)1/16 criterion
Mercury........0,895..........0,47........0,773..............0,934
Moon............0,998..........0,47........0,815..............0,982
Earth............1,368..........0,47........1,134..............1,365
Mars.............1,227..........0,47....... 1.....................1,207
Ceres............1,4535...........1............ - ................... - ......
Io..................1,169...........1............1,156..............1,156
Europa.......1,264.........0,47......1,072............1,294
Ganymede....1,209..........0,47........1,028..............1,242
Calisto..........1,147...........1............1,169..............1,169
Enceladus....1,341...........1.............1,340..............1,340
Tethys..........1,315...........1............1,292..............1,292
Titan..............1,1015.........1............1,1086............1,1086
Triton...........1,158..........? .............1,297. ?...........1,297 ?
Pluto............1,116...........1............1,189..............1,189
Charon.........1,218...........1............1,265..............1,265
It was considered the effective temperature Te of an airless celestial body ought to be higher than its respective measured average surface temperature Tsat, because Te is a uniform surface temperature.
Two identical bodies emitting the same amount of IR EM energy, the body with the less differenciated surface temperatures, has the higher average surface temperature.
Thus, for airless planets and moons, the theoretically calculated Te was considered they should be higher than their respective measured Tsat, because for planets and moons their surface temperatures are very much not uniform.
The concept is correct for the bodies which are previously warmed, or having some inner sources of thermal energy. And then the bodies spontaneously transform the already existing thermal energy into IR EM outgoing energy.
Planets and moons do emit IR EM energy, but that energy comes as a result of solar incident flux's interaction process with the planetary surface matter.
When the incident radiative energy interacts with matter it is not a simple the thermal energy absorption, as it was previously believed, because when interacting with matter, there are more complex transformations of energy occur.
(we explain about it further on below)
So, the theoretically calculated Te temperatures, as it was previously believed, should have been higher than their respective measured Tsat,
but NO, actually they (Te) are NOT higher than (Tsat), actually they are lower than (Tsat) – and this assertion is based on the actual observations -it is based on the planets' measured average surface temperatures Tsat, which confirm that the planets' and moons' the theoretically calculated temperatures Te are mostly not higher, but are lower than their respective measured Tsat -
- see table of data above.
–
Because planets and moons rotate, and the faster a planet or moon rotates – the higher is the solar energy INPUT – the higher the average surface temperature Tsat.
It is the Solar Irradiated Planet Surface Rotational Warming PHENOMENON.
************
Yes, planets and moons do obey to the same universal laws, it is an axiom, but every planet and moon has the very much distinquished the input parameters.
Also, it is important to notice, the PLANETARY
(Tsat /Te.correct) CRITERION is not the same for every planet and moon. The PLANETARY
(Tsat /Te.correct) CRITERION is not a constant.
Thus, every planet and moon has its own unique value for the PLANETARY (Tsat /Te.correct) CRITERION.
And, of course, there should be a good reason for that.
And it happens so, and the good reason is, because every celestial body is inevitably subjected to the Planet Surface Rotational Warming Phenomenon.
****************
Also, we have corrected their respective Blackbody Effective Temperatures (equilibrium temperatures) (Te),
because we concluded that planets and moons with smooth surface, along with their surface's diffuse reflection, they also have a STRONG SPECULAR REFLECTION.
*******************
Mercury in color.
Surface consists from basalt crust.
Mercury is a smooth surface planet, so the coefficient 'Φ' for Mercury is:
Φ = 0,47
Io: Galileo spacecraft true-color image of Io.
Surface consists from sulfur and sulfur oxides.
There are over 450 active cryo-volcanos.
Io is a heavy cratered (rough) surface moon, so the coefficient 'Φ' for Io is:
Φ = 1
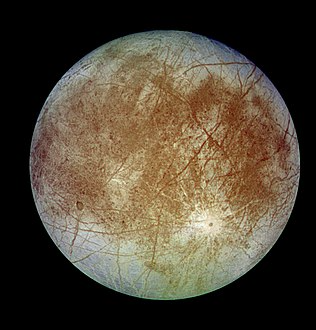
Europa: Imaged on 7 September 1996 by Galileo spacecraft.
Ice-crust surface.
Europa is considered the smoothest object in Solar System.
Europa is a smooth surface moon, so the coefficient 'Φ' for Europa is:
Φ = 0,47
Callisto: Imaged in 2001 by NASA's Galileo spacecraft.
Ice-crust surface.
Callisto is considered the most cratered object in Solar System.
Callisto is a heavy cratered (rough) surface moon, so the coefficient 'Φ' for Callisto is:
Φ = 1
Enceladus: View of trailing hemisphere in natural color
Surface consists from icy-snow-crust.
Enceladus is a rough surface moon, so the coefficient 'Φ' for Enceladus is:
Φ = 1
Ganymede by NOAA.
Ganymede is a smooth surface moon, so the coefficient 'Φ' for Ganymede is:
Φ = 0,47
In the Graph the cause and effect move in the same direction.
The Graph Ratio of Measured Planet Temperature to Corrected Blackbody Temperature (Tsat /Te.correct), as a Quasi-LINEAR function of the Rotational Warming Factor = (β*N*cp)1/16
The PLANETARY (Tsat /Te.correct)
CRITERION
In the (Tsat/Te.correct) ratio Graph the planets and moons with smooth surface (the red dots), and the heavy cratered surface (rough) respectively (the green dots), are streched along the SINGLE LINE, because there is a deterministic relationship with the Rotational Warming factor.
The Rotational Warming Factor (β*N*cp)1/16
************
What we did in the present research was to compare the different planets and moons surface temperatures in accordance with the incident on the planet surface their respective solar flux (S), with their respective average surface Albedo (a), and, also, in accordance with their respective rate of rotation (N) , and with their respective surface specific heat (cp) and surface roughness features (the light capturing abilities).
-
In this research we have shown, and now we demonstrate that a planet average surface temperature (Tmean K) is determined by those five (5) major parameters:
1. The distance from the sun (solar flux "S" W/m²).
The intensity of the incident on a celestial body solar EM energy is dependent on the distance from the sun the square inverse law value.
Because the intensity of solar EM energy upon a planet or moon is the major factor determiming the planet's or moon's average surface temperature Tmean.
2. The average surface diffuse reflectivity (Albedo "a"),
which is dependent on the planet's or moon's average surface the diffuse reflection properties.
Because the higher the planet's or the moon's Albedo - the less solar EM energy interacts with surface's matter, the less is the average surface temperature Tmean.
3. The surface's spherical shape and the surface's roughness coefficient (the solar irradiation accepting factor "Φ").
Because planets and moons have very distinguished their respective surfaces features - there are the smooth surface planets and moons and there are the rough surface planets and moons.
The rough surface planets and moons almost do not exhibit the specular reflection from their respective surfaces.
4. The rate of rotation ("N" rotations/day),
which is a unique for every planet and moon value.
Because when rotating faster a planet's or moon's surface accumulates more solar energy, and, therefore, the planet or moon average surface temperature Tmean appears to be higher.
5. The average surface specific heat ("cp" cal/gr*oC),
which is determined by the planet the respective average surface chemical composition.
Because when the average surface specific heat cp is higher, there are more atoms streched over the square meter of surface, there are more atoms to interact with the incident solar EM energy.
Therefore the average surface temperature Tmean appears to be higher.
Yes, the same universal laws, but applied to different input parameters.
So the same universal physical laws, but applied to different celestial bodies.
And, there also is, the very POWERFUL the Solar Irradiated planet surface Rotational Warming Phenomenon.
******************************************************
Summary:
A new universal equation for calculating a planet's mean surface temperature is developed here, to provide better estimates than the simple "blackbody" equation which was based on simplifying assumptions.
Recognizing that a real planet does not match the assumptions for an idealized blackbody, Vournas developed an expanded equation with four additional terms to better represent a planet's actual conditions, particularly considering planet axial rotation.
The derivation of the new equation from the planet energy balance is shown below, followed by a description for each of the four new terms in the new equation, including rotation (N), specific heat capacity (cp), solar light reflection and dispersion (Φ, a), and a new universal constant (β) determined empirically.
This new Vournas equation results are compared for twenty (20) solar system bodies (planets and moons), with the equation's calculated temperature closely matching the data, the NASA satellite measured temperature.
********************************************************
Earth is warmer than Moon, because Earth rotates faster.
The Planet Without-Atmosphere (Tmean) Universal Equation
What we need is a universal equation, when substituting in the equation for every planet's or moon's surface its respective the major characteristic data, because we should be able to theoretically calculate for every planet or moon the average surface temperature (Tmean).
The Planet Surface Rotational Warming Phenomenon should be necessarily present in the Planet Without-Atmosphere (Tmean) Universal Equation, because to this Phenomenon all planets and moons are strongly subjected.
******************
Very well...
***********
The (Tsat /Te) Ratio's Deterministic Relationship
-
Tsat - is the satellite measured the planet or moon average surface temperature.
Te - is the theoretically calculated the planet or moon uniform surface temperature (the effective temperature).
Te = [(1-a) S /4σ ]1/4 (K) (1)
where
a - is the average surface Albedo.
Albedo is defined as the diffuse reflected portion of the incident on planet surface solar flux.
S - W/m² the solar flux at the Planet's or moon's average distance from the sun.
σ = 5,67*10⁻⁸ W/m²K⁴, the Stefan-Boltzmann constant
*************
Whereas, because of its natural occurence, the (Tsat) is all the solar and planetary parameters dependent value, on the other, the theoretically calculated planet effective temperature (Te) is only the Solar flux (S) and the planetary Albedo (a) dependent value.
-
What we see is that for different planets and moons (Tsat) and (Te) do not give the same respective numerical values, hence (Tsat) and (Te) are not physically comparable,
also when we compare for the different planets and moons their respective (Tsat/Te) ratios, the ratios are very different,
and that implies the effect of some additional planetary physical variables...
therefore, there definitely should be some deterministic relationship between the (Tsat) and (Te) values.
Those two terms,
the measured average surface temperature (Tsat) simply occurs as the result of solar EM energy actual interaction with planet surface
and
the calculated uniform surface temperature (Te) is a result of theoretical approach
both those two terms (the average Tsat and the uniform Te) deal with the entire planetary surface temperature (they both resulting to the average surface temperature), but they are different the average surface temperatures physical terms.
Therefore, they cannot be (the measured Tsat average vs the calculated Te uniform), they cannot be straight-forwardly compared between them...
We note that (Tsat) and (Te) both depend on the same physical properties,
i.e. both (the measured Tsat average) and (the calculated Te uniform) strongly depend on the same two physical properties, namely:
S - the same solar flux (W/m²)
a - the same average Albedo
As we demonstrate further on, Tsat > Te almost for all planets and moons.
So there should be a good reason for that
Tsat > Te
The measured (Tsat) also necessarily depends on planet spin (N) and on planet surface specific heat (cp).
But for the theoretically calculated (Te) - according to the blackbody planet uniform surface temperature definition - the planet spin (N) and the planet surface specific heat (cp) are being ignored in the theoretical Te formula.
Thus, some necessarily expected influence from the planet spin (N), on the planet average surface temperature is whatsoever absent in the theoretical (Te) temperature.
And, some necessarily expected influence from the planet average surface specific heat (cp), on the planet average surface temperature is also whatsoever absent in the theoretical (Te) temperature.
When we compare for the different planets and moons their respective (Tsat/Te) ratio, we are confident we are on the right direction,
because there should be a deterministic relationship on the basis of the newly discovered the Rotational Warming Phenomenon.
Thus, we proceed then, for planets and moons, relating their respective
(Tsat/Te) ratio with their respective Rotational Warming Factor = (β*N*cp)1/16
where
β = 150 days*gr*oC/rotation*cal – ( the Rotational Warming Factor constant ),
which relates the rotating planet average surface temperature (Tsat) with the planet effective temperature (Te), and this new constant (β) is determined empirically.
Below we can see:
The Graph Ratio of Measured Planet Temperature to NOT CORRECTED Blackbody Temperature (Tsat /Te), as a linear function of the Rotational Warming Factor = (β*N*cp)1/16
Tsat /Te
(not corrected)
The Rotational Warming
Factor (β*N*cp)1/16
In the not corrected (Tsat/Te) ratio Graph the planets and moons with smooth surface (the red dots), and the heavy cratered surface (rough) respectively (the green dots), INEVITABLY are streched along TWO LINES, because for the planets and moons with SMOOTH SURFACE the SPECULAR REFLECTION has been NEGLECTED.
In the above graph we can observe those three major scientific truths:
1). (Tsat/Te) ratio, except for the very slow rotating Mercury and Moon,
the (Tsat/Te) ratio is (Tsat/Te) >1.
So there is Tsat > Te almost for all cases. And it is a very important observation.
2). We can see the obvious relation - the higher the Warming factor (β*N*cp)1/16, the higher is the (Tsat/Te) ratio.
3). The six smooth surface planets and moons - Mercury, Moon, Mars, Ganymede, Europa, Earth (the red dots) are streched in the lower line, under the heavy cratered (rough surface) planets and moons - (the green dots), which are streched in the upper line.
**************
The smooth surface planets' and moons' strong specular reflection was neglected in planet or moon radiative energy balance equation:
Energy in = Energy out
Thus in the equation, for the smooth surface planets and moons, the "Energy in" part was very much overestimated.
And that is why a New Term - the Solar Irradiation Accepting Factor "Φ" , (which is the planet surface spherical shape and the planet surface roughness coefficient) had to be inserted.
*****************
"The Bond albedo (also called spheric albedo, planetary albedo, and bolometric albedo), named after the American astronomer George Phillips Bond(1825–1865), who originally proposed it, is the fraction of power in the total electromagnetic radiation incident on an astronomical body that is scattered back out into space.
Because the Bond albedo accounts for all of the light scattered from a body at all wavelengths and all phase angles, it is a necessary quantity for determining how much energy a body absorbs. This, in turn, is crucial for determining the equilibrium temperature of a body.
Because bodies in the outer Solar System are always observed at very low phase angles from the Earth, the only reliable data for measuring their Bond albedo comes from spacecraft."
(Emphasis added)
-
-
"Albedo (/ælˈbiːdoʊ/al-BEE-doh; from Latin albedo 'whiteness') is the fraction of sunlight that is diffusely reflected by a body. It is measured on a scale from 0 (corresponding to a black body that absorbs all incident radiation) to 1 (corresponding to a body that reflects all incident radiation)."
(Emphasis added)
-
-
"is the fraction of power in the total electromagnetic radiation incident on an astronomical body that is scattered back out into space."
-
"is the fraction of sunlight that is diffusely reflected by a body."
What we noticed is that the Planetary Bond Albedo is defined as the total scattered back out into space.
Also we have noticed that when satellites measure the Planetary Bond Albedo, satellites cannot measure the planet surface specular reflection, because the planet specular reflection doesn't hit the meauring sensors.
Also the planet surface specular reflection is mistakenly considered as very insignificant,
therefore when the Planetary Bond Albedo is measured by satellites,
what satellites measure is only the fraction of sunlight that is diffusely reflected by a body.
Nevertheless, those measurements were considered as the Planetary Bond Albedo, which they are not.
Therefore, for the smooth surface planets and moons, their STRONG SPECULAR REFLECTION was neglected.
In conclusion, the satellite measured Planetary Bond Albedo should be considered the fraction of sunlight that only is diffusely reflected by a body.
But when neglecting their STRONG SPECULAR REFLECTION, then it results to a very huge overestimation for the smooth surface planets and moons their equilibrium temperature (Te).
There is the need for a very precise estimation of the TOTAL reflected fraction (DIFFUSE and SPECULAR) of incident on planet or moon solar energy, which is the necessary quantity for determining how much energy a body absorbs.
This, in turn, is crucial for determining the equilibrium temperature of a body.
Thus it was very much necessary we had corrected their respective Blackbody Effective Temperatures (equilibrium temperatures) (Te),
So we had inserted the Solar Irradiation Accepting Factor Φ, which is the planet spherical shape and the planet surface roughness coefficient.
We have explained all aspects about the Solar Irradiation Accepting Factor Φ further on in the site.
*******************
Correcting the Effective temperature (Te) formula:
Te = [(1-a) S /4σ ]1/4 (K) (1)
It is well known and it is long established that there is the planets and moons surface's the incident SW solar light the diffuse reflection. And that SW solar light the diffuse reflection is what actually being the satellite measured reflection.
What is New is that planets and moons with smooth surface, along with their surface's diffuse reflection, they also have a STRONG SPECULAR REFLECTION.
The planet effective temperature (Te) is not only a theoretically mistaken approach to planet surface temperature calculation.
It is also, by ignoring the smooth surface planets and moons very strong specular reflection, the (Te) is also being mistakenly calculated.
Thus, (Te) is all wrong then. We should, at least, calculate (Te) correctly.
The smooth surface planets and moons specular reflection should be necessarily considered in the planets' and moons' "Energy in" estimation, because otherwise the Planet Energy Income will be very much overestimated.
**************
We insert the
Φ - the solar irradiation accepting factor (the planet spherical shape and planet surface roughness coefficient).
Φ =0,47 for smooth surface planets and moons.
Φ =1 for heavy cratered (rough surface) planets and moons.
The Φ is a coefficient - it is not a some kind of constant.
Φ may have all the values from the Φ =0,47 to the Φ =1.
When planetary surface is smooth enough, the coefficient Φ =0,47
Because no matter how much smoother the surface may be, the light will not be reflected more strongly.
And, when planetary surface is rough enough (heavy cratered enough), the coefficient
Φ =1.
Because no matter how much rougher the surface may be, the light will not be captured more strongly.
Also, there are values of Φ in between the 0,47 and 1.
In solar system only Triton appears to have its surface's coefficient Φ somewhere in between of 0,47 and 1.
Because a planetary surface is either smooth enough (Φ =0,47),
or it is rough enough (heavy cratered enough), (Φ =1).
**************
So,we insert the
Φ - the solar irradiation accepting factor (the planet spherical shape and planet surface roughness coefficient).
the origin of the coefficient "Φ" and its use is thoroughly explained further on.
Φ =0,47 for smooth surface planets and moons
Φ =1 for heavy cratered (rough surface) planets and moons
Te.correct = [Φ(1-a) S /4σ ]1/4 (K) (2)
Te.correct, for the smooth surface planets and moons, has a much lower, than Te, numerical values.
And, in the below "Corrected Graph", where all planets and moons are streched in one LINE, we see an obvious Quasi-LINEAR Relationship,
between the (Tsat/Te.correct) ratio, and the Rotational Warming Phenomenon -
(the Warming factor (β*N*cp)1/16 ).
Tsat = ~ Te.correct*(β*N*cp)1/16
-
Sunset on Earth
Earth is a smooth surface planet, so the coefficient 'Φ' for Earth is:
Φ = 0,47
The Graph Ratio of Measured Planet Temperature to CORRECTED Blackbody Temperature (Tsat /Te.correct), as a Quasi-LINEAR function of the Rotational Warming Factor = (β*N*cp)1/16
The PLANETARY (Tsat /Te.correct)
CRITERION
The Rotational Warming
Factor (β*N*cp)1/16
In the (Tsat/Te.correct) ratio Graph the planets and moons with smooth surface (the red dots), and the heavy cratered surface (rough) respectively (the green dots), are streched along the SINGLE LINE, because there is a deterministic relationship with the Rotational Warming factor.
In the above Graph (Tsat /Te.correct) we distinquish the following scientifical truths:
1). Tsat is planets' or moons' the average surface satellite measured temperatures.
2). N is the planets' or moons' the measured rotational ratio.
3). cp is the planets' or moons' (for the known average surface chemical composition) the specific heat.
4). Earlier we have demonstrated the Powerful Rotational Warming Phenomenon, which is present in the Graph.
5). When the planets' or moons' effective temperature (Te) is calculated, it is based on the measured solar flux upon the planet and it is based on the measured average surface Albedo.
6). When the effective temperature (Te) was corrected, the six planets and moons, which are known for their smooth surface features, were aligned with the rough surface planets and moons.
Consequently, all data used in above Graph are measured values.
Also, all data used in above Graph are independently measured values.
The six smooth surface planets and moons alignment with the rough surface planets and moons testifies for the rightness of the
Solar Irradiation Accepting Factor
(Φ = 0,47) - the planet surface spherical shape and roughness coefficient.
Thus the Solar Irradiation Accepting Factor
(for smooth surface Φ = 0,47) - the planet surface spherical shape and roughness coefficient - is of the major importance for the planets' or moons' the Radiative Energy balance
Energy in = Energy out
the very much precise estimation.
*****************
***************************
The Theoretical Equation
The theoretical equation we seek for, the theoretical equation Tmean should have its calculated results to be very close to the satellite measured temperatures Tsat, because it is exactly what we are looking for, so we shall have:
Tmean = Tsat = ~ Te.correct*(β*N*cp)1/16
Tmean = Te.correct * (β*N*cp)1/16
Tmean = [ Φ (1-a) S /4σ ]1/4 * (β*N*cp)1/16
or
Tmean = [ Φ (1-a) S (β*N*cp)1/4 /4σ ]1/4 (K) (3)
and
Tmean = [ Φ (1-a) S (β*N*cp)1/4 /4σ ]1/4 (K) (3)
************
So, a planet surface responses to the (interacts with), responses to the incident solar energy, and because of that there is an average surface temperature.
The Planet Surface Rotational Warming Phenomenon what it does is to modulate (to rise) the planet average surface temperature. Because the higher the planet's (N*cp) product - the higher the planet average surface (Tmean) temperature.
–
The EM energy comes from sun. There is the
“energy in =energy out” the equilibrium energy balance axiom to be met.
So, there is the Solar flux (S), surface Albedo (a), the solar irradiation accepting factor (the spherical shape and roughness coefficient (Φ) ), and also, there is the Planet Surface Rotational Warming Phenomenon.
Therefore, we have formulated the Planet Mean Surface Temperature Theoretical Equation:
Tmean = [ Φ (1-a) S (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴ (K) (3)
Equation is building in the actual Rotational Warming Phenomenon.
The Phenomenon correlates spin (N) and average surface specific heat (cp) with average surface temperature (Tmean).
**************
************
**********
We shall proceed by calculating the planets' and moons' Mean Surface Temperatures by the use of the Planet Mean Surface Temperature Theoretical Equation:
1. Earth's Without-Atmosphere Mean Surface Temperature Calculation.
R = 1 AU, is the Earth's distance from the sun in astronomical units (R = 150.000.000 km, which is Earth's average distance from the sun).
Earth’s albedo: aearth= 0,306
Albedo is defined as the diffuse reflected portion of the incident on planet surface solar flux.
Earth is a smooth rocky planet, Earth’s surface solar irradiation accepting factor is:
Φearth= 0,47
Φ - is the planet surface solar irradiation accepting factor (the planet surface spherical shape and the planet surface roughness coefficient).
Φ(1 - a) - is the planet surface coupled term (it represents the NOT REFLECTED portion of the incident on planet surface solar flux, it is the portion of solar flux which gets in INTERACTION processes with the planet surface).
β = 150 days*gr*oC/rotation*cal – ( the Rotational Warming Factor constant ).
N = 1 rotation /per day, is Earth’s rate of rotation in reference to the sun. Earth's day equals 24 hours= 1 earthen day.
cp.earth = 1 cal/gr*oC, it is because Earth has a vast ocean. Generally speaking almost the whole Earth’s surface is wet.
We can call Earth a Planet Ocean.
σ = 5,67*10⁻⁸ W/m²K⁴, the Stefan-Boltzmann constant
So = 1.361 W/m² (So - it is the Solar constant - the solar flux at the Earth's average distance from the sun).
Earth’s Without-Atmosphere Mean Surface Temperature Equation Tmean.earth is:
Tmean.earth = [ Φ (1-a) So (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴
Τmean.earth = [ 0,47(1-0,306)1.361 W/m²(150 days*gr*oC/rotation*cal *1rotations/day*1 cal/gr*oC)¹∕ ⁴ /4*5,67*10⁻⁸ W/m²K⁴ ]¹∕ ⁴ =
Τmean.earth = [ 0,47(1-0,306)1.361 W/m²(150*1*1)¹∕ ⁴ /4*5,67*10⁻⁸ W/m²K⁴ ]¹∕ ⁴ =
Τmean.earth = ( 6.854.905.906,50 )¹∕ ⁴ =
Tmean.earth = 287,74 Κ
And we compare it with the
Tsat.mean.earth = 288 K, measured by satellites.
These two temperatures, the calculated one, and the measured by satellites are almost identical.
.................................................
2. Moon’s Mean Surface Temperature calculation.
Tmean.moon
Surface temp..Tmin..Tmean..Tmax Kelvin
........................100.K...220.K...390.K
So = 1.361 W/m² (So is the Solar constant)
Moon’s albedo: amoon = 0,11
Moon’s sidereal rotation period in reference to the stars is 27,32 earthen days. But Moon also orbits sun, so the lunar day is 29,5 earthen days.
Moon does
N = 1/29,5 rotations/per day
Moon is a rocky planet, Moon’s surface irradiation accepting factor Φmoon = 0,47
(Accepted by a Smooth Hemisphere with radius r sunlight is S* Φ*π*r²*(1-a), where Φ = 0,47)
cp.moon = 0,19cal/gr oC, moon’s surface specific heat (moon’s surface is considered as a dry soil)
β = 150 days*gr*oC/rotation*cal – ( the Rotational Warming Factor constant ).
σ = 5,67*10⁻⁸ W/m²K⁴, the Stefan-Boltzmann constant.
Moon’s Mean Surface Temperature Equation Tmean.moon:
Tmean.moon = [ Φ (1 - a) So (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴
Tmean.moon = { 0,47 (1 - 0,11) 1.361 W/m² [150* (1/29,5)*0,19]¹∕ ⁴ /4*5,67*10⁻⁸ W/m²K⁴ }¹∕ ⁴ =
Tmean.moon = ( 2.488.581.418,96 )¹∕ ⁴ = 223,35 K
Tmean.moon = 223,35 Κ
The newly calculated Moon’s Mean Surface Temperature differs only by 1,54% from that measured by satellites!
Tsat.mean.moon = 220 K, measured by satellites.
.................................................
3. Mars’ Mean Surface Temperature calculation.
Tmean.mars
Surface temp..Tmin..Tmean..Tmax
Kelvin............130.K...210.K...308.K
(1/R²) = (1/1,524²) = 1/2,32
Mars has 2,32 times less solar irradiation intensity than Earth has
Mars’ albedo: amars = 0,25
Mars performs 1 rotation every 1,028 day
For Mars
N = 1 /1,028 = 0,9728 rotations /day (or 0,9728 marsian day /per an earthen day)
Mars is a rocky planet, Mars’ surface irradiation accepting factor: Φmars = 0,47
cp.mars = 0,18cal/gr oC, on Mars’ surface is prevalent the iron oxide.
β = 150 days*gr*oC/rotation*cal – ( the Rotational Warming Factor constant ).
σ = 5,67*10⁻⁸ W/m²K⁴, the Stefan-Boltzmann constant.
Mars' Mean Surface Temperature Equation is:
Tmean.mars = [ Φ (1-a) So (1/R²) (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴
Tmean.mars = [ 0,47 (1-0,25) 1.361 W/m²*(1/2,32)*(150*0,9728*0,18)¹∕ ⁴ /4*5,67*10⁻⁸ W/m²K⁴ ]¹∕ ⁴ =
=( 2.066.635.457,46 )¹∕ ⁴ = 213,21 K
Tmean.mars = 213,21 K
The calculated Mars’ mean surface temperature
Tmean.mars = 213,21 K is only by 1,53% higher than that measured by satellites
Tsat.mean.mars = 210 K !
................................................
So, we know how to calculate the PLANETARY TEMPERATURES.
After the initial insights, in the present research we demonstrate a typical scientific analysis.
And we have calculated the planet mean surface temperatures for all planets and moons. The calculations are in the next pages of the present site.
Table 1. Comparison of Predicted (Tmean) vs. Measured (Tsat) Temperature for All Planets and moons in solar system
Tmean = [ Φ (1-a) S (β*N*cp)1/4 /4σ ]1/4 (K) (3)
Table 1. Comparison of Predicted vs. Measured Temperature for All Planets
Distance Flux Factor Bond rot /day surface cal /gr.°C Warming.Factor °K °K °K °K
( AU ) ( W/m² ) Φ Albedo N Spin Type Cp (β*N*cp)¹∕ ⁴ Te Te.correct Tmean Tsat
Mercury 0,387 9082,7 0,47 0,068 0,00568 basalt 0,20 0,64250 439,6 364,0 325,83 340
Venus 0,723 2601,3 1 0,77 60/243 gases 0,19 1,6287 226,6 255,98 - 737
Earth 1,0 1361 0,47 0,306 1,0 ocean 1 3,4996 254 210 287,74 288
Moon 1,0 1361 0,47 0,11 0,0339 regolith 0,19 0,99141 270,4 224 223,35 220
Mars 1,524 586,4 0,47 0,25 0,9728 rock 0,18 2,26495 209,8 174 213,11 210
Ceres 2,77 177,38 1 0,09 2,645 ice 1 4,463 162,9 162,9 236 -
Jupiter 5,20 50,37 1 0,503 2,417 gases - - 102 102 - 165 at 1 bar level
Io 5,20 50,37 1 0,63 0,5559 rock 0,145 1,8647 95,16 95,16 111,5 110
Europa 5,20 50,37 0,47 0,63 0,2816 ice 1 2,5494 95,16 78,83 99,56 102
Ganymede 5,20 50,37 0,47 0,41 0,1398 ice 1 2,14 107,08 88,59 107,14 110
Calisto 5,20 50,37 1 0,22 0,0599 ice 1 1,7313 114,66 114,66 131,52 134±11
Saturn 9,58 14,84 1 0,342 2,273 gases - - 81 81 - 134 at 1 bar level
Enceladus 9,58 14,84 1 0,85 0,7299 ice 1 3,2347 55,97 55,97 75,06 75
Tethys 9,58 14,84 1 0,70 0,52971 ice 1 2,9856 66,55 66,55 87,48 86 ± 1
Titan 9,58 14,84 1 0,22 0,06289 gases 0,4980 1,47223 84,52 84,52 93,10 93,7
Uranus 19,22 3,687 1 0,30 1,389 gases - - 58 MM * - - 76 at 1 bar level
Neptune 30,33 1,48 1 0,29 1,493 gases - - 46,4 46,4 - 72 at 1 bar level
Triton 30,33 1,48 0,47 (?) 0,76 0,17021 rock 0,4116 1,800 35,4 29,29 33,92 38
2) Triton 30,33 1,48 1 (?) 0,76 0,17021 rock 0,4116 1,800 35,4 35,4 40,97 38
Pluto 39,48 0,874 1 0,50 0,1565 rock 0,248 1,5533 37 37 41,6 44
Charon 39,48 0,874 1 0,2 0,1565 ice 1 2,2014 41,90 41,90 51,04 53
-------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
"This new Vournas equation results are compared for twenty (20) solar system bodies (planets and moons), with the equation's calculated temperature closely matching the data, the NASA satellite measured temperature."
So we have a compelling argument that the temperature of planets, among the other parameters, also is strongly related to their speed of rotation, and to their average surface specific heat.
******************
So we have concluded:
Earth's average surface temperature is much higher than Moon's average surface temperature, and it is so because of a good reason.
Earth's average surface temperature is much higher than Moon's average surface temperature, because Earth rotates very much faster than Moon.
Actually Earth rotates 29,5 times faster than our Moon.
And also, because
the earth's surface is everywhere full of water, and so it has
cp =1 cal/gr*oC
which is five (5) times higher than that of moon's regolith surface, which has
cp =0,19 cal/gr*oC.
Opponent:
"Have you eliminated other variables such as the atmosphere and the oceanic heat sink?
There’s also the problem that 70% of the Earth’s surface is smooth ocean while the Moon’s surface is rough regolith. By your own logic the Moon should be warmer than the Earth because the Moon reflects less radiation.
Thank you, for your response.
-
The other variables, the earthern atmosphere, is very thin, that is why it doesn't make any significant difference (when comparing with the satellite measured Earth's Tsat =288K)
when we theoretically calculate earth's average surface temperature without atmosphere.
By the way, the theoretically "eliminating" any planet's surface atmosphere commenced with the theoretical effective temperature (Te) definition implementation.
-
"The 70% of the Earth's surface is smooth ocean while the Moon's surface is rough regolith."
-
Yes, but for Earth, Moon, Mars, Mercury, Europa and Ganymede the
Solar Irradiation Accepting Factor "Φ" is very much close
to the Φ =0,47
All the six the above mentioned celestial bodies reflect solar light very much close to a perfectly smooth spherical body.
-
"The Moon would be warmer than the Earth because the Moon reflects less radiation".
-
Yes, Moon reflects less solar energy, because
Moon's Albedo is a =0,11 vs Earth's
a =0,306
Nevertheless, Moon is on average much colder than Earth, because Moon rotates 29,5 times slower, and, also, moon's regolith has
cp =0,19 cal/gr*oC, which is five (5) times smaller, than that of earth's.
-
******************
The planet specular reflection was neglected
In the billions of years of their existence, planets and moons surfaces were subjected to natural influences (which were different for every planet and moon). Those continuous influences had developed either the smooth surface pattern, or the heavy cratered (rough surface) pattern.
There is not a 100% smooth surface planet or a 100% smooth surface moon.
And when we say a 100% smooth surface, what we mean by that is that the incident EM energy flux interacts with matter in a single one-touch strike.
When, for theoretical reasons, we consider a 100% smooth surface planet or a 100% smooth surface moon, then we (only theoretically), then we are dealing with the EM energy one-touch strike on the matter.
And when one-touch striking the matter, part of the incoming EM energy gets ("absorbed"), and part gets specularly reflected.
The reflection's outcome is a directional EM energy flux, but it has weakened, compared to the incoming incident solar EM energy flux, because some of the incident EM energy being ("absorbed").
When solar flux strikes a real planetary surface, it is not a one-touch interaction with matter process.
The directional (because of the large distance) EM energy flux from the sun, when interacting with planetary surface matter is subjected to multiple tiny specular reflections, as the EM energy first gets scattered upon the surface's matter.
And the macroscopic outcome is the outgoing diffuse reflection, "in which light is scattered away from the surface in a range of directions."
What we observe, when solar flux interacts with planetary surface's matter, what we observe as the macroscopic outcome is the surface's the diffuse reflection - it is what we see from some distance when looking at that surface. And that diffuse reflection is measured and determined as Planetary Albedo.
But, for smooth surface planets and moons (not 100% smooth), for those planets and moons there is also a strong directional constituent, because it originates from the incoming solar flux's EM energy, which enters from the opposite direction.
Thus,
for planets and moons with smooth surface, the surface's specular reflection is not negligible.
The smooth surface planets and moons have a very strong the surface's specular reflection.
The specular reflection is not included in albedo.
So we had (for those planets and moons with smooth surface, and, therefore, with surface's strong specular reflection), we had to correct their respective the planet effective temperature Te.
Thus, for Earth, the Te =255K, when corrected, became Te.correct =210K.
But, notice, it is very important:
The planet effective temperature, even when it is corrected, the planet effective temperature does not exist, the planet effective temperature is a mathematical abstraction.
*****************
The First Conclusions
Conclusions:
1). We have written the theoretically exact the planet mean surface temperature equation as a very much reliable theoretical formula:
Tmean = [ Φ (1-a) S (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴ (K) (3)
The theoretically calculated planets temperatures (Tmean) are almost identical with the measured by satellites (Tsat.mean).
2). We shall now compare the theoretically calculated Earth's (without-atmosphere) the average surface temperature (Tmean) with the satellite measured one, the (Tsat), because we are very much interested to estimate the magnitude of the atmospheric greenhouse effect.
Planet…......Te......Te.correct....Tmean….Tsat.mean
Mercury....440 K......364 K........325,83 K…..340 K
Earth……...255 K......210 K........287,74 K…..288 K
Moon……..270,4 K....224 K........223,35 Κ…..220 Κ
Mars……….210 K......174 K........213,11 K…..210 K
The planet mean surface temperature New equation is written for planets and moons WITHOUT atmosphere.
When applied to Earth (Without Atmosphere) the New equation calculates Earth's mean surface temperature as 287,74K, which is very much close to the satellite measured 288K.
3). Thus for the planet Earth the 288 K – 255 K = 33 oC difference does not exist in the real world.
There is NO +33°C greenhouse enhancement on the Earth's mean surface temperature.
Both the calculated by equation and the satellite measured Earth's mean surface temperatures are almost identical:
Tmean.earth = 287,74K = 288 K.
........................
Also, there is not any +33C atmospheric greenhouse effect on Earth’s surface.
Because we have written a Universal Equation which is valid for all planets and moons in solar system.
Earth is a planet, thus when the Equation calculates for Earth's surface the mean surface temperature Tmean = 287,4 K and the satellite measured the Earth's average surface temperature
Tsat =288K,
Then there is no room for any significant atmospheric greenhouse effect, much more there is not any +33C atmospheric greenhouse effect on Earth's surface.
*****************
It is all explained further on below.
*****************
Also, we are explaining about the actual reasons of Global Warming at the bottom side of this page.
The unknown nature of phenomena makes us to consider them as "black boxes".
It is what a small child does. A child tests everything, because a child doesn't know how everything works and why.
-
It is the only way we are learning.
-
When considering Earth’s average surface temperature behavior as a
“black box”.
And when comparing it with the rest planets, also considered as
“black boxes”.
And when they all (Earth included) appear with the same average surface temperature behavior…
There is the only conclusion:
Earth’s atmosphere doesn’t have any significant influence on the Earth’s average surface temperature.
So, there is not any +33C atmospheric greenhouse effect on Earth’s surface.
-
-
Also, we are explaining about the actual reasons of Global Warming at the bottom side of this page.
Now, about Φ -factor:
Φ - is the solar irradiation accepting factor (the planet surface spherical shape and planet surface roughness coefficient).
Φ =0,47 for smooth surface planets and moons without-atmosphere, (or with a thin atmosphere, Earth included), assumes the similarity with the measured
Drag Coefficient =0,47 for smooth spheres in the parallel flow fluids.
Link:
We have Φ for different planets’ or moon's surfaces varying
0,47 ≤ Φ ≤ 1
For ideal smooth planet or moon surface
the Φ = 0,47
And for a planet or moon with a very rough surface, which surface doesn’t exhibit specular reflection, (which is also idealistic)
the Φ = 1.
Planets and moons with a smooth surface have
Φ -factor very close to
Φ =0,47.
And planets or moons with a rough surface have
Φ -factor very close to
Φ = 1.
Only Triton (Neptune's moon), presumably, has Φ -factor somewhere in between, thus it is not possible for us to estimate its exact value.
Wikipedia
https://en.wikipedia.org/wiki/Albedo
Albedo (/lˈbiːdoʊ/ al-BEE-doh; from Latin albedo ‘whiteness’) is the fraction of sunlight that is diffusely reflected by a body.
Specular reflection - Wikipedia
"Specular reflection, or regular reflection, is the mirror-like reflection of waves, such as light, from a surface.[1]
The law of reflection states that a reflected ray of light emerges from the reflecting surface at the same angle to the surface normal as the incident ray, but on the opposing side of the surface normal in the plane formed by the incident and reflected rays. This behavior was first described by Hero of Alexandria (ADc. 10–70).[2]
Later, Alhazen gave a complete statement of the law of reflection.[3][4][5]He was first to state that the incident ray, the reflected ray, and the normal to the surface all lie in a same plane perpendicular to reflecting plane.[6][7]
Specular reflection may be contrasted with diffuse reflection, in which light is scattered away from the surface in a range of directions."
-
*****
********
An example would be specular reflections from water, which can only be perceived from a particular direction. Or sunlight reflected by a mirror. You cannot measure how much light is being reflected unless it is shining on your instrument.
-
An instrument flying on a satellite cannot detect reflection that is outside its view angle. Even with direct reflection, the angle of reflection could be outside the instrument’s view angle.
-
*************
“Bond Albedo is the fraction of power in the total electromagnetic radiation incident on an astronomical body that is scattered back out into space.
Because the Bond albedo accounts for all of the light scattered from a body at all wavelengths and all phase angles, it is a necessary quantity for determining how much energy a body absorbs. ”
–
–
“it is a necessary quantity for determining how much energy a body absorbs.”
But Albedo doesn’t account for the entire fraction of electromagnetic radiation incident on an astronomical body that is scattered back out into space.
The measured by satellites planetary Albedo accounts only on the (isotropical-like part of the scattered diffuse reflection), which can be seen by the satellite sensors, since it falls upon them.
But Albedo doesn’t account on the (directional-like part of the diffuse reflection) which escapes the satellite sensors orientation.
And it is the (directional-like part of the diffuse reflection) which resemblances with the "pure" specular reflection, which is always directional.
We observe that directional-like reflection on the Earth all the time.
And we usually see it as a kind of specular reflection.
Sun emits EM energy as a diffuse outgoing flux.
We are used to see our world in a diffuselly reflected illumination.
We see our Moon in a diffuselly reflected solar light.
What we see as
Specular reflection is the elementary light reflection when EM energy hits matter.
Part of EM energy gets specularly reflected and part is not reflected - ("absorbed").
-
Diffuse reflection consists of numerous tiny specular reflections.
-
Sun emits EM energy as a diffuse outgoing flux, sun emits EM energy as an almost isotropic outgoing flux.
The incoming to planetary surface solar EM energy, because of the large distance from the source (sun), the solar incoming EM energy appears as a directional flux of energy (rays).
When hitting a smooth surface area, some EM energy gets ("absorbed"), and some gets in its entity reflected in a simple (elementary reflection) way, the specular reflection - which is the one-touch interaction process. The elementary specular reflection outcome also consists from a directional EM energy outgoing flux.
-
Some planets and moons have smooth surfaces, though they are not 100% perfectly smooth. Thus, because of their surface being smooth, they may have along with the diffuse reflection a very strong specular reflection constituent.
-
Those smooth surface planets and moons, and therefore with a very strong specular reflection constituent, those smooth surface planets and moons are:
1). Mercury
2). Earth
3). Moon
4). Mars
5). Europa (Jupiter's satellite)
6). Ganymede (Jupiter's satellite)
-
The planet surface specular reflection constituent is a directional flux of EM energy. And, an instrument flying on a satellite cannot detect reflection that is outside its view angle. And, that is the reason, because the Strong Planetary Specular reflection was Neglected.
*************************
Whether a mirror is large or small, it reflects in a specular fashion – otherwise it would not be a mirror.
Again, because the planet specular reflection issue is very important:
-
Every infinitesimal point reflects specularly.
And every infinitesimal point reflects specularly according to its respective infinitesimal orientation to the incident solar flux.
As a result, an observer sees a scattered light, which looks like a diffuse reflection.
Since the incoming solar flux is directional, there always is a stronger scattering of reflected light towards the opposite direction. Thus the reflection of solar light is always a combination of diffuse and specular reflection.
Some planets and moons have surfaces which permit that infinitesimal point reflection to escape the planet surface. Those are the smooth surface planets and moons (Mercury, Earth, Moon, Mars, Europa and Ganymede).
The rest planets and moons have surfaces' features, which capture that primer infinitesimal point reflection (because, on those surfaces, the primer reflected light gets reflected multiple times, and only after, what is not absorbed, finally escapes surface) ,
thus those planets and moons do not let the directional reflection out, so their respective the out reflected light is entirely diffuse reflection.
Albedo is the measure of diffuse reflection. Thus for those planets and moons the
"radiative energy in" = (1-a)S.
But for the smooth surface planets and moons the
"radiative energy in" = Φ*(1-a)S.
-
*************************
Also, more about specular reflection at:
https://www.rp-photonics.com/specular_reflection.html
It is very important what it says:
” There can be also a combination of specular and diffuse reflection; an object with such properties exhibits specular highlights (depending on the illumination conditions) in addition to the appearance generated by diffuse scattering.
Specular reflections can appear much brighter than diffuse reflections, when seen from a large distance because the reflected light is concentrated to a smaller range of directions. (That can be a problem in the context of laser safety.) On the other hand, specular reflections remain unnoticed for an absorber if the reflected light misses his or her eyes.”
****************
It is not "a hypothetical solar irradiation accepting factor."
By "accepting" means how much energy a spherical shape body may "stop".
A spherical shape body may stop a flow of energy. Because of spherical shape a sphere
may stop Φ(1-a)S of total incident energy.
Φ varies from 0,47 to 1.
The smoother the surface is the closer to 0,47 the Φ is.
Thus, a smooth surface sphere with Albedo a = 0
should "absorb" 0,47*S.
A sphere with rough surface (Φ = 1) and
a = 0 should "absorb" a 100% S.
And a sphere with Albedo a = 1 should not "absorb" EM energy, regardless the values of Φ.
****************
Some surfaces exhibit almost “Lambertian reflectance”, where the diffuse reflection is almost the same in all directions.
-
https://en.wikipedia.org/wiki/File:Lambert6.gif
It looks like, but it isn’t. There is not a 100% "Lambertian reflection".
There are surfaces that exhibit almost "Lambertian reflectance", Moon doesn't. But there are celestial bodies, which exhibit the almost "Lambertian reflectance".
There are surfaces with "Not Lambertian reflectance" and there are surfaces with almost "Lambertian reflectance".
When solar energy hits surface's matter, the solar energy partly gets reflected-scattered.
1). The one-touch scattering is when the reflected solar energy is subjected to only one single reflection and then escapes from the surface. Because surface is smooth enough not to stop the reflected solar energy to leave.
Those surfaces exhibit the "Not Lambertian reflectance".
2). The multi-touch scattering is when the reflected solar energy is subjected to multiple-times reflections in the surface's "folds" and only then, the not absorbed solar energy escapes from the surface.
Those surfaces exhibit the almost "Lambertian reflectance".
-
***************
There is not a 100% "Lambertian reflection".
-
Planets and moons which exhibit the "not Lambertian reflection" have along with the diffuse reflection, also a strong specular-like reflection. So they have Φ = 0,47 (the solar irradiation accepting factor).
Those planets and moons are:
Mercury
Earth
Moon
Mars
Europa
Ganymede
All other planets and moons exhibit the almost "Lambertian reflection". This includes the 4 gaseous planets and Venus and Titan, which also exhibit "Lambertian reflection".
So for them the Φ = 1.
-
The incoming solar EM energy averaging is a mistaken thought experiment
“340W/m² reaches Earth from the Sun.”
Ok,
1360W/m² /4 = 340W/m²
But we cannot average the perpendicular to the planet cros-section cycle the incoming solar flux of So =1360W/m² , because there is neither solar radiation, nor Albedo at the planet dark-side area.
"Not a problem. Just accept that the solar flux and albedo on the night side of the Earth are zero. Then calculate the averages as usual."
-
Of course, I can do that.
But it is a mistaken theoretical thought experiment.
Solar EM energy doesn't "go" to the planet dark-side area. It is the planet rotating, and, while rotating, there is always half of the globe faces the sun.
Solar energy only interacts with the matter it is fallen on, solar energy doesn't interact when the surface is out of view, solar energy doesn't interact when the surface is on the planet's dark -side area.
Thus, we cannot average the incident on a planet solar energy's interaction result with the planet surface matter, we are not justified to average it over the entire planet surface, because solar EM energy interacts with surface only on the solar lit side area.
Solar EM energy at the instant of incidence on the planet surface what it does is to interact with the surface's matter.
At every point of incidence solar EM energy produces at that point the EM/surface interaction result.
The EM/surface interaction result is localized at that very point of EM energy incidence.
We cannot average the incident on a planet solar EM energy over some planet surface areas, because solar EM energy interacts with surface only at the point of incidence.
Because when the incident solar EM energy averaging, the new EM/surface interaction layout changes the actual EM/surface interaction result.
Solar flux cannot theoretically be averaged over some area, because solar flux what it does is to interact with matter.
When solar flux is averaged, the averaged flux is four (4) times weaker, therefore there would be a new interaction result, which would be very different from the actual one.
Solar flux cannot theoretically be averaged over some area.
Yes, because solar flux interacts with matter, it vanishes then.
And there has no much energy of the initial incidence left to be averaged then.
Like-wise we cannot average snow over the entire Global area, because snow belongs where it has fallen on.
Snow also vanishes at spring, but you cannot average the amounts of snow over the entire planet surface.
Of course the math would be correct, but in reality there is not snow at most of the planet surface areas.
Solar flux cannot theoretically be averaged over some area, because solar flux what it does is to interact with matter.
When solar flux is averaged, the averaged flux is four (4) times weaker, therefore there would be a new interaction result, which would be very different from the actual one.
The solar flux /Earth's surface interaction result comes up with the actual
Tmean = 288K.
The averaged solar flux's the new interaction result comes up with the impossible Te = 255K.
***********************************
Specular and diffuse reflection cannot be averaged over the entire planet surface – the dark side doesn’t reflect solar light.
–
The reflection happens on the solar lit hemisphere.
The solar flux, when perpendicular to the surface is ~1360 W/m².
It takes place somewhere around equator at local noon.
When Eqinox, it takes place at local noon on equator.
The sun is exactly above then, at that one particular point.
For the rest of solar lit hemisphere, sun is not exactly above.
At any given time sun's rays are perpendicular to planet surface only on one particular point.
The angle of incidence changes for all directions away from that one particular point, towards the terminator, and not only pole-ward.
So, there is only one particular point on Earth’s solar lit hemisphere surface every given moment, where sun is exactly above.
Thus the entire solar lit hemisphere reflects specularly, the lower is the sun the higher is the specular reflection ratio.
The lower is the sun, the larger is the cycle on the hemisphere, at which cycle the stronger is the specular reflection.
–
For more visit page: "Φ = 0,47 and FRESNEL"
**********************
How A Planet Retains The Solar Energy
There is the Solar flux (S), surface Albedo (a), the solar irradiation accepting factor
(the spherical shape and roughness
coefficient (Φ) ), and also, there is the Planet Surface Rotational Warming Phenomenon.
Solar flux (S) and surface Albedo (a) are two well-known and generally accepted physical terms when for the solar irradiated surface of a planet the
ENERGY IN = ENERGY OUT
equilibrium radiation balance is considered.
What is new in present research are
The solar irradiation accepting factor
(the spherical shape and roughness
coefficient (Φ) ), which is thoroughly explained further below,
and
The Planet Surface Rotational Warming Phenomenon
1). The faster rotation (N), the less differentiated the surface temperature - the higher the planet average surface temperature.
And, also, the faster rotating planet absorbs more solar energy.
************
2). A planet with a higher surface specific heat (cp) has also less differentiated the surface temperature - the higher the planet average surface temperature.
And, also, the higher is the surface specific heat, the more solar energy the planet absorbs.
************
Where The Additional Solar Energy Comes From
There is no additional solar energy involved in the Rotational Warming Phenomenon.
Here it is what happens:
Solar energy arrives at a planet's (Earth’s) orbit distance from the sun, falls on the spherical shape surface a planet has and interacts with the matter.
–
When interacting with matter there are three processes occur:
(SW) reflection,
(LW) emission and
(HEAT) absorption.
1). Some of solar energy is reflected at the same wavelengths it has arrived (as SW EM energy). It gets reflected both diffuselly and specularly.
2). Some gets transformed into (LW) EM energy and at that very instant gets emitted (without being absorbed).
( When solar energy interacting with the surface's the very upper skin layer, the not reflected solar energy goes both ways - some is (LW) emitted and some is conducted as HEAT into the surface's inner layers. )
3). And some gets transformed into HEAT and gets conducted as HEAT into the surface's inner layers and absorbed in the inner layers.
The not reflected portion of the incident solar flux (S) can be calculated as:
Φ*(1-a)*S (W/m²)
where
S - the solar flux (W/m²)
a - the satellite measured average Albedo
Φ - the solar irradiation accepting factor (the planet spherical shape and planet surface roughness coefficient)
Now, the quantity of transformed into heat and absorbed in inner layers portion of solar energy is expressed as:
(absorbed) = (Not reflected) - (LW emitted) (W/m²)
or
(absorbed) = Φ*(1-a)*S – [ 2). the (LW)] (W/m²)
***********
The portion of solar energy that instantly gets transformed into a (LW) and instantly emitted
the amount of
(LW emitted)
or
[ 2). the (LW)] (W/m²)
varies because of the Rotational Warming Phenomenon.
When a planet rotates faster (N), and when a planet has a higher the surface specific heat (cp), (everything else equals),
the amount of
(LW emitted)
or
[ 2). the (LW)] (W/m²)
is smaller, and the amount of the absorbed solar energy in form of HEAT is higher.
And that is how the Rotational Warming Phenomenon makes a planet (Earth) warmer.
Thus, there is no additional solar energy involved in the Rotational Warming Phenomenon.
It is that the warmer planet is able to retain more energy from the incident on its surface solar flux.
It is that the warmer planet, at the instant of solar flux' incidence, emits less outgoing (LW) EM energy, at the instant of solar flux' incidence, the warmer planet emits less outgoing (LW) EM energy than a colder one.
Notice:
The W/m² is referred (in every planet case) to an area which is perpendicular to the arriving solar flux’ intensity W/m².
************
***************
The Polar latitudinal areas' role
A planet absorbs only a small portion of the incident SW EM energy W/m² as heat.
Some of that heat gets transported to the poles.
The planet average surface temperature (Tmean) is fundamentally determined by the Radiative energy balance's quantitative magnitude (on how much EM energy enters and leaves the planetary boundaries):
Energy in = Energy out.
The Polar latitudinal areas' participation in Planet Radiative energy balance is very insignificant.
******************
**************************
**********************************
It is time to look elsewhere and retool.
So far, in present research, we have corrected the two mistakenly assumed observations.
1). A planet reflects the incident solar SW EM energy not only diffuselly, but also specularly. The specular reflection was neglected in planet radiative
Energy in = Energy out
balance equation, and thus the specularly reflected SW EM energy was mistakenly considered as part of the "Energy in".
2). When solar irradiated, a planet surface not only reflects and absorbs the incident SW EM solar energy, but at the instance of the SW EM solar energy incidence, a planet also emits (LW) EM energy.
Thus, the at the instance of the SW EM solar energy incidence, the by planet surface emitted (LW) EM energy was mistakenly considered as part of the "Absorbed".
Also visit page:
A NEW UNIVERSAL LAW
------------------------------------------------------------------------------
Earth is warmer than Moon, because Earth rotates faster.
At the same distance from the sun, Earth receives 28% less solar energy (higher Albedo), but Earth rotates
very much faster...
Earth's average surface temperature, in comparison with Moon's, is much HIGHER!
The planet or moon average surface temperature (Tmean) is dependent on the distance from the sun (the solar flux) and on the celestial body's average surface Albedo.
-
But there is something else very interesting happens!
What is new, is that the average surface temperature (Tmean) is SIGNIFICANTLY amplified by the Planet Surface ROTATIONAL WARMING PHENOMENON.
-
*****
-
Things get hot when placed in sunlight.
Surface IR emission is isotropic, and is emitted back into the free space around it.
And there is only a “small portion” that gets converted to heat and absorbed in the inner layers.
-
********
The specularly reflected solar energy should be considered in the planetary radiative energy balance estimation.
-
*****
-
The Planet Surface Rotational Warming Phenomenon is a UNIVERSAL PHENOMENON.
-
All planets and moons are INEVITABLY subjected to that UNIVERSAL PHENOMENON.
-
*****
-
Here it is a very well known scientific observation:
-
Earth’s surface is warmer than Moon’s on average +68°C.
–
But it happens so not because of Earth’s thin atmosphere very insignificant greenhouse effect.
–
Earth’s surface is warmer than Moon’s on average +68°C, because of the Planet Surface Rotational Warming Phenomenon!
********
-
We examine here the full scope of the issues.
-
“What is an average?”
Because not all the measurements are the same, we can average them, to see what we shall have (on average).
–
Now, when we have for two different celestial bodies the average surface temperatures so much different
Earth Tmean =288K
Moon Tmean =220K
We have to find out why it is happening so.
Is Earth on average warmer than Moon?
Yes, Earth is on average warmer than Moon.
But why Earth is on average warmer than Moon?
Because Earth rotates faster, and Earth is able to absorb much more solar energy, than Moon.
When a planet rotates faster, its surface absorbs more solar energy. Thus the planet becomes on average warmer.
Two basic physics planetary AXIOMS
We shall start our narrative with two very simple, but nevertheless very important basic physics planetary axioms.
1. The planet's equatorial mean surface temperature (Tmean.equatorial) is always higher than the entire planet's the global mean surface temperature (Tmean.global).
and
2. The faster a planet rotates, the bigger is the difference
Δt = Tmean.equatorial - Tmean.global
and, likewise, the slower a planet rotates, the smaller is the difference
Δt = Tmean.equatorial - Tmean.global.
-
*******
These two simple axioms led us to the following very important conclusions:
1. No matter how fast a planet rotates, planet surface never approaches a uniform surface temperature (Tmean.uniform).
and
2. For a very slow rotating planet the
Δt = Tmean.equatorial - Tmean.global
the difference "Δt" is very small, and for the entire planet surface, the global mean surface temperature (Tmean.global) is very close to the equatorial mean surface temperature value (Tmean.equatorial).
Opponent:
“Earth is a smooth rocky planet, Earth's surface solar irradiation accepting factor Φearth = 0,47”
Bond Albedo is intended to include ALL reflections.
There is not some 47% correction needed for “smooth” planets.
Thank you for your response.
–
" Bond Albedo is intended to include ALL reflections. There is not some 47% correction needed for smooth planets.”
(Emphasis added)
–
–
Let’s consider the planet Mercury then.
Mercury is a smooth planet, because its surface is formed from basalt (basalt resemblances glass).
https://en.wikipedia.org/wiki/Mercury_(planet)
Mercury rotates in reference to the sun very-very slowly.
N = 0,00568 rot/day
Thus planet Mercury's the satellite measured Equatorial mean temperature is very close to the planet Mercury's average surface temperature Tmean.
Albedo: 0,088 (Bond)
Temperature 437 K (164°C) (blackbody temperature)[13]
Surface temp. min mean max
0°N, 0°W [14] −173°C 67°C 427°C
Thus planet Mercury’s mean equatorial temperature is 67°C = 340 K
–
Let’s compare with planet Mercury's blackbody temperature 437 K
The blackbody temperature is almost 100°C higher.
But the corrected blackbody temperature Te.correct = 364 K,
which is very much closer to the measured equatorial
Tmean =340K.
Now, we see how it is important to correct the smooth surface planets and moons their respective blackbody temperatures (effective temperatures Te).
Satellite Era moved planet surface temperatures measurements at a New Level!
The NASA spacecrafts very precise measurements
The present research is based on NASA spacecrafts the solar system planets and moons the surface temperatures very precise measurements.
-
Also it is based on NASA spacecrafts the solar system planets and moons the surface reflectivity (Albedo) very precise measurements.
-
NASA has precisely measured the solar system planets and moons the rates of rotation.
-
Also NASA spacecrafts missions have determined for the solar system the various planets and moons the planetary surface's the different chemical composition.
-
******
A satellite orbiting planet measures the planet's every point surface temperature.
At day-time the satellite measures the instantly induced by sun the surface temperature.
At night-time the satellite measures the planet surface "the warmth" temperature.
When the measured temperatures being averaged over the entire planet surface - it is the planet average surface temperature (Tmean) - it is the mean surface temperature.
-
******
Yes, planets and moons do obey to the same universal laws, it is an axiom, but applied to different input parameters.
What we did in the present research was to compare the different planets and moons surface temperatures in accordance with the incident on the planet surface their respective solar flux (S), with their respective average surface Albedo (a), and, also, in accordance with their respective rate of rotation (N) , and with their respective surface specific heat (cp) and surface roughness features (the light capturing abilities).
-
In this research we have shown, and now we demonstrate that a planet average surface temperature (Tmean K) is determined by those five (5) major parameters:
1. The distance from the sun (solar flux "S" W/m²).
The intensity of the incident on a celestial body solar EM energy is dependent on the distance from the sun the square inverse law value.
2. The average surface diffuse reflectivity (Albedo "a"),
which is dependent on the planet's or moon's average surface the diffuse reflection properties.
3. The surface's spherical shape and the surface's roughness coefficient (the solar irradiation accepting factor "Φ").
Because planets and moons have very distinguished their respective surfaces features - there are the smooth surface planets and moons and there are the rough surface planets and moons.
The rough surface planets and moons almost do not exhibit the specular reflection from their respective surfaces.
4. The rate of rotation ("N" rotations/day),
which is a unique for every planet and moon value.
5. The average surface specific heat ("cp" cal/gr*oC),
which is determined by the planet the respective average surface chemical composition.
Yes, the same universal laws, but applied to different input parameters.
So the same universal physical laws, but applied to different celestial bodies.
And, there also is, the very POWERFUL the Solar Irradiated planet surface Rotational Warming Phenomenon.
*********
You can have all the theory in the world, but sometimes you’ll come across a problem that defies all logic and any theory you have learned.
When comparing the various different planets' and moons' (without-atmosphere, or with a very thin atmosphere, Earth included), when comparing the planetary surface temperatures, a very persistent question needs to be answered:
How can the planet average surface temperature (Tmean) increase without the radiation increasing?
******
And here it is when a major basic physics concept BREAKS THRU!
The importance of the proper use, and the importance of the proper understanding of the STEFAN-BOLTZMANN BLACKBODY EMISSION LAW!
Jemit = σT⁴ W/m²
The Stefan-Boltzmann blackbody emission law actually is THE RADIATIVE ENERGY EMISSION LAW!
The Stefan-Boltzmann blackbody emission law is a mathematical abstraction, which has a relation with some real bodies' emission intensity at some higher temperatures.
When the Stefan-Boltzmann blackbody emission law is applied to various material bodies there is a physics need to be considered those bodies as non perfect emitters, so those are gray bodies.
In order to continue applying the Stefan-Boltzmann emission law to the non perfect emitters - the gray bodies, the term emissivity (ε) was invented.
After applying the theoretical Stefan-Boltzmann emission law formula to the different for every material or surface condition (oxidized or polished etc...) after applying the different and empirically estimated (measured) emissivity (ε), the theoretical Stefan-Boltzmann emission law was established as the UNIVERSAL FORMULA when the bodies' and surfaces' the EM emission intensity was theoretically calculated.
-
What we conclude is that every material body and every material surface emits EM energy according to its absolute temperature fourth power ( T⁴ ) followed by a coupled coefficient (ε*σ), so it takes the final form of:
Jemit = εσT⁴ W/m²
The empirically estimated (measured) the emissivity coefficient (ε) depends on the emitting surfaces' content.
What exactly atoms and what exactly molecules are streched upon the m² of emitting surface.
The (ε) also depends on the atoms' and molecules' dimensions - how small or how large they are.
When there are more atoms are streched over the
m² of emitting surface, there are much more emitters, so the emissivity coefficient (ε) is higher.
When surface consists of larger atoms, there are much less emitters, so the emissivity coefficient (ε) is lower.
The Stefan-Boltzmann emission law is limited to the hotter bodies and surfaces.
When bodies and their respective surfaces are not hot enough, there is not enough thermal energy available to be transformed and emitted as EM energy in accordance to what the Stefan-Boltzmann emission law
Jemit = εσT⁴ W/m²
for those bodies and for those surfaces dictates.
The colder bodies still do emit EM energy, but their emission is much weaker, the colder bodies' EM energy emission intensities are much lower than the S-B emission law intensities.
******************
**********
The Stefan-Boltzmann emission law cannot be applied as it is to the EM energy (to the solar energy) irradiated surfaces.
-
The notion on a 2-way transfer of heat via radiation is wrong.
What they have done is apply the Stefan-Boltzmann equation in both directions, but the S-B equation is not about heat transfer, only radiation intensity of a surface at a temperature T.
They also have contradicted the basic quantum theory as laid out by Bohr in 1913. Bohr said that Electrons in atoms are the only particles that can transmit or absorb radiative energy, which is electromagnetic energy.
In fact, when a body radiates EM it loses heat at the same rate it emits the EM. Therefore the process IS A ONE WAY process.
When EM radiation of the same intensity hits another body, the resulting INTERACTION with matter doesn't put-in the same amount of heat being lost in the emission process by the first body.
The notion that heat can be transferred two ways using EM is an anachronism dating back to the 19th century, when scientists believed heat was transmitted through space via heat rays. Bohr proved that idea wrong in 1913.
Here is a definition of S-B which might suffice:
The amount of radiation emitted per unit time from an area A of a black body at absolute temperature T is directly proportional to the fourth power of the temperature T.
***
Says nothing about radiation or heat transfers, just relates the temperature of a surface to the radiation intensity it emits.
-
*********
The Stefan-Boltzmann emission law is NOT the RADIATIVE ENERGY absorption law!
It was mistakenly believed that we are scientifically justified when directly converting the solar EM flux into temperature!
Thus, the Planet Effective Temperature Equation:
Te = [ (1-a) S / 4 σ ]¹∕ ⁴ (K) Hansen et. al., (1981), Link: [22]
"Greenhouse Effect
The effective radiating temperature of
the earth, Te, is determined by the need
for infrared emission from the planet to
balance absorbed solar radiation:
πR²(1 - A)So = 4πR²σTe⁴, (1)
or
Te = [So(1 -A)/4σ]¹∕ ⁴ (2)
where R is the radius of the earth, A the
albedo of the earth, So the flux of solar
radiation, and σ the Stefan-Boltzmann
constant. For A ~ 0.3 and So = 1367
watts per square meter, this yields
Te ~255 K.
The mean surface temperature is
Ts ~288 K. The excess, Ts - Te, is the
greenhouse effect of gases and clouds,"
which is based on the mistaken assumption, that the Stefan-Boltzmann Blackbody Radiative Energy Emission Law FORMULA
Jemit = σT⁴ W/m²
which describes the blackbody surface at absolute temperature (T) the blackbody surface emission intensity in (W/m²)
It was mistakenly assumed that an irradiated with the same intensity (W/m²) a blackbody will develop the same absolute temperature (T).
Thus the above S-B Formula was very mistakenly
re-written as:
T = [S / σ ]¹∕ ⁴ (K)
and it was very much mistakenly applied to the real planet Infrared Emission BEHAVIOR.
Thus they had a planet surface radiative energy emission behavior being confused with the blackbody emission behavior.
(Which is very much different, because what a blackbody does is to emit EM radiative energy at absolute temperature (T), when what a planet does is to INTERACT WITH THE INCIDENT SOLAR FLUX.
***
*****
It is the matter's property to spontaneously get rid of energy. The matter gets rid of energy by the spontaneous EM (Electromagnetic) emission. And, the warmer (hotter) the object is, the more intensively the object emits EM energy.
So, the matter spontaneously emits EM energy in order to get rid of energy. When irradiated, the matter does not welcome the incident on its surface the incoming EM energy.
So, when EM irradiated, the matter does everything in its powers not to let in the incident energy. It does what ever it takes to absorb as little as possible.
Yes, when irradiated, the matter (on the very instant of incidence) reflects, emits and absorbs. And absorbs as little as possible.
When a planet surface is solar irradiated with some amount of EM energy, at the same very instant the surface INTERACTS WITH THAT AMOUNT of EM energy, and by doing so, the surface (on the very instant) SW reflects a portion of the incident EM energy, and (on the very instant) transforms some other portion from the SW into IR and emits it as IR outgoing EM energy, and what EM energy is left from SW reflection and IR emission, the planet surface (on the very instant) transforms it into heat and absorbs it in the inner layers.
Thus, when planet surface is solar irradiated:
at the same very instant the surface INTERACTS WITH THAT AMOUNT of EM energy and as a result
1). Some of SW gets reflected as SW
2). Some of SW gets transformed straight into IR (by omitting to decay as heat) which IR instantly gets emitted as IR
3). And the rest of the incident SW EM energy, gets transformed into HEAT, and that heat is what gets absorbed in the inner layers.
***
*******
*****
This special application (Te) derived from Stefan-Boltzmann, what Hansen calls the “Planet Effective Temperature Equation”, was used to recover the planet without-atmosphere the global average surface temperature (Tmean).
–
Yes, the (Te) “a special application derived from Stefan-Boltzmann” it is a good “working” name. Maybe to call it “the Hansen’s Equation”…
The
Te = [So(1 -A)/4σ]¹∕ ⁴ (2)
is based on a brilliant insight Hansen had, "The effective radiating temperature of
the earth, Te, is determined by the need for infrared emission from the planet to
balance absorbed solar radiation".
That it is how everything started! Hansen saw the possibility to THEORETICALLY calculate for a planet without-atmosphere the average surface temperature (Tmean).
It was neglected though, that a planet surface radiative energy emission behavior should not be confused with the blackbody emission behavior.
The EFFECTIVE RADIATING TEMPERATURE is the temperature of a body with a single approximate emission temperature that radiates the same power over its whole spherical surface as it receives as a disk from the sun, based on the albedo-reduced SOLAR FLUX.
This emission temperature should not be confused with any NORMAL planet average SURFACE temperature, because the Stefan-Boltzmann emission law cannot be applied to any kind of average surface temperature!
The solar FLUX's ratio of the PLANET’s surface area to its cross-sectional area is 4:1. Once again, the EFFECTIVE RADIATING TEMPERATURE is an equivalent uniform surface temperature, based on the SIMPLIFYING ASSUMPTION that a PLANET radiates like a STAR.
*******
The Planet Effective Temperature is only the FIRST STEP to mathematical APPROACH, and, therefore, the FORMULA:
Te = [So(1 -A)/4σ]¹∕ ⁴ (2)
is an IMPERFECT, and it is an INCOMPLETE equation for the Planet the Mean Surface Temperature (Tmean) the THEORETICAL calculation.
*******
A planet surface in radiative equilibrium with the sun has NOT any resemblance with the radiative equilibrium in the cavity with a small hole.
–
The planet average surface temperature (Tmean) is not a blackbody’s temperature.
–
Planet does not have a blackbody temperature, because planet has not a uniform temperature, and because planet is not a blackbody.
*******
It was used saying:
“NET incoming solar (what will be absorbed by the surface, incoming minus reflected away).”
–
We should say now:
NET absorbed solar (what will be absorbed by the surface, incoming minus reflected away, minus at the spot instantly IR emitted away).
*******
I mean, at the instant of the SW solar energy incidence to the surface, the IR emission takes place without an absorption. The incident SW solar energy gets IR emitted on that very spot, without being accumulated in inner layers as heat.
–
The result is that only a small portion is accumulated as heat and gets IR emitted later at night, or at different times.
–
Thus it is impossible to consider the incident solar flux to be averaged over the entire planet surface, because it is not averageble – the incident solar energy mostly is getting out from the sunlit side of a planet. Only a small part is converted to heat and gets accumulated.
The 240 W/m² has no physical meaning, has no physical analog, for radiative energy subjected spheroids (planet Earth) and, therefore, the planet Te =255K is simply a mathematical abstraction, and cannot be a comparison model for planet average surface temperature.
When based on the blackbody-planet theory, it was wrongly calculated:
"The earth's surface emits on average 240 W/m². "
-
Also it was very much wrongly concluded:
"Without greenhouse effect, Earth’s surface would be some 33°C (59°F) cooler.”
*********
Clearly there is something wrong
By accepting the 255K as an approximation, you accept the 255K or -18°C to emit the impossible
240 W/m².
Because the generally accepted physics say so. They have averaged the incident on Earth solar flux over the entire planet surface and came up with the 240 W/m².
It is known, from our everyday's practice, that a body does not emit the impossible high 240 W/m² at the very low temperature of -18°C.
In our homes, it is the fridges what produce to that very low temperature.
When outside in winter, at -18°C, there it is a deadly cold, there is nowhere any 240 W/m² emission to warm our bones a little bit.
Clearly there is something wrong.
Also, the inference is that ice, at 0°C, can transfer an energy of 315 W/m² of energy to a nearby body. So, if you have a square of ice 1 metre square, you should be able to put a body above it that is 1 metre square and warm it from the ice.
Clearly there is something wrong. The Stefan-Boltzmann emission law cannot describe the matter's EM emission at low temperatures.
-
And there is not any Back-calculatiǹg method permitted by the use of the Stefan-Boltzmann law.
Stefan-Boltzmann law is about a hot body's at uniform temperature the EM energy emission intensity.
Stefan-Boltzmann law doesn't describe the incident EM energy/ surfase matter interaction processes.
Also, the Stefan-Boltzmann law doesn't "work" at terrestrial temperatures, because it gives very much overestimated results.
Example:
It is said Earth, according to S-B law, as a uniform surface temperature sphere
emits 240 W/m² at Te=255K or -18°C.
I have experienced the -18°C what it is like. When outdoors at -18°C it is a deadly cold. There is nowhere 240 W/m² emitting.
A small 3m x3m x3m room at -18C according to S-B should emit:
3m x3m x6 = 9m^2 x6 = 54m²
54m² x240W/m² = 12.960 W or ~ 13 kW
Have you experienced 13 Kw heater in a small 3m x3m x3m room ?
Have you experienced how it is inside a refrigerator the size of 3m x3m x3m room at -18°C ?
Therefore the Stefan-Boltzmann law doesn't work at terrestrial temperatures, because it gives very much overestimated results.
****************
*******
The actual matter, which is made from atoms and molecules, does not have the necessary energy to support the expected IR EM emission levels the Stefan-Boltzmann theoretical equation dictates for the terrestrial and for lower than terrestrial temperatures.
***
***************
*******
Hot bodies do not radiate heat, they radiate electromagnetic energy (EM). If nothing intercept that radiation nothing can warm.
Therefore a planet interacts only with radiation. Should not get confused between interaction with radiation, and the thermal energy (heat) input.
The properties of EM are very different than the properties of heat. EM has no mass and is an electric field orthogonal to a magnetic field. It has a frequency. Heat has no frequency and cannot exist without mass. The two energies are of different physics behavior, thus when interacting with matter not the entire EM energy gets put in (not the entire EM energy gets absorbed).
–
***************
*******
The low temperature bodies have much less heat, and, therefore, they are not capable to emit their, according to Stefan-Boltzmann emmission law, the respective intensity EM radiation, those that the S-B law dictates.
–
And that is why, when S-B law is applied to planets and moons the IR emission intensities, the S-B respective to their absolute temperatures, the result is very much mistaken.
Thus we have the laughable 255K (-18C) emitting the impossible
240 W/m² !!!
–
Conclusion:
The S-B emission law application is limited by the energy a body is capable to give up.
If a body is over-loaded with energy, the energy is pouring out by EM radiation according to the S-B emission law, because at the emitting surface there is an instant equilibrium of energy transfer gets established – and establishing that equilibrium is what the Stefan-Boltzmann emission law is all about.
–
When there is much less energy in the body (at lower temperatures), it cannot support the IR emission intensity the S-B emission law dictates to emit at body’s respective low temperatures.
-
When deeping a thermometer in the lower temperature matter, thermometer is adjusted to the matter's temperature (the kinetic energy of molecules).
Molecules do vibrate at lower temperatures, but there is not enough energy to exitate the electrons in atoms, and they, the electrons, cannot emit the respective amounts of EM energy the S-B emission law dictates.
–
There’s nothing wrong with the Stefan-Boltzmann Law. It’s just that it is limited on the hotter bodies.
****************
********
******
The Temperature Graph (from Diviner) Of The Lunar Equator
This is a temperature graph (from Diviner) of the Lunar equator. As the sun light angle decreases to night it looks as if the temperature drops rapidly (much higher rate of radiant heat loss vs incoming solar energy).
Then at night, when cold, it does drop but much slower as the radiant loss is reduced significantly.
Links:
Lunar Surface Temperature Graph 1
–
Lunar Surface Temperature Graph 2
-
Lunar Surface Temperature Graph 3
-
Lunar Surface Temperature Graph 4
-
Let's analyse
As the sun light angle decreases to night it looks as if the temperature drops rapidly (much higher rate of radiant heat loss vs incoming solar energy).
–
What I think is that while the solar EM energy hits the surface, the solar EM energy interacts with the matter.
When interacting with matter:
1). Some of solar energy is reflected at the same wavelengths it has arrived (as SW EM energy). It gets reflected both diffuselly and specularly.
2). Some gets transformed into (LW) EM energy and at that very instant gets emitted (without being absorbed).
3). And some gets transformed into HEAT and gets absorbed in the inner layers.
********************
Thus, while the solar EM energy interacts with matter, it induces the skin layer’s measured the very high ~400 K temperature.
At the very moment the solar EM energy stops interacting with matter, the temperature instantly drops, because there isn’t any significant amount of heat being absorbed during the solar lit hours…
And
Then at night, when cold, temperature does drop but much slower as the radiant loss is reduced significantly.
At night the temperature drops in a linear relation, because the energy absorbed in inner layers during the day, the absobed heat, provided from inner layers, comes up to the surface according to the lunar surface regolith’s conductivity.
At night the lunar surface radiative cooling rate is determined by the regolith’s upgoing conductivity – there is not any other source of energy to support the night hours EM energy radiative emission.
–
The close analyse of the Graph confirms that the solar EM SW energy portion, which is not reflected as SW, it mostly doesn’t get absorbed in the lunar surface's inner layers.
The major part of not reflected SW EM energy gets at the instant transformed into the LW outgoing EM energy, which leaves to outer space.
Only a small portion gets absorbed as heat and, later at night, it is IR emitted to outer space.
–
Also we see in the Graph, that at almost the very instant of solar incidence at the next morning, the surface temperature rises at a tremendous high rate from ~100 K to ~ 400 K.
Thus we can conclude, that the arriving solar SW EM energy on the instant of incidence induces the skin layers high temperature, with very little of that EM energy being absorbed.
****************
********
*****
The faster rotation prevents a planet to get much warmed at day-time. Thus it prevents it from emitting during the day-time hours much IR outgoing EM energy.
Now, please compare the above lunar, at the local lunar noon the upwelling IR, with the very important (Godwin-Creek-MS-upwelling-IR-).
https://i.postimg.cc/sXhYqDNR/SURFRAD-Godwin-Creek-MS-upwelling-IR-050423.png
Because…. The faster rotation (EARTH) prevents a planet to get much warmed at day-time. Thus it prevents it from emitting during the day-time hours much IR outgoing EM energy.
***************
********
*****
I think planets and moons absorb some of the not reflected portion of solar EM energy at solar lit hours by transforming part of it into HEAT and get warmed, and release EM energy at dark hours at night and get cooled.
–
The rate of cooling slows the cooler surface gets. The S-B emission law is limited to work on much higher than terrestrial temperatures, when an abundance of thermal energy makes it pouring out
at its highest rate of ~ ε*σT4.
–
The emittance from surfaces at lower temperatures doesn’t happen
at the highest ~ σT4 rate.
–
And I think that is the reason why planets and moons in solar system (Earth included) still hold enough of their primordial thermal energy.
****************
********
*****
Quote:
“If there is something very slightly wrong in our definition of the theories, then the full mathematical rigor may convert these errors into ridiculous conclusions.”
Richard Feynman
Also, something else I have to quote is Gandhi's saying...
"A mistake does not become truth because it is widespread, nor does truth become wrong because no one sees it."
-
And:
"Because everything is cognized in comparison."
It means you can only know this or that thing for real if you compare it to something else.
The authorship of the phrase "everything is cognized in comparison" belongs to the great French philosopher-Cartesian Rene Descartes.
-
**************
No, the Earth does not emit on average 240 W/m².
-
In addition, Earth is a planet. A planet is irradiated from one direction only, and a planet rotates. A planet's average surface temperature cannot be estimated by simply averaging over the entire planet surface the not reflected portion of the incident solar flux.
-
No, without greenhouse effect, Earth’s surface would NOT be some 33°C (59°F) cooler.
Because there is not a 33°C (59°F) greenhouse effect on Earth's surface.
-
When solar irradiated, a planet surface does not absorb the entire not reflected portion of the incident solar energy.
What planet surface does is to INTERACT with the incident solar flux.
Only a small portion of the incident solar energy a planet surface absorbs in inner layers.
***
-
So, it is actually a completely different approach, it is a completely different mechanism when describing the planet radiative energy balance, the
Energy in = Energy out
which (in radiative equilibrium) should be necessarily met.
–
The above naturally begs for the question:
Where to goes the not absorbed solar energy then?
The not absorbed SW solar energy, when interacting with planetary surface’s matter, the surface’s skin layer gets warmed, and instantly transforms the SW solar energy into the LW (IR) outgoing radiative EM energy.
The temperature (at every spot) developed by the surface’s skin layer is measured by satellite sensors and that skin layer’s temperature is considered as the spot’s surface temperature.
The spot’s skin layer’s temperature is a superficial temperature. That temperature doesn’t represent the temperature the surface’s inner layers are at.
Because the not reflected SW solar energy is not entirely absorbed in surface’s inner layers.
-
***
When a planet rotates faster, its surface temperatures are less differentiated.
Also, when a planet rotates faster, its surface absorbs more solar energy.
Thus the planet becomes on average warmer.
But when a planet rotates faster, its day-time temperatures lessen and the night-time temperatures rise. So there is the self limiting factor:
The planet average surface temperature (Tmean) is always less than the day-time average temperature.
So when N2 > N1
Tnight↑→ T↑mean ← T↓day
-
But, the night-time temperatures rise more (the night-time temperatures AMPLIFICATION - because of the non-linearity of EM energy emission law), than the day-time temperatures lessen. Thus on average (as a whole), when faster rotating, the planet surface is always warmer.
************
There is a widely known MATHEMATICAL CONSTRAINT
For identical spheres emitting the same exactly amount of IR EM energy, for those with higher differentiated surface temperatures, the average surface temperature (Tmean) will be lower.
Thus, the higher the spheres' differentiated surface temperatures, the lower their average surface temperature.
So, consequently, the spheres with UNIFORM (not differentiated) surface temperatures will have the highest (the maximum) AVERAGE surface temperature.
For them,
Tmean(maximum) ≤ Tuniform
-
It is true for identical spheres emitting the same exactly amount of IR EM energy.
-
We should mention here, that those spheres emit the same exactly amount of IR EM energy, but the source (or sources) of that emitted energy are originated from the spheres' inner layers. That energy comes from the inside of the spheres.
Thus that mathematical constraint cannot be applied to the planets and moons the surfaces' temperatures estimation.
Because, for planets and moons, the source of emitted IR EM energy is very much different: for planets and moons, the source of emitted IR EM energy originates from the INTERACTION with SOLAR IRRADIATION.
-
The widely known and the widely used, when considering the real bodies' the emission temperature - the physical term emissivity (ε), cannot be applied when the source of EM radiative emission energy originates from the EM/surface INTERACTION PROCESS from the incident on that surface an outer EM radiative energy.
Thus,
The Planet Effective Temperature FORMULA:
Te = [So(1 -A)/4εσ]¹∕ ⁴ (1)
is always written with ε =1, that is why, after simplification, it has its usual form of appearance as:
Te = [So(1 -A)/4σ]¹∕ ⁴ (1)
and which calculates for Earth the theoretical effective temperature as:
Te = 255 K
but the ε = 1 is for spheres emitting their inner source's heat transformed on their surface into IR EM radiative energy.
For planets and moons, their source of energy is already radiative energy, what they emit as IR EM radiative energy is the result of interaction of the incident radiation with the surface (with the matter).
-
There is NOT for the EM energy interaction process, which results in the surface's IR emission, there is NOT any kind of surface emissivity term to be applied.
So, under those, very much different circumstances, there is NOT any room for the real planets the average surface temperatures to be compared with their respective theoretical effective temperatures.
-
Earth's oceanic waters do have emissivity close to ε = 1 , but there is NOT possible to the term emissivity being applied, when incident solar EM energy induces the waters to emit IR (to re-emit the SW as IR) - there is NOT any room for the emissivity term to be applied.
Therefore, the above MATHEMATICAL CONSTRAINT also cannot be applied, when we consider for real planets and moons the actual emission behavior.
The planet (or moon) EFFECTIVE TEMPERATURE, which is a pure theoretical ABSTRACTION, cannot be accepted as the planets' (or moons') Mathematical CONSTRAINT
Teffective = Tmean(maximum),
which mistakenly led to the very much confusing conclusion, that planet (or moon) without-atmosphere, the average surface temperature (Tmean) would be constrained to be less than or equal to the Teffective:
Tmean ≤ Teffective
-
***
Of course, (everything else equals), for planets and moons, the less their surface temperatures are differentiated, the higher their average surface temperatures are.
But the theoretical Teffective does not pose any Mathematical CONSTRAINT to planets' and moons' the average surface temperatures (Tmean).
-
***
The real subject matter is the reality of a dynamic process of a fast spinning ball lit by incoming radiation of 1.362 W/m² from one direction.
*******
A larger question presents itself. Why don’t we just observe the real-time physical processes as they occur on the Solar System's the Various Planets' surfaces and then draw our conclusions from those direct observations, ones being made in real time as the physical processes themselves are happening?
Said differently, the planets' surfaces themselves, as they exist in the real world, might become the ‘computational computer’ which, by the use of planet surface's major parameters will be able to theoretically calculate the expected global mean surface temperatures very much close to those measured by satellites.
Instead of plugging numbers into a physics-based, hand-tuned models, we look at all planets surface's major parameters as an interconnected vector fields.
Last time updated:
October 14, 2024
*******************************
********************
************
We do the planets surface temperatures comparison as our method
What we do in our research is to compare the satellite measured planetary temperatures.
There are not two identical planets or moons in solar system.
Nevertheless all of them, all planets and moons in solar system are subjected to the same ROTATIONAL WARMING PHENOMENON!
The Planet Surface Rotational Warming Phenomenon is expressed QUANTITATIVELY.
It appears to be a very POWERFUL the planet surface warming factor.
The Planet Surface Rotational Warming Phenomenon:
It is well known that when a planet rotates faster its daytime maximum temperature lessens and the night time minimum temperature rises.
But there is something else very interesting happens. When a planet rotates faster it is a warmer planet.
The Earth seen from Apollo_17
Thus, we have demonstrated that the planet mean surface temperature (Tmean) is amplified by the Planet Surface Rotational Warming Phenomenon.
Next, we formulated the Planet Mean Surface Temperature NEW Equation:
Tmean = [ Φ (1-a) S (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴ (K) (3)
Where:
Φ- is the Planet Surface Solar Irradiation Accepting Factor (the planet spherical shape and the planet surface roughness coefficient).
The Φ -Factor is what made it possible the precise estimation of the NOT REFLECTED portion of solar flux.
In the appended pages we have thoroughly analyzed and explained the Φ -Factor's origine, the Φ -Factor's importance, and the Φ - Factor's variants.
Φ(1 - a) - is the planet surface coupled term (it represents the NOT REFLECTED portion of the incident on planet surface solar flux, it is the portion of solar flux which gets in INTERACTION processes with the planet surface).
β = 150 days*gr*oC/rotation*cal – ( the Rotational Warming Factor constant ).
-
*****
In the current work, we use these exact two concepts, the Planet Surface Rotational Warming Phenomenon and the Solar Irradiation Accepting Factor (Φ).
We discuss the difference between the Hansen's formula on the one hand, and the New Equation's on the other hand, and test the hypotheses that Planet Mean Surface Temperature (Tmean) can be Theoretically calculated by mathematical methods.
After having addressed this core point, we performed the calculations based on the available data to see how they fit.
And, we ended up to the following remarkable results:
Earth is warmer than Moon, because Earth rotates faster!
Comparison of results the planet's Te calculated by the Incomplete Equation (the Planet Effective Temperature Te):
Te = [ (1-a) S / 4 σ ]¹∕ ⁴ (K) (1)
the planet's mean surface temperature Tmean calculated by the Planet's Without-Atmosphere Mean Surface Temperature New Equation:
Tmean = [ Φ (1-a) S (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴ (K) (3)
and then we proceed comparing with the planet's average surface temperatures, the actual Tsat.mean measured by satellites:
To be honest with you, at the beginning, I got by surprise myself with these results.
Correlation is not causation, but the match is striking.
Because I was searching for a mathematical approach…
We have collected the results now:
Planet….........Te..........Tmean…..Tsat.mean
Mercury.....439,6 K.....325,83 K…..340 K
Earth……....255 K……....287,74 K…..288 K
Moon……....270,4 K…….223,35 Κ…..220 Κ
Mars……....209,91 K…..213,21 K…..210 K
the calculated with Planet's Without-Atmosphere Mean Surface Temperature Equation and the measured by satellites are almost the same, very much alike.
Because it is a situation that happens once in a lifetime in science.
.
The Control Volume Approach
For energy transfer problems, the control volume approach involves studying the flow of energy across the boundaries of the control volume. This is done by considering the energy flux, at each boundary of the control volume. By applying the conservation of energy to the control volume, we can then derive an energy balance equation, which relates the rate of change of internal energy within the control volume to the net rate of energy transfer across its boundaries.
–
***
It is exactly what we do when deriving to the planet mean surface temperature NEW EQUATION (Tmean).
–
What we do in present research is to consider the planets’ surfaces energy transition boundaries in their entirety for every planetary surface.
–
A planet surface interacts with incident solar flux as a whole (IN TOTAL), and not in average surface, as it is very much wrongly asserted by the current scientific consensus.
–
While interacting a planet responds to the incoming solar energy AS A WHOLE, planet responds to the incoming solar energy with all its major characteristic features, or, in other words, planet responds with the entire “set” of the planetary surface qualities.
–
–
1). For the six smooth surface planets and moons
Mercury
Earth
Moon
Mars
Europa
Ganymede
the specular reflection is very strong, but it is being ignored as insignificant. Thus it led to “energy in” much higher estimations.
2). The “Energy in = Energy out” concept is about the black box, which is an open system with a boundary.
You study the inputs and outputs across the boundary without necessarily knowing what goes on inside.
(In GHE theory the average surface temperature differs because of the rising greenhouse gases content.)
In my point the solar energy “Energy in = Energy out” concept is the basic concept, it should be necessarily met.
The average surface temperature is a measured value. So we know what the planets’ and moon’s the average surface temperatures are.
And yes, “Energy in = Energy out”, but the energy interacts with surface’s matter. When interacting the average surface temperature occurs.
–
–
There is a well known scientific POSTULAT:
When two identical spheres emitting the same amount of EM energy, the less surface temperature differentiated the higher the average surface temperature.
What is New, is that when considering spheres (planets or moons), which are getting warmed by incoming EM energy, because they are solar irradiated, the less surface temperature differentiated the more solar energy the planet or moon absorbs!
–
And it is the “black box”, or the radiative equilibrium.
When the radiative equilibrium gets “switched” up – the average surface temperature rise.
When it is “switched” down – the average surface temperature lessens.
In my opinion the currently observed global warming is not due to CO2 (not due to fossil fuels intensive burning), but because of orbital forcing, because of the current orbital circumstance our planet Earth is subjected to.
–
The Planet Effective Temperature Equation
Te = [ (1-a) S / 4 σ ]¹∕ ⁴ (K) (1)
is incomplete because it is based only on two parameters:
1. On the average solar flux S (W/m²) on the top of a planet’s atmosphere and
2. The planet’s average Albedo "a".
The planet's without-atmosphere mean surface temperature equation has to include all the planet surface major properties and all the characteristic parameters.
3. The planet's axial spin N (rotations/day).
4. The thermal property of the surface (the average specific heat cp).
5. The planet surface solar irradiation
accepting factor Φ ( the spherical shape and the surface roughness coefficient).
Altogether these parameters are combined in the Planet's Without-Atmosphere Mean Surface Temperature New Equation:
Tmean = [ Φ (1-a) S (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴ (K) (3)
Consequently, the planet mean surface temperature Tmean is based on Stefan-Boltzmann emission law,
and on precise estimation by planet surface the total amount of emitted energy
πr²Φ*S*(1-a) (W)
and on the different for each planet the energy emission distribution (the temperatures distribution) over surface area - resulting in the very POWERFUL
the Planet Surface Rotational Warming Phenomenon.
( ...on the way the energy emission is distributed over the entire planetary surface – the Planet Surface Rotational Warming Phenomenon. )
*******************
Let's consider another pair of planets and their satellite measured mean surface temperatures:
Moon and Mars (220K vs 210K)
Mars is at 1,5 AU from the sun, thus Mars receives 2,32 times less solar energy on its surface than Moon.
Also Mars has higher than Moon average Albedo (0,25 vs 0,11). It can be shown that if Moon had the same as Mars Albedo, Moon's mean surface temperature would be 210,8K, therefore it would be almost equal to Mars' mean surface temperature of 210K.
Thus Mars receives 2,32 times less solar energy than Moon, yet Mars and Moon would have (for equal average Albedo) the same mean surface temperature 210K.
-
Therefore, there is only the Rotational Warming Phenomenon what justifies for Earth and for Moon, the measured, but the so very much the different, the mean surface temperatures (288K vs 220K).
–
And also, therefore, there is only the Rotational Warming Phenomenon what also justifies, now in the case of Moon and Mars, the measured, but this time the so very much the proximate, the mean surface temperatures (220K vs 210K).
Rotational Warming Phenomenon justifies for Earth and for Moon, the measured, but the so very much the different, the mean surface temperatures (288K vs 220K).
-
Rotational Warming Phenomenon also justifies, in the case of Moon and Mars, the measured, but this time the so very much the proximate, the mean surface temperatures (220K vs 210K).
********************************
The Planet Effective Temperature (Te) is the very important insight.
"The effective radiating temperature of
a planet, Te, is determined by the need for infrared emission from the planet to
balance absorbed solar radiation".
The planet (Te) is a brilliant mathematical abstraction.
Nevertheless, there is an important reason to change the word "absorbed" with "not reflected". Because we found that only a small part of not reflected solar radiation is actually getting absorbed in inner layers.
For EM irradiated surfaces the "not reflected" EM energy does not equal with the "absorbed".
A large portion of the not reflected energy, on the very instance of incidence, without being absorbed, gets instantly transformed into IR outgoing EM energy.
Thus, the above the planet (Te) determination should be read as:
"The effective radiating temperature of
a planet, Te, is determined by the need for infrared emission from the planet to
balance the not reflected solar radiation."
After the word "absorbed" is changed with the "not reflected", the planet (Te) still remains a brilliant mathematical abstraction.
********************************
The rightness of the Rotational Warming Phenomenon is many times demonstrated and, also, it has been theoretically explained by the physics first principles.
-
The planet Radiative "Energy In" is ruled by the three major parameters:
1. The intensity of Solar flux "S" (W/m²), which is defined as the solar energy intensity perpendicular to the planet cross-section cycle (it is the proximity to the sun dependent value).
-
2. The planet average surface Albedo "a".
-
3. The planet surface Solar Irradiation Accepting Factor "Φ" (in other words - the planet surface spherical shape and the planet surface roughness coefficient).
-
All those three major parameters are combined in the Radiative
"Energy In" Equation:
Energy In = Φ*(1-a)*S (W/m²)
-
Also, the planet mean surface temperature Tmean is amplified by the Planet Surface Rotational Warming Phenomenon.
-
****
Recognizing the difference between what theory suggests and practical knowledge demonstrates is critical.
Academics have the luxury of focusing on one or a limited number of parameters at a time. The traditional scientific method of hypotheses testing through experimentation is better suited to studies involving limited numbers of variables. Wicked complex systems full of all sorts of inconvenient interactions and feedback tend not to always work as might be suggested by theory from the "settled" sciences.
-
The planet blackbody equilibrium temperature Te (the planet effective temperature) was the first scientifically supported attempt to theoretically estimate the expected planet mean surface temperature Tmean.
-
It was the brilliant insight - to apply the planet radiative energy ballance (Energy in = Energy out), and to attribute to the "Energy out" the planet mean surface temperature Tmean THE TOTAL infrared outgoing radiative energy.
-
This brilliant approach had two serious basic science cavets though.
1. Planets are not blackbodies.
2. The Stefan-Boltzmann emission law cannot be applied wice-versa (we cannot calculate the radiated surface's temperature by simply measuring the incident on the surface radiative energy flux.)
-
Also it was omitted that the planets have spherical shape and that the different planets' surfaces may have very much different levels of roughness. The planets' spherical shape and the planets' surface roughness play a major role in solar irradiation- planet surface interaction processes.
-
****
Also, we are explaining about the actual reasons of Global Warming at the bottom side of this page.
................................................
Now we can explain why the planet Mars’ average surface temperature Tsat.mean = 210K is the same as Mars’ theoretically calculated effective temperature Te = 210K
In the New Tmean equation in the case of Mars the
(β*N*cp)¹∕ ⁴
and
Φ
eliminate each other, because in the case of Mars, by a pure coincidence
Φ = ~ 1 /(β*N*cp)¹∕ ⁴
or
0,47 = ~ 1 /(150*0,9728*0,18)¹∕ ⁴ = 0,441138
( 0,47 /0,44 )¹∕ ⁴ = (1,0682)¹∕ ⁴ = 1,0166
so there is only a
1,66 % difference in the final result of Te =210K and Tsat.mean =210K
Thus we have here a clear confirmation of the rightness of Φ =0,47 for the smooth surface planets and moons.
*********************
In the New Tmean equation in the case of Mars the
(β*N*cp)¹∕ ⁴
and
Φ
eliminate each other, because in the case of Mars, by a pure coincidence
Φ = ~ 1 /(β*N*cp)¹∕ ⁴
********************
It is not a circular resoning. It is an observation, which proves right the initial insight, which assumes the similarity of the
Φ =0,47 for smooth surface planets and moons without-atmosphere, (or with a thin atmosphere, Earth included), assumes the similarity with the measured
Drag Coefficient =0,47 for smooth spheres in the parallel flow fluids.
Link:
****************************
We have calculated The Planet Mean Surface Temperatures by the use of the New Equation:
Tmean = [ Φ (1-a) S (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴ (K) (3)
for all the twenty (20) major planets and moons in solar system. The results are very close to the satellite measurements.
The theoretically calculated results for all the twenty (20) major planets and moons in solar system being very much precisely close to the measurements performed by satellites is a sufficient and necessary condition, which states for the rightness of the Planet Surface Rotational Warming Phenomenon.
Also we have estimated the:
accurate values for (average) albedo, cp, β, Φ, S, with minor uncertainties for each.
thus there is no need for propagation of the uncertainties for the total equation, as a formal scientific research method requires.
because our calculations come so close to the measured for Earth, I think is not at all luck but the result of a thorough analysis.
*****
******
The detailed Mean Surface Temperatures calculations for each and every planet and moon in solar system, by the use of the New Equation, are posted in the next pages of this site.
******
*****
Albedo
“Albedo (/ælˈbiːdoʊ/; from Latin albedo ‘whiteness’) is the fraction of sunlight that is diffusely reflected by a body. It is measured on a scale from 0 (corresponding to a black body that absorbs all incident radiation) to 1 (corresponding to a body that reflects all incident radiation).”
–
“is the fraction of sunlight that is diffusely reflected by a body.”
https://en.wikipedia.org/wiki/Albedo
The planet specular reflection was neglected
Smooth spherical shape objects have not only the solar light diffuse reflection, but also they have the solar light a strong mirroring reflection constituence, where there is the phenomenon of specular reflection involved.
Specular reflection is very well demonstrated when we "catch" solar light with a mirror.
The reflection of solar light by the mirror is blinding. It is so much strong, it is almost like looking at the sun with a naked eye.
When out in the street, turn your back to the sun. The illuminated asphalt is seen as a solar light diffuse reflection.
But when we turn towards the sun, when looking at asphalt, we need to narrow our eyes, because the reflection is much more intense.
It is happening so, because of the solar illuminated asphalt reflecting the combination of diffuse and specular reflected solar light.
When looking at the sea, we watch the open water areas the solar specular reflection.
Also, when we look from some elevation to the plain areas, we observe there solar specular reflection too.
But when we are looking at the Moon, we see the solar illuminated Moon in diffuse reflection only.
It happens so, because of the Moon's spherical shape, and the specular reflected from Moon's surface solar light, which is a directional light, cannot be seen from Earth's distance.
The specular reflected light from Moon does not go towards Earth's direction.
The same with spacecrafts, orbiting planets and moons.
The spacecrafts sensors do not "see" the specular reflection, because it goes the different directions, and doesn't fall on the spacecrafts.
And it was the planet surface Lambertian reflectance considerations (or diffusely reflecting surface) the cause to wrongly estimate the planet specular reflection as a negligible value.
https://en.wikipedia.org/wiki/Lambertian_reflectance
When, for planets and moons with smooth surface, the specular reflection was neglected, it resulted to the very much mistakenly for the planet radiative "energy in" estimation.
For planets and moons with smooth surface, the surface's specular reflection is not negligible.
The smooth surface planets and moons have a very strong the surface's specular reflection.
So we had (for those planets and moons with smooth surface, and, therefore, with surface's strong specular reflection), we had to correct their respective the planet effective temperature Te.
Thus, for Earth, the Te =255K, when corrected, became Te.correct =210K.
But, notice, it is very important:
The planet effective temperature, even when it is corrected, the planet effective temperature does not exist, the planet effective temperature is a mathematical abstraction.
*******
*****
How much of the incident on a planet surface solar flux’s radiative energy a planet can absorb?
-
What portion of the incident on planet surface solar flux's radiative energy gets transformed from SW incident into the IR emitted (the IR outgoing) energy?
-
Io (Jupiter’s satellite), Io’s Albedo a =0,62
–
Moon (Earth’s satellite), Moon’s Albedo a =0,11
–
Why those Albedo are so much different?
*****
Earth’s Albedo without clouds is a =0,08
Europa's Albedo a =0,62
–
Why there is so much difference?
-
Yes, we are approaching now the second very much important concept of this research:
Planets and moons (without-atmosphere, or with a very thin atmosphere, Earth included) may have very smooth planetary surfaces, or they may have very much cratered (the heavy cratered) planetary surfaces.
The smooth surface planets, when solar irradiated, exhibit a very strong specular reflection, which cannot be measured by satellites (as a supplementary portion of Bond Albedo), because the specular reflected portion of solar flux does not enter into the satellite's sensor.
The heavy cratered surface planets, when solar irradiated, do not exhibit specular reflection, because the incident solar flux is subjected there to multiple reflections within the planetary surface craters, and thus, its radiative energy being captured and absorbed - not having the ability to escape as a specular reflection.
-
***
We demonstrate here that the Lambertian reflectance concept has very mistakenly influenced the planet radiative "energy in" estimation.
Lambertian reflectance - Wikipedia
"Lambertian reflectance is the property that defines an ideal "matte" or diffusely reflecting surface. The apparent brightness of a Lambertian surface to an observer is the same regardless of the observer's angle of view.[1]More precisely, the reflected radiant intensity obeys Lambert's cosine law, which makes the reflected radiance the same in all directions. Lambertian reflectance is named after Johann Heinrich Lambert, who introduced the concept of perfect diffusion in his 1760 book Photometria.
Examples[edit]
Unfinished wood exhibits roughly Lambertian reflectance, but wood finished with a glossy coat of polyurethane does not, since the glossy coating creates specular highlights. Though not all rough surfaces are Lambertian, this is often a good approximation, and is frequently used when the characteristics of the surface are unknown.[2]
Spectralon is a material which is designed to exhibit an almost perfect Lambertian reflectance.[1] "
********
The Bond Albedo is the fraction of power in the total electromagnetic radiation incident on an astronomical body that is scattered back out into space.
Because the Bond albedo accounts for all of the light scattered from a body at all wavelengths and all phase angles, it is a necessary quantity for determining how much energy a body absorbs.
This, in turn, is crucial for determining the equilibrium temperature of a body.
The planetary Bond Albedo is measured by satellites.
There are two types of reflection - the diffuse reflection and the specular reflection.
The specular reflection part in Bond Albedo is commonly considered so much insignificant, that the definition of Bond Albedo is limited to the diffusely reflected ratio.
Thus, for planets and moons with smooth surface, their STRONG SPECULAR REFLECTION is being neglected.
************
And it was the planet surface Lambertian reflectance considerations (or diffusely reflecting surface) the cause to wrongly estimate the planet specular reflection as a negligible value.
The-not reflected-portion = It is the what has left after reflection and dispersion of the incident solar flux
In the E-in versus E-out radiation balance, you always see on the left side of the equation:
π * r²
The total solar irradiance hitting a hemisphere of 2*π*r² is obtained by weighting the 1.362 W/m² by the square of the cosine of the incidence angle; that gives π*r².
–
–
Thus the total solar irradiance hitting a hemisphere is:
π*r²*So = π*r²*1.362 W/m²
–
-
The next very important step is to determine what has left of
“the total solar irradiance hitting a hemisphere”
for the planet radiation balance left side of the equation
E-in versus E-out
–
Because it is one thing the E-in and it is another thing
the
E-in- the-not reflected-portion of
“the total solar irradiance hitting a hemisphere” .
–
In other words- it is about of the -what has left after reflection and dispersion of the incident solar flux.
******
Planet Mars’ Te =210K and
Tsat =210K coincidence.
Planet Mars’ Te =210K and Tsat =210K coincidence
cannot be explained otherwise, but by aknowledging a theoretical mistake.
However, both (measured Tsat average) and (calculated Te uniform) depend heavily on the same two physical properties, namely:
S - the same solar flux (W/m²)
a - the same satellite measured average Albedo
As we have already demonstrated, Tsat > Te almost for all planets and moons.
So there should be a reason for that coincidence...
And the measured (Tsat) also necessarily depends on planet spin (N) and on planet surface specific heat (cp). And the calculated (Te) - does not.
Planet spin (N) and planet surface specific heat (cp) are being ignored in the theoretical Te aproach formula.
Planet Mars’ Te =210K, but it is a wrongly calculated planet (Te), planet Mars’ (Te) is calculated only on the basis of the two the surface major parameters, namely on planet Mars’ measured Albedo (a =0,250) and Solar flux at Mars' orbit distance from the sun.
Planet Mars exibits a strong specular reflection, which is (similarly as in Earth’s case) being simply neglected.
When planet Mars’ strong specular reflection is taken into consideration, planet Mars’ Te =210K is corrected to
Te.correct=174K.
Let's introduce to the Φ -Factor
It is very important!
Φ - is the dimensionless Solar Irradiation accepting factor - very important.
It is a realizing that a sphere's surface "absorbs" the incident solar irradiation not as a disk of the same diameter, but accordingly to its spherical shape.
For a smooth spherical surface
Φ = 0,47
What a smooth enough planet surface is for the incident solar light's specular reflection to occur?
Here it is an interesting insight we share with you !
We shine a light on a wall (from a fair way back).
Then we place a tennis ball in front of the wall, thus reducing the amount of light striking the wall.
Next to the ball we fix a disc of opaque material to the wall, with radius equal to the radius of the ball.
Ignoring minor effects due to non-parallel rays, which object blocks the most light from hitting the wall?
–
****
The tennis ball’s shadow on the wall is the same size as the disk’s shadow on the wall…
–
So they block the same exactly amount of light from hitting the wall!
–
–
Now, which one of the above described objects, reflects the most light, and which one absorbs the most light?
–
If the surfaces are made of the same material, and provided that reflection is isotropic, they each reflect the same total amount of light.
-
Near the “edge” of the earth (as seen from the sun) the surface flux of an incoming “sunbeam” reduced by exactly the same percentage as the area of that surface increases.
-
In our example we were comparing a disk to a sphere assuming all other parameters were constant.
****
What same material?
Also the material can be a smooth layer, or the material can form a rough surface...
What exactly the rough planet surface can be defined for - in order for the surface to be capable capturing some of the specular reflected solar energy?
What makes planet surface smooth, and what makes it rough?
Also, there should be a gradation of the planet surface smoothness, or, of the planet surface roughness.
And (it is demonstrated in this site) the smoothness is limited to some level, which doesn't make the more smooth surface to reflect more.
And, likewise, the roughness is limited to some level, beyond which the more rough planet surface does not absorb more solar radiative energy.
-
*****
Opponent:
"We need some logical explanations of Φ (hydrodynamic drag coefficient of a sphere is not an adequate explanation), why earth [and other celestial bodies] are smooth (they are not with respect to the wavelength of the interacting EMR)."
–
Answer:
When spheres in parallel fluid flow, the spheres’ surface gets in interaction with the fluid’s atoms and molecules, which also are very small, not small as a photon is, but very small too.
-
Those spheres in the fluid’s flow, no matter how well polished they are, on the atomic and molecular level they interact with fluid, their surfaces also are not smooth.
–
The surface is smooth or not, it has to do with the dimensions of the objects.
A planet as a whole can be a smooth surface planet, or it can not be a smooth surface planet.
Some planets and moons are considered smooth surface, and others are considered not.
-
******
A heavy cratered planet surface has a resemblance with a dence urban area, where high buildings form the deep-down streets-canjons. Solar rays are subjected to multiple reflections and absorptions there...
Heavy cratered planet surface reflects less and absorbs more of the incident solar radiative energy.
Consequently, when planet surface is a heavy cratered, everything else equals, the heavy cratered planet develops a warmer surface (a higher average surface temperature).
****
Earth also has glint.
****
The Earth is actually a smooth planet.
–
Yes, they are made from the same material.
But we should be very careful here, because the reflection of light is not an isotropic phenomenon.
The reflection of light is a complex phenomenon, in most cases it appears as a diffuse reflection, but diffuse reflection (it looks like), but it is not an isotropic reflection...
-
Actually reflection is not isotropic, reflection is resulting from the radiative flux’s interaction with matter.
Flux is a directional radiative energy. Reflection cannot be characterized as a 100% isotropic phenomenon.
–
***
The following question arises:
What a smooth enough planet surface is for the incident solar light's specular reflection to occur?
Φ factor explanation
The Φ - solar irradiation accepting factor - how it "works".
It is not a planet specular reflection coefficient itself. There is a need to focus on the Φ factor explanation. Φ factor emerges from the realization that a sphere reflects differently than a flat surface perpendicular to the Solar rays.
Φ – is the dimensionless Solar Irradiation accepting factor.
"Φ" is an important factor in the Planet Mean Surface Temperature Equation:
Tmean.planet = [ Φ (1-a) S (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴ (K) (3)
It is very important the understanding what is really going on with by planets the solar irradiation reflection. There is the specular reflection and there is the diffuse reflection. The planet's surface Albedo "a" accounts only for the planet's surface diffuse reflection.
........................................
The importance of the Solar Irradiation Accepting Factor Φ.
For smooth surface planets (like Earth) the Φ =0,47
So = 1362 W/m² - is the solar flux on the TOA (the top of atmosphere). It is also called the Solar Constant.
a = 0,306 - is the Earth's average surface Albedo.
Thus the incident on Earth solar energy not reflected from the planetary cross-section disk is:
1362 W/m² *Φ(1 - a) = 1362 W/m² *0,47(1 - 0,306) = 444 W/m²
This not reflected energy doesn’t get distributed over the hemisphere or over the sphere.
The not reflected portion of 444 W/m² is INTERACTING with planet’s surface matter on the very instant of incidence.
The TSI (Total Solar Irradiance) is related to the amount of energy reaching a flat earth disc area at one solar unit.
The Solar Irradiation Accepting Factor "Φ" makes a difference, out by possibly as much as 53 percent and hence all figures for amount of energy in, amount of visible light reflected, amount of IR energy emitted are very much different too.
-
and that means nearly everything we considered till now about the earth’s surface energy budget needs to be thrown out.
Nearly everything we had considered about on the earth’s surface energy budget needs to be thrown out.
........................................
In short, the Φ -Factor is not the planet specular reflection portion itself. The Φ -Factor is the Solar Irradiation Accepting Factor (in other words, Φ is the planet surface spherical shape and planet surface roughness coefficient).
............................
How to formally prove Φ -Factor's correctness in the
Ein = Eout formula.
-
Answer: The Energy in:
Ein = (1-a)S W/m²
used in the blackbody planet effective temperature Te is an empirical assertion, which is not based on any theoretical research, not to say, its correctness has not been demonstrated, quite the opposite…
–
The Energy in:
Ein = Φ(1-a)S W/m²
is based on measurements (the Drag Coefficient for smooth spheres in a parallel fluid flow Cd = 0,47), and it is demonstrated to be the correct one.
The Φ -Factor's importance is explained in every detail in next pages in this site.
Link:
https://www.cristos-vournas.com/445559911
***************************
The 4th root powers twice
The 4th root powers twice is an observed the Rotational Warming (N*cp) in sixteenth root power phenomenon when planet mean surface temperatures comparison ratios with the coefficients is compared.
Please visit the page “Earth/Mars 288K/210K”
The entire subthread there is devoted to the planets’ mean surface temperatures comparison. And every time for the compared planets’ the (N*cp) in sixteenth root is necessarily present.
................................................
Earth / Mars satellite measured mean surface temperatures 288 K and 210 K comparison.
It is a demonstration of the Planet Surface Rotational Warming Phenomenon!
These ( Tmean, R, N, cp and albedo ) planets' parameters are all satellites measured.
These planets' parameters are all observations.
Planet…....Earth.….Moon….Mars
Tsat.mean.288 K….220 K…210 K
R…...............1... AU..1 AU..1,525 AU
1/R²…..........1…........1….…0,430
N…..............1....1 /29,531..0,9747
cp................1.........0,19.......0,18
a..............0,306......0,11......0,250
1-a…........0,694……0,89…….0,75
(1-a)¹∕ ⁴…0,9127....0,9713…0,9306
coeff...........1...................0,72748
As we can see Earth and Mars have very close values for
(1-a)¹∕ ⁴ term;
For Earth (1-a)¹∕ ⁴ = 0,9127 and for Mars (1-a)¹∕ ⁴ = 0,9306.
Also Earth and Mars have very close N; for Earth N = 1 rotation /day, and for Mars N = 0,9747 rotation /day.
Earth and Mars both have the same Φ = 0,47 solar irradiation accepting factor.
Thus the comparison coefficient can be limited as follows:
Comparison coefficient calculation
[ (1/R²) (cp)¹∕ ⁴ ]¹∕ ⁴
Earth: Tsat.mean = 288 K
[ (1/R²)*(cp)¹∕ ⁴ ]¹∕ ⁴ =
= [ 1*(1)¹∕ ⁴ ] ¹∕ ⁴ = 1
Mars: Tsat.mean = 210 K
[ (1/R²)*(cp)¹∕ ⁴ ]¹∕ ⁴ =
= [ 0,430*(0,18)¹∕ ⁴ ] ¹∕ ⁴ = ( 0,430*0,65136 )¹∕ ⁴ =
= ( 0,2801 )¹∕ ⁴ = 0,72748
Let's compare
Earth coeff. / Mars coeff. =
= 1 /0,72748 = 1,3746
And
Tmean.earth /Tmean.mars =
= 288 K /210 K = 1,3714
.............................................
The results (1,3746) and (1,3714) are almost identical! .
Conclusion:
Everything is all right. It is a demonstration of the Planet Surface Rotational Warming Phenomenon!
And It is the confirmation that the planet surface specific heat "cp" should be considered in the (Tmean) planet mean surface temperature equation in the sixteenth root:
Tmean.planet = [ Φ (1-a) So (1/R²) (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴ (K) (3)
............................................
Earth / Europa (Jupiter's moon) satellite measured mean surface temperatures 288 K and 102 K comparison.
It is a demonstration of the Planet Surface Rotational Warming Phenomenon!
All the data below are satellite measurements.
All the data below are observations.
Planet….Earth….Europa
Tsat.mean 288 K….102 K
R…...........1 AU…5,2044 AU
1/R²………1…….0,0369
N………....1……1/3,5512 rot./day
a…………..0,306……0,63
(1-a)………0,694……0,37
coeff...0,9127...0,3158
Comparison coefficient calculation
[ (1-a) (1/R²) (N)¹∕ ⁴ ]¹∕ ⁴
Earth: Tsat.mean = 288 K
[ (1-a)*(1/R²)*(N)¹∕ ⁴ ]¹∕ ⁴ =
= ( 0,694 * 1 * 1 )¹∕ ⁴ = 0,9127
Europa: Tsat.mean = 102 K
[ (1-a)*(1/R²)*(N)¹∕ ⁴ ]¹∕ ⁴ =
= [ 0,37*0,0369*(1/3,5512)¹∕ ⁴ ] ¹∕ ⁴ = 0,3158
Let's compare
Earth coeff. /Europa coeff. =
= 0.9127 /0,3158 = 2,8902
And
Tmean.earth /Tmean.europa =
= 288 K /102 K = 2,8235
...............................................
The results (2,8902) and (2,8235) are almost identical!
Conclusion:
Everything is all right. It is a demonstration of the Planet Surface Rotational Warming Phenomenon!
Notice:
We could successfully compare Earth /Europa ( 288 K /102 K ) satellite measured mean surface temperatures because both Earth and Europa have two identical major features.
Φearth = 0,47 because Earth has a smooth surface and Φeuropa = 0,47 because Europa also has a smooth surface.
cp.earth = 1 cal/gr*°C, it is because Earth has a vast ocean. Generally speaking almost the whole Earth’s surface is wet. We can call Earth a Planet Ocean.
Europa is an ice-crust planet without atmosphere, Europa’s surface consists of water ice crust,
cp.europa = 1cal/gr*°C.
Conclusion:
Everything is all right. It is a demonstration of the Planet Surface Rotational Warming Phenomenon!
And It is a confirmation that the planet axial spin (rotations per day) "N" should be considered in the (Tmean) planet mean surface temperature equation in the sixteenth root:
Tmean.planet = [ Φ (1-a) So (1/R²) (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴ (K) (3)
******************
Io vs Europa (Tsat 110K vs Tsat 102 K) comparison
Why Io is warmer than Europa?
"It takes Io about 42.5 hours (1.77 days) to complete one orbit around Jupiter (fast enough for its motion to be observed over a single night of observation). Io is in a 2:1 mean-motion orbital resonance with Europa "
-
Io is in a 2:1 mean-motion orbital resonance with Europa,
also Io average surface specific heat is cp = 0,145 cal/gr*oC, whereas Europa is cp = 1 cal/gr*oC.
Thus, Io rotates twice as fast, but Io has
1/0,145 = 6,89 times smaller average surface cp.
Both Io and Europa have the same Albedo a = 0,63
Io has warming factor =(β*N*cp)¹∕ ⁴ = 1,8647
Io has average surface temperature measured by satellite
Tsat.io = 110K
Europa has Tsat.europa = 102K
And Europa has warming factor =(β*N*cp)¹∕ ⁴ = 2,5494
But also Europa is characterized as the smoothest object in the entire solar system.
So Φeuropa = 0,47 vs Φio = 1.
Let's compare:
[(1,8647 /2,5494) /0,47 ]¹∕ ⁴ = 1,116
110K /102K = 1,07
the 1,116 and 1,07 are very close ~ 3% difference,
which means the comparison is performed correctly.
And that is why Io is warmer than Europa
(Tsat.io = 110K vs Tsat.europa = 102K)
-
............................................
More Planets and Moons the satellite measured average surface temperatures comparison
Links:
Jupiter/Saturn/Neptune 165K /134K /72K
The rightness of the Rotational Warming Phenomenon is many times demonstrated and, also, it has been theoretically explained by the physics first principles.
Planet Surface Rotational Warming Phenomenon
The faster rotation - the warmer the planet!
The first steps
At the very first look at the data table we distinguish the following:
Planet..Tsat.mean..Rotations..Tmin..Tmax
...........measured...per day......................
Mercury..340 K.....1/176....100 K...700 K
Earth.....288 K........1.............................
Moon....220 Κ.....1/29,5.....100 K...390 K
Mars.....210 K....0,9747.....130 K...308 K
The Earth's and Mars' by satellites temperatures measurements, in relation to the incident solar irradiation intensity, appear to be higher,
and it happens because of Earth's and Mars' faster rotation.
I should say here that I believe in NASA satellites temperatures measurements. None of my discoveries would be possible without NASA satellites very precise planet temperatures measurements.
It is the "magic" of the planet's spin. When it is understood, it becomes science.
The closest to the sun planet Mercury receives 15,47 times stronger solar irradiation intensity than the planet Mars does.
However on the Mercury's dark side Tmin.mercury = 100 K, when on the Mars' dark side Tmin.mars = 130 K.
These are observations, these are the by satellite the planet surfaces temperatures measurements.
And they cannot be explained otherwise but by the planet Mars' 171,5 times faster rotation than planet Mercury's spin.
..........................................
Let's study the table of data above.
Interesting, very interesting what we see there
-
Earth and Moon are at the same distance from the Sun
R = 1 AU.
Earth and Mars have almost the same axial spin
N = 1rotation /day.
Moon and Mars have almost the same satellite measured average temperatures
220 K and 210 K.
Mercury and Moon have the same minimum temperature
100 K.
Mars' minimum temperature is 130 K, which is much higher than for the closer to the Sun Mercury's and Moon's minimum temperature 100 K.
And the faster rotating Earth and Mars appear to be relatively warmer planets.
..................................
Two planets with the same mean surface temperature may emit, on the average surface area, may emit dramatically different amounts of IR outgoing EM energy.
Moon's average surface temperature is Tmoon = 220 K
Mars' average surface temperature is Tmars = 210 K
Moon's average surface Albedo a =0,11
Mars' average surface Albedo a =0,25
It can be demonstrated that for the same Albedo Mars and Moon would have had the same average surface temperature.
The solar flux on Moon is So =1361W/m²
The solar flux on Mars is S =586W/m²
It is obvious, that for the same average surface temperature, the emitted amounts of energy from Moon, on the average surface area, are dramatically higher than the emitted amounts of energy from Mars.
..................................
To continue with the solar system's coincidences, which would be very useful in the further research, there is another very interesting observation shouldn't be neglected:
I have the gaseous planets at 1 bar level the satellite measured temperatures comparison in relation to the gaseous planets’ rates of rotation.
Gaseous planets (Jupiter, Saturn, Uranus, Neptune) have, between them, similar atmospheric gases content.
The more close the content is, the better the satellite measured temperatures relate in accordance to the Rotational Warming Phenomenon.
Link:
https://www.cristos-vournas.com/445559910
*******************
There has to be a PROCESS.
Somehow, someway a transformation has to be generated to affect the planet’s surface temperature.
You can’t just say RADIATIVE energy get converted into Heat. It’s more likely it stays Radiative energy.
There has to be a PROCESS.
...........................................
Planets and moons do everything differently.
Βy DEFINITION, the planet theoretical effective radiative temperature’s formula doesn’t consider planet rotating.
The formula is for planet with uniform surface temperature, and it is for planet with uniform surface irradiance.
Te = [ (1-a) S /4σ ]¹∕ ⁴ (1)
The Te cannot be some kind of a theoretical limitation for planets and moons without-atmosphere the mean surface temperatures not to exceed their theoretical Te calculated temperature.
Planets and moons do not have uniform surface temperature; and they do not have uniform surface irradiance either. And planets and moons do ROTATE.
Consequently, the
Te = [ (1-a) S /4σ ]¹∕ ⁴ (1)
is not capable to describe the real planets’ and moons’ the mean surface temperatures.
------------------------------------
Planet mean surface temperature cannot be associated with any kind of BB profile spectrum.
The BB (black body) profile spectrum is associated with a single BB emitting temperature.
A planet doesn’t have a uniform surface temperature. The planet’s mean surface temperature doesn’t have a BB profile spectrum, because planet doesn’t emit at mean surface temperature…
Every spot on the planet’s surface at every given instant has a different emitting temperature…
Every spot at that given instant emits with its own spectrum profile…
A planet’s mean surface temperature’s BB profile spectrum (theoretically expected) cannot be considered as the planet’s mean BB profile spectrum.
Planet mean surface temperature cannot be associated with any kind of BB profile spectrum.
————–
Two planets with the same mean surface temperature may emit, on the average surface area, may emit dramatically different amounts of IR outgoing EM energy.
****************
Also, we are explaining about the actual reasons of Global Warming at the bottom side of this page.
Earthrise, taken in 1968 Dec 24 by William Anders, an astronaut on board Apollo 8
Moon and Earth - so close to each other - and so much different...
We may conclude that for a faster rotating planet there is the phenomenon of its warmer surface...
The Planet Surface Rotational Warming Phenomenon
The Planet Surface Rotational Warming Phenomenon
I’ll try here in few simple sentences explain the very essence of how the Planet Surface Rotational Warming Phenomenon occurs.
A planet surface doesn't absorb solar energy first, gets warmed and only then emits IR EM energy.
No, a planet surface emits IR EM energy at the very instant solar flux hits the matter.
Lets consider two identical planets F and S at the same distance from the sun.
Let’s assume the planet F spins on its axis Faster, and the planet S spins on its axis Slower.
Both planets F and S get the same intensity solar flux on their sunlit hemispheres. Consequently both planets receive the same exactly amount of solar radiative energy.
The slower rotating planet’s S sunlit hemisphere surface gets warmed at higher temperatures than the faster rotating planet’s F sunlit hemisphere.
The surfaces emit at σT⁴ intensity – it is the Stefan-Boltzmann emission law.
Thus the planet S emits more intensively from the sunlit side than the planet F.
There is more energy left for the planet F to accumulate then.
That is what makes the faster rotating planet F on the average a warmer planet.
That is how the Planet Surface Rotational Warming Phenomenon occurs.
And it states:
Planets’ (without atmosphere, or with a thin atmosphere) the mean surface temperatures relate (everything else equals) according to their (N*cp) products’ sixteenth root.
......................................................................................................
Here it is what I have also to say.
1). The faster rotating planet has a less differentiated surface temperatures distribution. Thus, for the same amount of solar energy transformed into HEAT and accumulated in inner layers, the faster rotating planet has a higher average surface temperature.
2). The not reflected portion of the incident SW EM energy is NOT ENTIRELY transformed into HEAT.
3). In addition, the faster rotating planet is able to transform into HEAT and accumulate in inner layers LARGER amounts of the incident on surface solar energy, than a slow rotating planet.
Important Notice:
Rotational Warming Phenomenon states about the (N*cp) products' sixteenth root, not only the planetary rate of rotation (N) is involved, but also the planet average surface SPECIFIC HEAT (cp)!
...........................................................
Every spot on planet surface experiences its peak hot and cold temperature. The less are those differences, the higher is the average surface temperature
for the same not reflected portion of the incident solar flux.
-
The (N*cp)^1/16 is the way the planet average surface temperature “responds” to that.
The faster the rotation, the less time every spot is exposed to the solar flux’ EM radiative energy, the less the skin surface layer’s INDUCED temperature is.
The more atoms (higher surface cp) are getting exposed (INTERACTED) on the skin layer to the solar flux’ EM radiative energy, the less the skin surface layer’s INDUCED temperature is.
The Planet Mean Surface Temperature New equation
The planet mean surface temperature New equation is written for planets and moons WITHOUT atmosphere.
The results of calculations are remarkably exact!
When applied to Earth (Without Atmosphere) the New equation calculates Earth’s mean surface temperature very much close to the 288K.
Earth is a planet, like any other planet we know in solar system.
Neither Stefan, no Boltzmann said anything about planets being ideal blackbodies.
-
Hansen compared the theorized planet UNIFORM surface temperature
(the Earth's EFFECTIVE temperature Te =255K) with the Satellite Measured Earth's average surface temperature Tmean =288K.
-
Those temperatures, the planet UNIFORM surface temperature, and the planet AVERAGE surface temperature are different Physical Terms.
-
By Hansen's idealized Formula, when considering a planet AVERAGE surface temperature, it cannot mathematically exceed the same planet idealized UNIFORM surface temperature.
-
Thus, Hansen resumed, the satellite measured Earth's AVERAGE surface temperature Tmean =288K,
is at least +33°C warmer than the theorized Earth's UNIFORM temperature 255K.
-
The +33°C had to be somehow explained. So it was attributed to the not existent (the very insignificant) the Earth's atmosphere Greenhouse Effect.
-
Also, it was asserted, the above very confusing and very mistaken conclusion (the Earth having at least +33°C (Greenhouse Effect), it was asserted the above was in full accordance with the 1st Law of Thermodynamics (1LOT).
-
They ignored the INITIAL Fundamental Mistake, they had made.
The Stefan-Boltzmann emission law cannot be used wise-versa. One cannot determine a surface temperature by simply measuring the radiative flux's intensity falling upon it.
-
***
-
What I did in my research was to compare the satellite measured planetary temperatures for every known planet and moon in solar system, Earth included.
-
When I wrote the New equation, yes, I hopped for coming up to something, but the results were successful beyond any expectations.
Here it is the planet 1LOT energy balance analysis
related New equation:
Tmean = [ Φ (1-a) S (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴ (K) (3)
The New equation is based both, on precise radiative
“energy in = Φ (1-a) S” estimation and
on the “Planet Rotational Warming Phenomenon“.
We are capable now for the THEORETICAL ESTIMATION of the planetary mean surface temperatures.
And, now, it should be considered proven - there is not any Greenhouse Warming Effect on the Earth's surface temperature!
............................
Also, the Incomplete Equation of the Planet Blackbody Effective Temperature,
Link from Wikipedia:
the Planet Effective Temperature Te
https://en.wikipedia.org/wiki/Planetary_equilibrium_temperature
Another Link:
Lecture 2: Effective temperature of the Earth
the Incomplete Equation of the Planet Blackbody Effective Temperature
Te = [ (1-a) S / 4 σ ]¹∕ ⁴ (K) (1)
should be abandoned, because it is very much wrong!
==========
The false "RADIATIVE equilibrium" CONCEPT
Also, I should note that the average solar flux is a pure mathematical abstraction.
Solar flux does not average over the planet surface in the real world.
When we "imagine" solar flux averaging on the entire planet surface it is like having (the false RADIATIVE equilibrium CONCEPT), it is like having the actual planet being enclosed in an imaginary sphere, which sphere is emitting towards the planet surface a constant flux of 240 W/m^2.
But it is not what happens in the real world!
.................................................
Planet is not a uniformly heated body.
Planet is a solar irradiated from one side spherical object.
The irradiated side is not uniformly irradiated.
The planet’s opposite side is in total darkness.
Thus, a planet is not a blackbody!
……………..
I use the Stefan-Boltzmann emission law in the right way.
The planet black body formula averages solar flux over the entire planet area in form of HEAT.
The New equation doesn’t average solar flux over the entire planet area in form of HEAT.
For the New equation the outgoing EM is a result of the incident on the planet surface solar energy INTERACTION process with the matter.
Black body by definition transforms its calorimetric HEAT into its absolute temperature T fourth power EM emission intensity.
On the other hand, planet doesn’t emit EM energy supplied by a calorimetric source. The planet’s surface temperature is INDUCED by the incident on the planet solar EM flux.
Only a small portion of the incident solar EM energy is transformed into HEAT.
The vast majority of the incident solar energy is IR emitted at the same very moment of incidence and interaction with matter.
This EM energy induces the planet surface temperature without being accumulated in the inner layers.
It is entirely different physics when compared with the “quiet” blackbody calorimetric HEAT black body emission phenomenon.
.................................................
Earth “absorbs” 28% less solar energy than Moon (Albedo Earth a =0,306; Albedo Moon a =0,11).
And yet,
The measured Earth’s average surface temperature Tearth=288K.
The measured Moon’s average surface temperature Tmoon=220K.
Mars orbits sun at R = 1,524 AU.
(1/R²) = (1/1,524²) = 1/2,32
Mars has 2,32 times less solar irradiation intensity than Earth has.
So the solar flux at Mars’ orbit is 2,32 times weaker than on Moon too.
And yet
The measured Mars’ average surface temperature Tmars=210K.
Which is close to the measured Moon’s average surface temperature Tmoon=220K.
Mars' Albedo a =0,250; Moon's Albedo a =0,11.
It can be shown, that for the same Albedo Mars and Moon would have the same average surface temperature.
……………..
Let's see now:
Tmoon =220K
Tearth =288K (for Earth having 28% less than Moon solar energy "absorbed")
Tmars =210K (for Mars having 2,32 times less than Moon solar energy "absorbed")
These obvious discrepancies can be explained only by the Earth's and by the Mars' much faster than Moon's rates of rotation.
These obvious discrepancies can be explained only by the Planet Surface Rotational Warming Phenomenon.
Opponent:
There has to be a tested hypotheses. Otherwise its not reliable.
No quality assurance? No replication? No checking? No testing?
Not even peer review?
Its unreliable!
Answer:
"There has to be a tested hypotheses. Otherwise its not reliable.”
Or , as Richard Feynman said “It doesn’t matter how beautiful your theory is, it doesn’t matter how smart you are. If it doesn’t agree with experiment, it’s wrong.”
-
Well, the method we use in present research is "the planets surface the satellite measured temperatures comparison".
-
We do everything correctly. Haven't we demonstrated reproducible experiments?
-
When we do the same calculations on every planet and on every moon in solar system and the results are so very much close to those measured by satellites... those calculations are adequate to the very much convincing reproducible experiments!
The planet temperature varies with planet rotation. It is an observation.
There is no need in an experiment with a rotating sphere in a vacuum exposed to sunlight…
That is why no experiment is needed.
We have here the "Planet Surface Rotational Warming Phenomenon" observed.
The planet temperature varies with planet rotation. It is an observation.
There is no need in an experiment with a rotating sphere in a vacuum exposed to sunlight…
Here is the clear relation example:
Let's illustrate on the planet's effective temperature old equation
Te = [ (1-a) S /4σ ]¹∕ ⁴ (K)
Mars is irradiated 2,32 times weaker than Moon, but Mars rotates 28,783 times faster.
And… for the same albedo, Mars and Moon would have the same satellite measured mean temperatures.
For Moon Tmean = 220K; Moon’s Albedo a=0,11
For Mars Tmean= 210K; Mars’ Albedo a=0,25
Let’s do a simple calculation:
The rotation difference’s fourth root is
(28,783)¹∕ ⁴ = 2,3162
Now, please compare these two numbers:
2,32 and 2,3162
They are very-very much close, they are almost identical!
That is why no experiment is needed.
In this example we have demonstrated that the Mars' solar irradiation intensity deficit being 2,32 times less is compensated by Mars' 28,783 times higher rate of rotation in fourth root
(28,783)¹∕ ⁴ = 2,3162
We have here the "Planet Surface Rotational Warming Phenomenon" observed.
*****
We shall continue in the next pages.
And
———-
The Solar Radiation Input.
Every spot on planet surface experiences its peak hot and cold temperature. The less are those differences, the higher is the average surface temperature for the same not reflected portion of the incident solar flux.
The (N*cp)^1/16 is the way the planet average surface temperature “responds” to that.
The faster the rotation, the less time every spot is exposed to the solar flux’ EM radiative energy, the less the skin surface layer’s INDUCED temperature is.
The more atoms (higher surface cp) are getting exposed (INTERACTED) on the skin layer to the solar flux’ EM radiative energy, the less the skin surface layer’s INDUCED temperature is.
The Planet Surface Rotational Warming Phenomenon:
It is well known that when a planet rotates faster its daytime maximum temperature lessens and the night time minimum temperature rises.
But there is something else very interesting happens.
When a planet rotates faster it is a warmer planet.
————
The physics notion: solar radiation input.
It is important to differentiate between the “not reflected portion of the incident solar flux ” and the “solar radiation input.”
—
Well, the “not reflected portion of the incident solar flux” is not even ” with solar radiation input.
—
The “solar radiation input” is only a part of the “not reflected portion of the incident solar flux”, it is only a part of “Energy in”.
—–
The “solar radiation input” is only the energy accumulated in inner layers. It is not the entire “Energy in”.
Energy in = energy out
Energy in = πr²Φ*S*(1-a) (W) is the “not reflected portion of the incident solar flux”
Jemit = 4πr²σΤmean⁴ /(β*N*cp)¹∕ ⁴ (W) is the energy out
Planet Energy Budget:
Jnot.reflected = Jemit πr²Φ*S*(1-a) = 4πr²σTmean⁴ /(β*N*cp)¹∕ ⁴ (W)
Solving for Tmean we obtain the PLANET MEAN SURFACE TEMPERATURE EQUATION:
Tmean.planet = [ Φ (1-a) S (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴ (K)
——–
When hitting matter the solar EM energy INDUCES surface skin layer’s temperature.
The higher this temperature, the more the instantly emitted IR EM radiative energy out, and the lesser the solar radiation input.
And
The lesser this temperature, the less the instantly emitted IR EM radiative energy out, and the more the solar radiation input.
———
When planet rotates faster it has for a constant
“Energy in = πr²Φ*S*(1-a) (W)” a larger solar energy input.
—
The entire
“Energy out” = Jemit = 4πr²σΤmean⁴ /(β*N*cp)¹∕ ⁴ (W) consists from both “the instantly emitted IR EM radiative energy out” plus the solar radiation input.
———-
When rotating faster, planet surface has more “solar radiation input” to get rid of, thus the Tmean gets “balanced” at higher level.
So, the higher the (N*cp)¹∕ ⁴ is, the higher should be the Tmean⁴.
That is why it is so powerful the Planet Surface Rotational Warming Phenomenon.
———-
------
“The amount of solar radiation received does not change because a planet spins faster. It has to radiate away what it receives.”
It is a basic principle! The difference is that, when interacting with matter, the “not reflected portion of solar flux” is not getting inside the skin layer in its entirety.
A very considerable part of it gets IR emitted on the very instant the solar EM energy hits the surface.
This part of energy goes immediately out, it INDUCES the surface temperature on the instant of incidence, and that temperature is the temperature of that spot at that particular instant.
———-
And, there is another part, the “SOLAR RADIATION INPUT” which is on that instant is accumulated in the inner layer. And this part is radiated away later…
———-
Planet always radiates away what it receives.
Planet with a higher (N*cp) product has this second part, the “SOLAR RADIATION INPUT” larger, and the on the instant of incidence IR emitted amount of solar EM energy is smaller, because a planet with a higher (N*cp) product INDUCES a lower on the spot of incidence the skin layer’s temperature.
———-
We shall call from now on as “the “SOLAR RADIATION INPUT” the amount of energy the Solar Flux – Planet Surface INTERACTION process manages to PUT IN the planet inner layer.
"That’s right, and EM energy contains no heat. Therefore no heat can be transferred, as heat, from the Sun to Planet.
Old heat -> EM emission -> EM energy/matter interaction -> New heat".
********
-
What GREENHOUSE EFFECT?
The actual greenhouse (agricultural, with transparent to solar rays glass windows, or with transparent to solar rays membrane...) gets warmed because the solar SW EM energy comes through and warms the inside walls and the inside floor.
The emitted by the walls and the floor IR EM energy is only absorbed or reflected back from the glass windows or the membrane, because they are not transparent to the IR EM energy. The phenomenon causes the rise in temperature of the inside of the greenhouse's walls and floor.
The phenomenon is called greenhouse effect.
-
Now, when a greenhouse is hermetically closed, it resemblances the case of the indoors air. The indoors air is in thermal equilibrium with the surrounding walls, and, therefore, the indoors thermometer indicates the same exactly temperature for the inside of the walls and for the inclosed air.
-
If we let an outside cold air indoors (into a room, or into a greenhouse) the air temperature will remain cold for a while, untill it is adjusted to the inside temperature.
-
The air inside of a greenhoouse, or inside of a room, gets warmed by conduction from the inside of walls.
The lnside air is not getting warmed by EM radiation, because, as it is known, air is for the most of its content is transparent to both, SW and IR EM energy.
-
The same, the greenhouse effect phenomenon, the same is taking place in the case of planets, which planets have some amounts of greenhouse gasses in their atmospheric content.
-
Greenhouse gasses in atmosphere are transparent to the incoming SW EM energy, but the greenhouse gasses are capable to reflect back to the planet surface some amount of emitted by the surface IR EM energy.
-
As a result, the temperature of the planet surface gets higher, than it would otherwise be in the absense of those greenhouse gasses.
-
-
Earth's atmosphere is very thin. The greenhouse gasses content in Earth's atmosphere are considered as trace gasses.
-
So, we have in Earth's already thin atmosphere some trace greenhouse gasses...
-
-
It was those very obvious circumstances that lead us to the only conclusion - there cannot be +33 oC greenhouse effect from Earth's atmosphere.
-
I estimate the Earth's atmosphere TOTAL GREENHOUSE EFFECT about some +0,4 oC, which is almost two orders of magnitude less than +33 oC.
-
“The Tmean equation gives a decent approximation for small range of rotation rates.”
Yes, that is what it does. The equation is limited in a small range of rotation rates.
–
Let’s see what we have:
We have an equation which theoretically calculates for all planets and moons (without-atmosphere, or with a thin atmosphere), the average surface temperatures (Tmean), and the equation's results are very much close to those measured by satellites.
–
Also we have the Rotational Warming Phenomenon, and all planets and moons, (including the gaseous giants Jupiter, Saturn, Uranus and Neptune), all of them are subjected to that Rotational Warming Phenomenon, which is demonstrated in the site, and which could be considered as a confirmed observation.
–
And, for all planets and moons, (without-atmosphere, or with a thin atmosphere), the theoretical effective temperatures (Te), when calculated, are always lower than the satellite measured mean surface temperatures (Tmean).
The only exception is the very slow rotating Mercury.
–
And there is the planet Mars with the satellite measured Tmean =210 K, the Mars having dramatically less than our Moon (because of the distance from the sun) having dramatically less solar irradiation, vs Moon’s the satellite measured Tmean =220 K.
The Mars' and Moon's Tmean proximity can only be explained by the Mars’ very fast, compared to Moon rate of rotation.
-
*******
It is the same model, but the settled science mistakenly uses – the comparison of the planets' without-atmosphere the theoretical uniform surface effective temperatures Te, (which is a mathematical abstraction), the settled science uses Te for the comparison with the actual satellite measured average surface temperatures Tmean, for every planet and moon, Earth included.
Scientists base their ideas on evidence. What they can observe, measure and test. They change their ideas to match what is observed. This is science in a nutshell. Follow the evidence!
-
We can’t talk, evidence based, about the impact of CO2 on the climate system of the Earth.
-
We have already established that Planet Surface Rotational Warming Phenomenon is what actually accounts for the full difference between Earth and Lunar mean global surface temperature.
And yes, it is our reality – a world without GHGs.
The very small amounts of GHGs in our thin atmosphere do not make it a world with GHGs.
The Conclusions
Conclusions:
1). The planet mean surface temperature equation
Tmean = [ Φ (1-a) S (β*N*cp)¹∕ ⁴ /4σ ]¹∕ ⁴
produces remarkable results. The theoretically calculated planets temperatures (Tmean) are almost identical with the measured by satellites (Tsat.mean).
Planet…......Te......Te.correct....Tmean….Tsat.mean
Mercury....440 K......364 K........325,83 K…..340 K
Earth……...255 K......210 K........287,74 K…..288 K
Moon……..270,4 K....224 K........223,35 Κ…..220 Κ
Mars……….210 K......174 K........213,11 K…..210 K
2). The 288 K – 255 K = 33 oC difference does not exist in the real world.
There are only traces of greenhouse gasses. The Earth’s atmosphere is very thin.
There is not any measurable Greenhouse Gasses Warming effect on the Earth’s surface.
There is NO +33°C greenhouse enhancement on the Earth's mean surface temperature.
Both the calculated by equation and the satellite measured Earth's mean surface temperatures are almost identical:
Tmean.earth = 287,74K = 288 K.
........................
The planet mean surface temperature New equation is written for planets and moons WITHOUT atmosphere.
The results of calculations are remarkably exact!
When applied to Earth (Without Atmosphere) the New equation calculates Earth's mean surface temperature as 287,74K, which is very much close to the satellite measured 288K.
It happens so because Earth's atmosphere is very thin and, therefore, doesn't have any essential greenhouse effect on the Earth's average surface temperature.
When it is acknowledged Earth’s atmosphere is very thin – it will become obvious, Earth doesn’t have any significant greenhouse warming effect.
-
***
Earth's atmosphere greenhouse effect is only some
+0,4 oC.
-
Earth's atmosphere greenhouse effect was very mistakenly estimated as being
+33 oC
which is very much wrong !
-
The +1,5 oC rise is due to orbital forcing, the additional CO2 cannot be considered as warming Earth's surface by +1,5 oC, because the entire atmosphere warms surface only by some
+0,4 oC !
-
*****
Thus, the current observed GLOBAL WARMING cannot be attributed to the fossil fuels burning.
-
*****
Earth's average surface temperature is 68°C higher than Moon's average surface temperature.
Also Earth, because of a higher than Moon Albedo, Earth receives 28% less solar radiative energy than Moon.
-
And yet, Earth's average surface temperature is 68°C higher than Moon's average surface temperature.
-
Since Earth and Moon are at the same distance from the sun, but Earth's average surface temperature is 68°C higher than Moon's average surface temperature... there is only one explanation left:
It is the planet surface ROTATIONAL WARMING PHENOMENON !
-
2). There is not any detectable (spacecraft measured) the solar system moons' (Io, Europa and etc.) tidal warming.
-
3). Gaseous Giants (Jupiter, Saturn, Uranus and Neptune) do not have any detectable (spacecraft measured) inner source of energy.
-
We have to answer these two questions:
1. Why Earth’s atmosphere doesn’t affect the Global Warming?
It is proven now by the Planet's Mean Surface Temperature Equation calculations. There aren’t any atmospheric factors in the Equation.
Nevertheless the Equation produces very reasonable results:
Tmean.earth = 287,74 K,
calculated by the Equation, which is the same as the
Tsat.mean.earth = 288 K,
measured by satellites.
Tmean.moon = 223,35 K, calculated by the Equation, which is almost identical with the
Tsat.mean.moon = 220 K, measured by satellites.
2. What causes the Global Warming then?
The Global Warming is happening due to the orbital forcing.
And… what keeps Earth warm at
Tmean.earth = 288 K,
when Moon is at
Tmean.moon = 220 K?
Why Moon is on average 68 oC colder? It is very cold at night there and it is very hot during the day…
Earth is warmer because Earth rotates faster and because Earth’s surface is covered with water.
And Moon also emits from its very hot daytime surface hard.
Does the Earth’s atmosphere act as a blanket that warms Earth’s surface?
No, it does not.
What else the very hot surface does but to emit hard, according to the Stefan-Boltzmann emission Law.
The very hot surface emits in fourth power of its very high absolute temperature.
Jemit ~ T⁴
A warm object in space loses heat via emission. The hotter is the object, the faster it loses heat.
Moon gets baked hard during its the 14,75 earth diurnal cycles long lunar day.
So there is not much energy left to emit during the 14,75 earth diurnal cycles long lunar night.
And it becomes very cold on the Moon at night. It is in our Earth's immediate neighborhood happens.
That has almost nothing to do with atmosphere, and everything to do with rotation speed.
.......................................................
The planet Earth's and the planet Mars' faster rotation creates the necessary level of the "solar irradiation - planet surface" interaction phenomenon...
which results in the day-time much lower surface temperatures and, consequently, in much lower day-time surface infrared radiation emissions
and which results in higher planet surface 24-hours average temperatures.
The planet Earth’s and the planet Mars’ faster rotation is what creates the necessary interaction for the incident on the planets' surfaces solar energy the much more efficient accumulation.
The best news science could give us is that we aren’t about to be destroyed by calamitous climate change, that there’s no need to panic, there’s still plenty of time to solve, to adapt, to reconcile ourselves to a different future, and to stop worrying.
Science is all about confirming theories.
-
See, science can be either confirming or denying conjectures, depending on the conjectures.
It is all in the details...
Summary:
A new universal equation for calculating a planet's mean surface temperature is developed here, to provide better estimates than the simple "blackbody" equation which was based on simplifying assumptions.
Recognizing that a real planet does not match the assumptions for an idealized blackbody, Vournas developed an expanded equation with four additional terms to better represent a planet's actual conditions, particularly considering planet axial rotation.
The derivation of the new equation from the planet energy balance is shown below, followed by a description for each of the four new terms in the new equation, including rotation (N), specific heat capacity (cp), solar light reflection and dispersion (Φ, a), and a new universal constant (β) determined empirically.
This new Vournas equation results are compared for twenty (20) solar system bodies (planets and moons), with the equation's calculated temperature closely matching the data, the NASA satellite measured temperature.
***
******
**********
The Global average surface atmospheric greenhouse effect +33°C PARADOX
I also tried to answer that question:
“If the Global average surface atmospheric greenhouse effect is +33°C, then what approximately is the atmospheric greenhouse effect at the different latitudes, like:
1). Kenya
2). Egypt
3). Greece
4). Czechia
5). Sweden
6). North Pole
Notice, the higher latitudes represent smaller areas on the Globe.”
-
But I couldn’t answer that question, because there is not such an answer.
-
***
******
If there is +33°C atmospheric global average surface greenhouse warming effect, it should be stronger at places with higher solar irradiance, because the core issue in atmospheric global average surface greenhouse warming effect is the surface LW emission atmospheric feedback.
–
If there is +33°C atmospheric global average surface greenhouse warming effect, it should be the strongest at equatorial zone, because at equatorial zone the solar irradiance is the strongest.
–
We face a paradox here:
We should have assumed, in the case of Planet Earth without-atmosphere, at Earth’s equatorial zone the far below zero °C the surface temperatures…
***
******
**********
What is the atmospheric greenhouse warming effect on the Earth’s surface then?
-
It depends on what conditions are assigned to the mythical earth without atmosphere, depending mostly on choice of albedo.
-
Of course...
-
We need, though, to correctly estimate what would be the Earth's greenhouse gases warming effect, without- greenhouse gases.
-
Also, we have the notion of the planet effective temperature, which refers to a planetary surface without atmosphere, but with the actual existing Albedo.
-
Our purpose is to evaluate the estimated greenhouse effect vs the observed rise in global temperature.
-
If the Global atmospheric greenhouse effect is small ~ 0,4°C, then the temperature rise of 1,5°C from predindustrial period 1850 is not due to fossil fuels (mostly coal, oil and natural gas) burning.
-
If the Global atmospheric greenhouse effect is higher than the temperature rise of 1,5°C, then we need to know how much higher it is.
-
***
******
**********
The accepted research on Climate Global Warming all it leads to is a confussing cloud of inconsistencies, of discrepancies, and of uncertainties...
The research always comes to the dead end.
It is time to get back, and to start anew from the science's the very basic beginnings...
The S-B emission law cannot be applied neither to the planet solar lit side, nor to the planet darkside.
The Stefan-Boltzmann emission law is about the blackbody emission intensity of the hot bodies.
Hot bodies are the previously warmed bodies, or bodies having their own inner sourses of thermal energy.
Planets and moons surfaces' are very much insulated from the primordial heat the inner cores possesed.
Thus, planets and moons surfaces' temperatures do not rely on the inner sources of thermal energy.
Planets or moons are used to be confused with the hot bodies in the S-B emission sense, and it lead to the mistaken assertion:
“Nothing, other than the absorbed radiation is what warms the matter to some (local) temperature, which, along with the matter properties, determines the Planck spectrum and S-B flux of the outgoing thermal radiation.”
A New, a CORRECT ASSERTION should be made:
"When incident on planets and moons solar flux (the solar EM energy), the solar flux interacts with surface's matter, because the EM energy is not HEAT ITSELF!"
–
Ok!
_
Well, the planet’s dark side cools by emitting to space IR radiation. The dark side’s surface heat is the energy source of that IR EM energy emission.
There are not enough thermal energy (heat) at darkside terrestrial temperatures to support the S-B equation emission demands for the darkside respective surface temperatures.
Thus, the outgoing IR EM energy flux from the planet darkside is much-much weaker than what S-B equation predicts for those local temperatures.
–
On the planet’s solar lit side an interaction of the incident EM energy with surface’s matter occurs.
Part of the incident SW EM energy gets reflected (diffusely and specularly).
Another SW part gets instantly transformed into outgoing IR EM energy, and gets out to space.
When SW EM energy gets transformed into IR EM energy, the transformation is not a perfect process, there are always some inevitable energy losses, which dissipate as heat in the interacting surface’s matter and gets absorbed in the matter’s inner layers.
–
The S-B emission law cannot be applied neither to the planet solar lit side, nor to the planet darkside.
-
**********
************
S-B never works in real material world. It only works for imaginary black bodies with perfect spectral emission curves. That is why the term
Surface Emissivity (ε) was invented.
-
the S-B equation
Jemit = σT⁴ W/m² had for different materials, and for variations of temperature to be added
with Surface Emissivity (ε), which is an empirical for every application value.
and, therefore, the S-B equation was re-written as:
Jemit = ε*σT⁴ W/m²
The universality of S-B constant (σ) has been transformed into:
(ε*σ) coupled term.
Therefore,
a planet doesn't emit IR according to S-B emission law.
What it is believed is that the Stefan-Boltzmann blackbody curve is merely a benchmark from which to launch a research project from,
then its a multi-faceted job to determine emissivity at every spectral line
and then do the research experiments that will determine how a particular frequency will result in an equilibrated temperature
and what that temperature is.
***********
********
*****
So when things go wrong, we see how we can learn. It's all an opportunity for improvement.
Ok
There is always valid: For planets and moons without-atmosphere, or with a thin atmosphere (Earth included)
“…the mean surface temperatures RELATE (everything else equals) as their (N*cp) products’ SIXTEENTH ROOT.”
From Wikipedia
https://en.wikipedia.org/wiki/Stefan%E2%80%93Boltzmann_law
” In the more general (and realistic) case, the spectral emissivity depends on wavelength. The total emissivity, as applicable to the Stefan–Boltzmann law, may be calculated as a weighted average of the spectral emissivity, with the blackbody emission spectrum serving as the weighting function. It follows that if the spectral emissivity depends on wavelength then the total emissivity depends on the temperature.”
"It follows that if the spectral emissivity depends on wavelength then the total emissivity depends on the temperature.”
************
********
Electrons in the emitting body, require EM quanta of a discrete frequency that matches their orbital angular frequency.
Since matter emits EM energy as the EM quanta of a discrete frequency that matches their orbital angular frequency, and since matter consists from a number of elements… how scientists expect a planet surface to emit IR EM energy of a continuous spectra?
I don’t think the surface can emit a continuous IR spectrum. Rather, it would be a collection of individual frequencies from different elements in the surface at different temperatures.
I think there is something very wrong with the entire blackbody emission theory.
************
********
The Stefan-Boltzmann emission law is an abstraction. It doesnt define the mechanism the matter emits EM energy, because the blackbody is described as surface at some uniform temperature.
It was not said blackbodies consist from some kind of matter.
Blackbodies do not have any chemical composition, because blackbody is an abstraction.
***********
*******
“the GHE is based on the physics of the relatively great transparency of the atmosphere for shortwave radiation in comparison to a smaller transparency for longwave emission. Such that, the mean radiation balance at the earths surface is a positive value.”
–
How it happens? There is also the night. There is not any shortwave radiation at night.
–
Maybe it is meant that during day hours surface inevitably accumulates more energy, than solar flux provides?
Because less energy is emitted out of Earth’s system, than enters Earth’s system?
–
But doesn’t always a quasi equilibrium being achieved. The rise of Earth’s system energy emission, vs the rise of temperature?
–
In other words, the warmer the planet, the more energy the planet emits?
–
Doesn’t that eventually keep surface temperature at equilibrium levels?
-
Yes, and it really depends on the role of nonlinear feedbacks, as well as the direct forcings, as to where that equilibrium will be, and how long before it is reached.
When less energy is emitted to space, than it enters the Earth’s system, then the planet gets warmer.
It is the only way a planet gets warmer.
–
And when more energy is emitted to space, than it enters the Earth’s system, then the planet gets cooler.
-
It is all about electromagnetic energy balance; however, how that energy is captured and released depends on the various negative feedbacks to the orbital forcing’s changes.
-
******************
*************************
Mars is a unique case, which can help to clear everything up
Because, by a pure natural coincidence, the planet Mars' satellite measured mean surface temperature
Tsat.mars = 210K is the same as the planet Mars' the theoretical calculated effective temperature (not corrected)
Te.mars = 210K
******************
Ok
By moving planet Mars from its orbit at 1,52 AU distance from the sun, by moving Mars to Moon's and Earth's orbit distance from the sun at 1 AU,
by moving Mars, to Earth's-Moon's orbit, by doing so, the above condition for Mars
Te.mars = Tsat.mars
is always right
**************
Now
Te.earth = 254K
If Moon had Earth's Albedo, Te.moon would be
Te.moon =254K
If Mars had Earth's Albedo and Moon's (and Earth's 1AU) distance from the sun, the Mars' "would be" average surface temperature
Te.mars1AU = Tsat.mars1AU =254K.
Mars' cp = o,18 cal /gr*oC
Earth's cp = 1 cal /gr*oC
N.earth = 1 rot /day
N.mars = 0,9028 rot /day
******************
Mars and Earth are both smooth surface planets (Φ =0,47)
and...the mean surface temperatures RELATE (everything else equals) as their (N*cp) products' SIXTEENTH ROOT.
Ok
Let's apply the Planet Surface Rotational Warming Phenomenon, to calculate (via Mars) the Earth's without-atmosphere the average surface (Tmean) temperature
Tmean.earth.
Tmean.earth /Tmean.mars1AU =
= [ (N.earth*cp.earth) /(N.mars*cp.mars) ]1/16
Tmean.earth /254K = [ (1*1) /(0,9028*0,18) ]1/16 =
= (1 /0,1625)1/16 =
= (6,15369)1/16 = 1,120266
Tmean.earth = 254K * 1,120266 = 284,57K
or
Tmean.earth = ~ 284,57K
**************************
planet Earth's measured Tsat.earth =288K
the two numbers differ only by 1,2%.
Thus we have derived Earth's average surface temperature (by comparison) from an airless planet Mars.
**********
************
****************
So, there is not any +33°C
Atmospheric Greenhouse Effect on Earth’s surface.
The +33°C figure comes from a very much mistaken mathematical abstraction.
The currently observable global warming is a natural process caused by the Earth’s position while orbiting sun, it is the so called the ORBITAL FORCING.
There is nothing we can do to reverse the ORBITAL FORCING.
It is a natural process.
We shoud adapt…
–
************
****************
The next very important topic we discuss in the current research, which is explained and analized in every detail in the appended pages is:
The actual reason of the observed Global Warming.
It is dedicated to Milankovitch cycle and it explains where Milankovitch was absolutely right, and what Milankovitch overlooked.
*******************
Obliquity
It is the cycle Milankovitch attributed to as the main cause the Earth's climate getting in and out of Glacial Age.
The angle Earth's axis of rotation is tilted as it travels around the Sun is known as obliquity. Obliquity is why Earth has seasons.
Over the last million years, it has varied between 22.1 and 24.5 degrees with respect to Earths orbital plane.
The greater Earth's axial tilt angle, the more extreme our seasons are, as each hemisphere receives more solar radiation during its summer, when the hemisphere is tilted toward the Sun, and less during winter, when it is tilted away.
Larger tilt angles favor periods of deglaciation (the melting and retreat of glaciers and ice sheets). These effects aren't uniform globally — higher latitudes receive a larger change in total solar radiation than areas closer to the equator.
Earths axis is currently tilted 23.4 degrees, or about half way between its extremes, and this angle is very slowly decreasing in a cycle that spans about 41,000 years.
It was last at its maximum tilt about 10,000 years ago and will reach its minimum tilt about 10,000 years from now.
As obliquity decreases, it gradually helps make our seasons milder, resulting in increasingly warmer winters, and cooler summers that gradually, over time, allow snow and ice at high latitudes to build up into large ice sheets.
As ice cover increases, it reflects more of the Sun's energy back into space, promoting even further cooling.
When the tilt angle is low, summers at high latitudes will be cool, and the tropics will be closer to the equator.
Therefore, the ice will not melt in the summer because the warm oceans will provide plenty of snow in the winter.
–
Also, winters at high latitudes will be warmer, and, again, the tropics will be closer to the equator.
So, the picture has cleared, the more tilted the axis is, the less annually differentiated the surface temperatures are.
A sphere emitting the same amount of IR energy, the less differentiated the surface temperatures are, the higher the average surface temperature.
Therefore, according to Milankovitch, the tilt gradually lessens, and, as a result, the annual surface temperatures are getting more differentiated, therefore the planet average surface temperature should be in a lessening pattern.
–
Earth is going through a very slow and naturally caused warming trend!
Earth’s energy never balances, as both incoming and outgoing energies constantly vary.
Earth Energy balance, use units of energy.
To actually find Earth’s energy Imbalance, energy-in must be compared to energy-out.
-
The temperature is going up – it’s called orbitally forced climate change phenomenon.
-
Planet Earth is very slowly getting warmer. It is happening due to orbital forcing.
It has been calculated by Milankovitch.
-
The Milankovitch's Graph, which is calculated on the basis of all three major orbital cycles (rotational axis Obliquity, the Earth's elliptical orbit Eccentricity, and Precession of equinoxes) shows for current time Earth being in a slow cooling trend.
-
Milankovitch simply assumed the glacial periods should be associated with the higher latitudes cooler summers.
-
-
Milankovitch was considering solar EM energy simply being either reflected, or absorbed.
But what the solar EM energy actually does, is to INTERACT with planet surface matter.
When INTERACTING with matter, EM energy
1). Partly gets reflected
2). Another portion gets instantly transformed into IR EM emitted energy
3). And only the rest (only the what is left after INTERACTION) gets absorbed in inner layers.
The Earth's surface has a different distribution of land and oceanic waters areas between the Northern and the Southern Hemispheres.
There is much more land on the Northern Hemisphere, and there is much more oceanic waters on the Southern Hemisphere.
-
So, there, inevitably, the results of EM energy INTERACTION with surface should be (between the Northern and the Southern Hemispheres), it should be dramatically DIFFERENTIATED.
-
Thus, along with all three Milankovitch cycles, we have the solar EM energy INTERACTION with surface the differentiated results.
In that consideration, the orbital Eccentricity combined with the Earth's rotational axis Precession play the major deterministic role.
In our times Earth's Perihelion almost coincides with the Southern Hemispher's summer solstice.
What actually happens, as the combination-result of all three Milankovitch cycles, plus the solar EM energy INTERACTION with earthen differentiated surface ( a different distribution of land and oceanic waters areas between Northern and Southern Hemispheres),
the combination-result is that when the Northern Hemisphere is in cool summers, the Souther Hemisphere is in very Hot Summers.
-
Earth's surface thermal energy reservoir are Earth's oceans. There are much more oceanic waters in Southern Hemisphere, compared to the North Hemisphere.
-
In our time Earth accumulates much heat in the Southern Oceans during those very hot summers. That is why Earth's Global temperature rises!
-
The Milankovitch Graph is being very precisely calculated. Only it has to be read reversed.
When we look at the Milankovitch Graph reversed, it becomes very much obvious, Earth is going through a very slow and naturally caused warming trend!
-
-
What Earth's surface does, is to still emerging from an ice age!
The Original Milankovitch Graph states:
"You get an interglacial when the Summer is warm enough to melt the snow that fell during the Winter.
You get a glacial period when there is not enough summer heat to melt the snow. Then each year the thickness of the snow increases until you have new ice sheets."
-
-
The Reversed Milankovitch Graph states:
You get an interglacial when Winter on North Hemisphere occurs close to Earth's Perihelion. The Southern Hemisphere's vast oceanic waters are tilted towards the sun, when Earth is at its closest to the sun.
Thus, as it occurs in our era, during the North Hemisphere's warmer Winter, the very much hotter Southern Hemisphere's SUMMER oceanic waters are heavily accumulating, and that is why we observe the current Global Warming.
-
Earth in our era is in a very long term continuous warming period. This warming is caused by natural orbital forcing.
–
It is the continuation of the MWP (Medieval Warm Period). The LIA (Little Ice Age) was a phenomenical cold because of the intensive mitigation of sea ice. During the LIA period, Earth continued accumulating solar energy, Earth continued getting warmer.
–
******
Milankovitch Cycles and Glaciation (iu.edu)
https://geol105.sitehost.iu.edu/images/gaia_chapter_4/milankovitch.htm
*****
And from the current temperature data there is no question that we are firmly in a steady secular warming period.
*****
Every planet is subjected to its annual average surface temperature (the mean surface temperature) T (K).
The planet annual average surface temperature is a dependent on the planet's distance from sun value.
Of course it is dependent on the planet's radiative energy balance.
It is also dependent on the planet's rotational warming phenomenon.
And, in addition to all that above, the planet annual average surface temperature is a dependent on the annual planet surface temperature differentiation.
The less planet surface temperatures annually differentiated - the higher is the planet annual average surface temperature.
And the more planet surface temperatures annually differentiated - the lower is the planet annual average surface temperature.
-
In our times Planet Earth is in an exceptional annual orbital pattern, which pattern (earth's orbit eccentricity, when Earth is at its closest to the sun during the North Hemisphere's winter, and it is very much close to the sun at the times of winter Solstices...)
-
Thus we witness the seasonal warming and cooling periods.
And they are orbitally forced yearly cyclical phenomena.
What we witness in Northern Hemisphere is the summers being cooler and winters being warmer.
The opposite phenomenon, (the summers being hotter and the winters being colder) which actually takes place in Southern Hemisphere, is being smoothed by the Southern Hemisphere'svast oceanic waters areas.
As a result, at current times Earth's annual orbital pattern creates a slow lowering the Planet Earth's the annual average surface temperature differentiation.
This exact phenomenon is what creates the observed in our era the very slow (millennia’s long) continuous (gradual)Global Warming.
********
My greatest philosophy has been that to know any subject accurately, a scientist must look at the cause and effects in the simplest terms. That way, errors in assessments will not skew the predictions unnecessarily.
********
I was referring to the Precession.
“The third and final of the Milankovitch Cycles is Earth’s precession. Precession is the Earth’s slow wobble as it spins on axis. This wobbling of the Earth on its axis can be likened to a top running down, and beginning to wobble back and forth on its axis. The precession of Earth wobbles from pointing at Polaris (North Star) to pointing at the star Vega. When this shift to the axis pointing at Vega occurs, Vega would then be considered the North Star. This top-like wobble, or precession, has a periodicity of 23,000 years.”
https://geol105.sitehost.iu.edu/images/gaia_chapter_4/milankovitch.htm
“Due to this wobble a climatically significant alteration must take place. When the axis is tilted towards Vega the positions of the Northern Hemisphere winter and summer solstices will coincide with the aphelion and perihelion, respectively. This means that the Northern Hemisphere will experience winter when the Earth is furthest from the Sun and summer when the Earth is closest to the Sun. This coincidence will result in greater seasonal contrasts. At present, the Earth is at perihelion very close to the winter solstice.”
********
Our planet Earth is in millennials long continuous orbital forced warming pattern.
-
When in warming pattern, a planet accumulates more solar energy than planet is capable to emit.
In planet's effort to emit that excessive solar energy so to establishing the radiative energy equilibrium, the planet average surface temperature rises.
-
When the planetary temperature becomes higher, then, according to the Stefan-Boltzmann emission law, the planet surface becomes capable of emitting IR (infrared radiation) more intensively.
Thus the mechanism of getting rid of energy does establish onto the planet surface, a close to radiative equilibrium (energy in =energyout) state.
-
The planet surface temperatures from Equator to Poles are very much differenciated.
Here it is when the nonlinearity of the Stefan-Boltzmann's emission law gets in action!
-
The Polar zone's temperature rises faster, than the equatorial, or the average planet surface mean.
It is the phenomenon of Polar Temperatures Amplification.
-
Due to the Stefan-Boltzmann emission law nonlinearity, the Polar areas (in order to get rid of the excessive incoming solar energy)...
The Polar areas surface temperatures rise faster - and it is observed in the melting of the ice sheet sea cover.
********
The orbital cycles forcings are slow. Only our Earth is now in a culmination phase of the current orbital warming curve.
–
Milankovitch Graph, read as it should be read – when it is read correctly, when it is read the inverse way, because currently when our planet is nearPerihelion (the closest to the sun point) at January 4,
At that exactly time Earth’s Southern Hemisphere is in Solstice, and the Earth’s Southern End of axis is tilted towards sun.
–
In our era we are witnessing an exceptional coincidence phenomenon – Earth is accumulating solar energy at its fastest way in millennials.
*********
March is usually the hottest time of the year for the oceans because it is late summer in the Southern Hemisphere, where most of the world's major seas are located.
Again,
According to Milankovitch, Glacial Cycles are driven by the amount of heat reaching high Northern latitudes, which varies with orbital eccentricity and the relative timing of apoastron and the precession of the equinoxes.
-
During an interglacial enough heat arrives to melt Winter snow above 50N latitude during each Summer.
The other 90% of the time snow persists until the end of each Summer and the next Winter’s snow falls on top of last year’s.
-
Over years the snow builds up into extensive ice sheets which can extend as far as New York or London.
-
Incidentally a change in global average of 4C is sufficient to make the difference between ice sheets in Greenland and ice sheets in England.
-
--
Milankovitch made a mistake, because in his time he witnessed a gradual cooling.
Ice has specific heat 0,5 cal/(gr*oC)
Water has 1 cal/(gr*oC)
Thus, at the period of sea ice intense melting, the average air temperature dropped, the land glaciers started grow – and planet experienced it as the LIA (Little Ice Age).
That period is over now.
*********
There is not any emergencies to rush. The fossil fuels burning (the intensive CO2 emissions) do not whatsoever affect Global climate temperature.
-
Global warming happens because of the more uniform global temperature. It is getting warmer for some millennials now.
At present, the Earth is at perihelion very close to the winter solstice.
*************************
So, we have the annual swings in Earth’s Global temperature.
Annually the Earth’s energy solar input varies
about 90 W/m² during a calendar year.
Earth’s average surface temperature
is 2.24 degrees C higher when the Earth is much farther from Sun (Aphelion).
The global temperature swings turn out to depend more strongly on the warming & cooling of the land, than on the warming & cooling due to distance.
So, we are witnessing a 2.24 degrees C annual swings in global temperature.
-
-
A natural thought begs the question:
What were the global temperature swings ~ 11.000 years ago at Holocene's Optimum ?
Were they higher than 2.24 degrees C ?
Of course they were higher, and they were much-much higher, because continents heat up and cools down more than oceans.
The vast majority of land is in the northern hemisphere. The global temperature swings turn out to depend more strongly on the warming & cooling of the land, than on the warming & cooling
due to distance.
But about 11.000 years ago the distance played a very significant role, because Earth’s energy solar input also varied about 90 W/m² during
a calendar year.
About 11.000 years ago in January, Earth was much farther from Sun (Aphelion). In July, Earth was much closer to Sun (Perihelion). And the difference in solar flux between those two extremes was also
about 90 W/m².
So we have the proofpoint here:
When the global annual temperature swings are higher, then the global average temperature inevitably is lower.
So, at times of Holocene Optimum, instead of the allegedly said sweet warm global climate, there was a much-much colder global climate, than what we have in our era.
Also, since then, Earth is experiencing a slow, 11.000 years long continuous orbitally forced
WARMING PATTERN.
-
-
*******
A higher CO2 content in ice cores' samples
A higher CO2 content in ice cores' samples may as well testify for a much colder temperatures at the times the ice was formed.
At very low temperatures the CO2 got frozen out of the air, had fallen on the glacier and had sequestered in the ice - thus a higher CO2 content in ice core samples.
https://en.wikipedia.org/wiki/Carbon_dioxide
Properties:
Density
1562 kg/m3 (solid at 1 atm (100 kPa) and −78.5 °C (−109.3 °F))
1101 kg/m3 (liquid at saturation −37 °C (−35 °F))
1.977 kg/m3 (gas at 1 atm (100 kPa) and 0 °C (32 °F))
-
-
*******
There is the Higher Latitudes Temperatures Amplification Phenomenon.
Because the warming is an orbitally forced process.
But there is also the transportation of the accumulated at the equatorial areas heat by winds and oceanic currets towards the globe’s higher latitudes.
–
CO2 is a very insignificant for the global temperatures, because the CO2 is a trace gas and its participation is infinitesimal.
********
8000 year old boatyard which is 11 metres underwater
There is archaeological evidence of an 8000 year old Mesolithic boatyard off the East coast of England.
https://www.ancient-origins.net/news-history-archaeology/oldest-shipyard-0012465
It’s 11 metres underwater, on a coast which has been sinking at 1.5mm/year since the ice melted. Sounds about right for a boatyard which was at sea level 8000 years ago.
And it is a strong evidence that Planet Earth is in a continous millenials long warming pattern.
********
And we are still in an Ice Age…
How much of the Antarctic Ice Sheet is below sea level?
"In terms of area:
5.50 x 10^6 km^2 (or 5,500,000 km^2) of ice is grounded below sea level.
The total area of the ice sheet is 12.295 x 10^6 km^2 (or 12,295,000 km^2).
Therefore, ~45% of the ice sheet, in terms of area, is grounded below sea level.
However, a great volume of ice remains above sea level. This ice, above flotation point, contains enough ice to raise global sea levels by 57.9 m."
–
https://www.antarcticglaciers.org/question/much-antarctic-ice-sheet-sea-level/
********
For more Pages please Click on the Box at the Top
Opponent:
“Christos,
And you don’t include the effects of atmosphere and its composition (rather, dismissing it as “thin” when it is not), which has been the whole point of this never-ending discussion. I hope the discussion will help you reformulate your analysis and to justify the unjustified dismissals, but I fear not – it is rather circular to dismiss the effect that you are trying to prove doesn’t exist.”
–
Thank you for your comment.
“you don’t include the effects of atmosphere and its composition (rather, dismissing it as “thin” when it is not)”
Well, I do not dismiss the Earth’s Global Greenhouse Effect.
What I say is I don’t see it happening.
–
When eight years ago I started having my very serious reservations about the role of CO2 trace greeehouse gas content of ~400 ppm in Earth’s atmosphere I learned about an equation (the planet effective temperature equation) which theoretically estimated planet average surface temperature for every planet and moon without-atmosphere in solar system.
When the planet without-atmosphere New Tmean equation was formulated, I tried it on every planet and moon without-atmosphere in solar system, and, when realising the equation was very much precise, then I also checked the New Tmean equation in the case of Earth… to estimate what the on the Earth’s surface the whole atmosphere greenhouse effect would be.
And, the New Tmean equation for planets and moons without-atmosphere, which equation didn’t include the effects of atmosphere and its composition (why it had to, it was for planets and moons without-atmosphere, the exact way the effective temperature equation is), that New Tmean equation is able to theoretically calculate the Earth’s average surface temperature very precisely close to the measured by satellites.
It is then I added to the planets and moons without-atmosphere expression, I added the sentence : “(or with a thin atmosphere, Earth included)”.
Because I understood, that for EM (electro-magnetic) radiative energy an Earth’s kind of atmosphere should be considered thin, or transparent, to the solar energy interaction processes with planetary surface, as it is happen to be transparent for planets and moons without or with a thin planetary atmospheres.
If the New Tmean equation had theoretically calculated for Earth, par example, Tmean =275K, then the Earth’s entire atmosphere greenhouse effect would have been as:
288K – 275K =13C
But,
And the result was, that the equation calculated Earth’s without-atmosphere average surface temperature being as Tmean =287,74K
wich result is very much close to the measured by satellites the T = 288K.
The New Tmean equation does not include the effects of atmosphere and its composition…
And it is concluded then, that the equation does not have to include the effects of atmosphere and its composition, because their effect is very insignificant when the Earth’s mean surface temperature is being by the New Tmean equation theoretically calculated.
–
So, we should conclude, and we conclude with great confidence, there is no room left for the trace greenhouse gas CO2 to influence in any possible way the Earth’s average surface temperature.
*******************
Opponent:
“How do you (1) calculate the solar flux absorbed if it is not S(1-α)πr² ?”
–
I do not calculate the absorbed part, what I do is to correctly calculate the not reflected portion of the incident on planet the TOTAL solar energy:
πr²*Φ*(1 -a)*S by the use of the Φ -the solar irradiation accepting factor (the planet spherical shape and roughness coefficient).
Φ =0,47 for smooth surface planets and moons.
Φ =1 for heavy cratered planets and moons, and, also for gases planets and moons.
the not reflected portion of the incident on planet the TOTAL solar energy:
πr²*Φ*(1 -a)*S is the TOTAL solar energy which actually interacts with the planetary surface.
The same πr²*Φ*(1 -a)*S is the TOTAL IR emitted, because of energy in = energy out to met
–
Important NOTICE:
The same πr²*Φ*(1 -a)*S is the TOTAL IR emitted,but the amount of absorbed is always less.
And, the faster a planet rotates, the higher is the amount of solar energy absorbed.
**********************
Theoretically, a planet with zero Albedo (a = 0).
The diffuselly reflected a*S = 0*S =0 W/m²
1). When it is a planet (a = 0) with rough surface
Φ = 1,
Φ(1 -a)*S = 1*(1 -0)*S = S W/m²
the not reflected equals to the incident.
2). When it is a planet (a = 0) with smooth surface
Φ = 0,47,
Φ(1 -a)*S = 0,47(1 -0)*S = 0,47*S W/m²
the not reflected is 0,47*SW/m²
and the specularly reflected is
S -0,47*S =0,53*S W/m².
–
In solar system planets and moons are either with rough surface
Φ =1, or with a smooth surface Φ =0,47
Only Triton’s surface is somewhere in between
(0,47 < Φ < 1)
**********************
Opponent:
“(2) How do you account for heat conduction which causes heating of the subsurface on the light side, and replenishment of emissive losses on the dark side?”
-
The part of energy, which is absorbed as heat “travels” and gets emitted from the dark side along with the rotating surface.
–
Opponent:
“(3) Can you derive/describe β from first principles if it is to be a universal constant?”
-
I cannot. And we have unknown principles – the not reflected portion of the incident on planet the TOTAL solar energy:
πr²*Φ*(1 -a)*S does not get absorbed in surface, only a part of it gets absorbed.
Maybe we do not know everything that is going on in nature yet.
Aren’t there other universal constants which are not derived/described from first principles?
-
*****************
Now, let's estimate it the other way...
Since Earth's total atmospheric greenhouse effect is ~ 0,4°C, how much could the 0,04% CO2 content's in atmosphere participate in that ~ 0,4°C greenhouse forcing?
-
Light impacting a surface does those three things.
It is reflected, it is transformed into IR outgoing EM energy, and it is absorbed.
The portion of light which is not reflected, it is interacting with matter.
Absorption is included in interaction.
When solar light gets reflected, it is also a result of interaction, but let’s consider it separately, because the reflected light doesn’t count in the planet radiative energy balance.
When the not reflected light interacts with matter
it gets partly transformed into IR outgoing EM energy
and partly transformed into heat, which heat is absorbed.
-
Opponent:
“Light that is not reflected from the Earth is absorbed. That means its energy is deposited in the material.
Only if the surface warms as a result does it get emitted as IR.”
–
Answer:
Do you mean that when energy is deposited in surface, the surface gets warmed first, then surface, as a result, emits that energy as IR ?
How much should the surface get warmed before it starts emitting ?
Doesn’t surface emit all the time ?
-
If a planet (EARTH) was a perfect uniform surface content spherical object. But it is not.
–
The Northern Hemisphere is crouded with land, and the Southern Hemisphere is mostly ocean.
So the two hemispheres have a very different respond when interacting with incoming the Solar EM energy.
–
There is Earth’s axial tilt. Earth’s axis is pointed to the star Polaris.
Thus Earth has big differences on its surface interaction process with solar energy during Earth’s yearly orbit around the sun.
–
It is not an exageration to say, that the Earth’s Hemispherical yearly seasons are the orbitally forced on the shortest time-scale (one year) the two orbitally forced “climate changes” – the warming
period (spring-summer) and the cooling period (autumn-winter). Both they are consisting a yearly cycle, which results to the yearly average surface temperature.
-
***********
******
When a Hemisphere is in its mid-summer, there is a big difference between the Northern Hemisphere mid-summer solar energy absorption, compared to the Southern Hemisphere mid-summer solar energy absorption.
Because Southern Hemisphere exposses to sun much more sea-water areas, than the Northern Hemisphere at its summer-time does.
The difference between solar irradiated land and the solar irradiated water - the water gets less warm, and the water accumulates more heat, because water vs land has a five times higher the specific heat cp (cp.land ~ 0,19 (cal/gr*oC)
vs cp.water ~ 1 (cal/gr*oC)).
-
Not to forget the most important thing - planet Earth orbits sun on an elliptical (eccentrical) orbit.
Currently Earth's Perihelion occurs very close to the December Solstices ~ 4th of January. At the time there is midsummer at Southern Hemispher's oceanic waters.
Perihelion - Aphelion = 7% more solar irradiation intensity at closer to sun Perihelion, than at Aphelion.
But at Perihelion a stronger sun falls on oceanic waters and gets accumulated year after year, and causes the currently observed global warming.
-
it is not a yearly cycle accumulation process, but a multimillennial one.
12 thousands years ago, the Perihelion was occured at Northern Hemisphere's summer time. The 7% stronger sun fell on the land and was accumulated much less.
-
And that is why we experience a global warming trend now!
-
https://www.timeanddate.com/astronomy/perihelion-aphelion-solstice.html
A Fragment of the Original MIlankovitch Grapheme
"So why has that warming accumulation only been happening for the last 150 years, not the last 8,000?"
-
-
It is not happening only for the last 150 years, but for the last 8,000. It was a Glacial (remember?), and now we are in an Interglacial.
-
What you asking is the why it is getting hotter so fast now.
Well, it is an impression we have as living creatures. We have our biology producing heat. When it is warm enough it becomes difficult for us to cool our bodies, so we start sweating.
This time of the year (when we sweat) is in mid July and August.
We do not sweat in March, not in April, not in May, not even in June - all this months there is a warming accumulation happening on the Northern Hemisphere!
-
Well, NATURE has its own mechanism - it is a gradual reducing the amount of ice and getting the oceanic depths a little warmer...
-
When there is not much ice left, the temperatures rise faster.
-
And, yes, Precession of the equinoxes (22 ka) only has relatively smaller effects, particularly now, while eccentricity is low…
A couple of thousands years and we shall be in the cooling pattern again.
A Fragment of the Reversed MIlankovitch Grapheme
“Without the greenhouse gas effect, surface temperatures would drop 33 degrees Celsius (59.4 degrees Fahrenheit), according to the World Meteorological Organization (WMO) — making the planet a frozen, uninhabitable place.
For thousands of years, nature had well-regulated the concentration of these gases. But this started changing when humans began burning fossil fuels as a global means of creating energy — resulting in a sharp rise of unnatural CO2 emissions. This has interfered with the planet’s atmospheric balance.
And, as a result, Earth started warming faster.”
–
“For thousands of years, nature had well-regulated the concentration of these gases.”
***********
************
It is impossible to reconstruct the global temperatures for tens thousands years back.
"Look at the graph. Starting 22,000 years ago temperatures began to rise.
http://railsback.org/FQS/FQS22katoFutureTemps03.jpg
They stabilised around 10,000 years ago and remained around 14.3C until 5000 years ago.
That is the sweet spot, the Holocene Optimium.
Temperatures then began to cool. Over the next 5000 years to 1880 the temperature dropped naturally from 14.3C to 13.8C.
Since 1880 artificial warming has raised the temperature by 1.1C to 14.9C.
All these values have confidence limits of +/- 0.1C and are real values."
http://railsback.org/FQS/FQS22katoFutureTemps03.jpg
What I can do is to reconstruct a trend (either it is a warming trend, or it is a cooling trend) for some period of time.
It is the warming trend for the last ~ 11000 years.
First it was a slower warming, then as it proceeded, the rate of warming accelerated.
What we witness now are the culmination times of the warming trend.
In about a millenial and half it will start gradually cooling.
–
Now, how it is possible to reconstruct the global temperatures for tens thousands years back –
I think it is impossible.
-
***************
***********
**********
Thank you GOOD NATURE!!!
What Nature does is a one way decaying process. It is a never stopping cooling process.
-
Earth is slowly cooling since its formation.
Once in the process carbon (C) occured, when combined with hydrogen (H2) the life occured.
Carbon in form of CO2 gets captured and sequestered. It gets sequestered in the natural sinks (oceanic waters, rocky sediments, and coal, natural gas and oil deposits).
By doing so, Nature exploites life. Also, Nature doesn't care about life's future existence.
Many large forms of life (dinosaurs etc...) went extinct, because the Natural CO2 depletion from Earth's atmosphere made the food for the large species very scarce, so the smaller species were more adapted. It was then large mammals (whales) turned back into sea, because there still was enough food.
If Nature is left on its own, Nature will lead life on Earth to inevitable natural ecological catastrophe.
-
It is a naturally accelerating warming phenomenon!
Well, a planet has a spherical shape, right?
Earth is a planet, so Earth has a spherical shape.
The more solar energy Earth accumulates, the warmer the higher latitudes become.
Also, the higher is the latitude on sphere, the smaller is the area.
Which means that for the same additional portion of absorbed solar energy the Global Warming will be a more and more accelerated orbitally forced phenomenon…
Some centuries ago the Gulf Stream was bringing its warm waters up to the 65 degrees.
As the Polish Meteorologist Ireneusz Palmowskiexplains:
“warming will continue in the northern hemisphere for several thousand more years until perihelion shifts to May.
Then the extent and thickness of ice in the north will increase because less solar energy will reach the northern hemisphere in winter.”
Link:
https://judithcurry.com/2024/07/10/implications-of-the-linear-carbon-sink-model/#comment-1008324
Now the Gulf Stream is flooding with its warm waters the Arctic area up to 80 degrees.
It will take some millenials this orbitally forced natural phenomenon to be reversed.
Opponent:
“The Gulf Stream is weakening.”
Answer:
Of course the Gulf Stream is weakening. Because it is reaching the end of its jorney!
The Gulf Stream what it did was to get more and more northern.
It is almost nowhere to move up the Globe any more. So the Stream is flooding the Arctic areas with its warm waters.
It is a naturally accelerating warming phenomenon!
*************
Notice:
The Storage Batteries are only efficient the faster their cycles of charging/discharging goes on.
If battery factories get seldom discharged to the 1/2 of their capacity, the project is never going to pay off the costs.
Also, the pumped storage, compressed air, liquid air and stored thermal solar generation are all storage mechanisms from which electricity can rapidly be retrieved on demand.
-
Of course they are energy storage mechanisms. Energy storage always reduces the renewables efficiency.
It is meant that we shall storage when we have excessive renewable electricity production.
There will be times the storage capacities were full, and still having plenty of exceesive renewable electricity.
How much storaged energy should we have untouched, for the emergency cases?
Now, imagine, there is not for long periods renewable energy and the storage capacities already empty.
Should we have supplementary renewable energy production to always support the storaging efforts?
The Storage Batteries are only efficient the faster their cycles of charging/discharging goes on.
The same goes with every other storage mechanism. The storage mechanisms are good when they have faster cycles of charging/discharging.
It is much better to backup the solar farms with fossil fuels burning electricity production. It is very much cheaper and it is the only perfectly balanced scheme.
Again, the fossil fuels burning does not pose any danger to Global climate.
The Global warming observed happens due to orbital forcing- it is a millenials long slow warming trend.
The more planet Earth gets along that trend the faster the global average temperature rises. At the top of the Globe, at the Northern areas every year there is less sea ice cover.
Since the higher the latitude, the smaller the Globe's respective area, thus the advance of sea-ice melting is every year proportionally higher.
Fifty years ago there always were variations on the electric energy demand. When there were lower demand electric plants went on idle, or even stopped for a while.
When there was higher demand the plants worked full capacity.
The emergencies higher demand was covered by gas turbines and by hydros.
On current trends, the all-in cost of the electricity they produce promises to be less than half as expensive as the cheapest available today.
How can the cost going up, up, up have a trend to go down?
We need a cheaper, not a more expensive electric energy.
There is no need to have built a tremendous giantic infrastracture in order to store more and more electric energy, because the main argument – the Anthropogenic Global Warming (AGW) is a huge scientific mislead.
There is not any problem for Earth’s climate when we proceed using fossil fuels as we always used to.
****************
References - Links
1).
Earth Temperature without GHGs - Energy Education
2).
3).
Using Earth’s Moon as a Testbed for Quantifying the Effect of the Terrestrial Atmosphere (scirp.org)
4).
************************
For more Pages please Click on the Box at the Top
*************
Comments
Thank you, Dug, for your respond. I am concerned the same as you for the reasons of climate warming. It is not happening due to the tiny CO2 content in Earth's atmosphere, isn't it?
I recently thought about checking this website again and just wanted to ask, have you made any extra progress with your theory lately? How have debates been going? The science seems sorta solid.
08.08.2022 13:43
Christos Vournas
07.08.2022 23:16
High KL
Thank you for your interest in my work
Have you seen the last page yet?
T= ( J /σ )¼ mistake
23.05.2022 13:41
Shadeburst
How many molecules of CO2 in a single cubic centimeter of atmospheric air at stp? Roughly one followed by sixteen zeros. As you are mathematically literate you can easily verify this.
Christos Vournas
23.05.2022 15:56
Hi, Shadeburst.
Yes, I know.
KL
12.01.2022 06:02
I wish I could accept your science but it’s not that convincing I guess.
Christos Vournas
12.01.2022 09:40
Hi KL. I am glad you wish to accept my work. Maybe you have not seen all my work. There are over 100 separate pages in my website. My arguments are very strong and very much convincing.
12.01.2022 06:01
KL
I’ve read your arguments with other people on Roy’s website but I’m more inclined to believe the people who refute your claims. A lot more evidence just seems to point to the fact we’re causing AGW.
Shadeburst
23.05.2022 13:37
That's the actual problem. Outside of urban legend there is no evidence.
11.01.2022 09:10
Christos Vournas
KL, I discuss my views in D.Ph. Roy Spencer's Blog since April 2021. Here yesterday
https://www.drroyspencer.com/2022/01/uah-global-temperature-update-for-december-2021-0-21-deg-c/#comment-1117652
KL
12.01.2022 05:59
Hi! Again, I’ve read your work and I’m interested but at the same time skeptical. I’m not really all too well versed with numbers and math so I can’t debate with you really. 😂
KL
11.01.2022 07:46
Late followup question, don’t you feel like you could possibly be wrong? A lot of evidence is pointing towards AGW being real. Not to discredit you but just curious!
Christos Vournas
11.01.2022 08:54
The method is "The Planets Surface Temperatures Comparison Method". I discovered the "Planets Surface Rotational Warming Phenomenon". Also the Φ -Planet Surface Solar Irradiation Accepting Factor.
KL
Well that leaves me with more questions, why have the years been getting warmer and warmer from what I’ve observed? This winter was cold but not as cold as usual in the past. Something is going on imo
11.01.2022 04:18
Christos Vournas
11.01.2022 08:33
KL
Thank you for asking. Planet is in slow millennia continuous warming trend. Please visit "Reversed Cycle" and "Milankovitch Reverse" pages in my site. The warming caused by orbital natural phenomenon
09.01.2022 07:15
So can you explain in laymans terms what all of your research means for global warming?
Christos Vournas
10.01.2022 09:13
The conclusion - there is not any measurable GWE on Earth's surface.
Christos Vournas
23.08.2021 19:19
Thank you for asking George. Venus is a unique case of rocky planet with dense atmosphere- and strong GHE. Please visit the page for Venus in my site:
https://www.cristos-vournas.com/446364348
Douglas Cotton (Retired Physicist)
I can't wait to see you explain with your fictitious science (that treats the globe as if it had multiple suns all around it)
how the Venus surface warms on the sunlit side. (LOL)
Christos Vournas
KL
25.10.2022 11:26
25.10.2022 13:54